丁衛(wèi)平

(湖南理工學院 數(shù)學學院, 湖南 岳陽 414006)

帶p-Laplacian算子時滯微分方程邊值問題多個正解的存在性

丁衛(wèi)平

(湖南理工學院 數(shù)學學院, 湖南 岳陽 414006)

應(yīng)用錐上不動點定理, 研究具有p-Laplacian算子邊值問題

邊值問題; 錐; 不動點定理; p-Laplacian算子; 正解

引言

邊值問題一直受到不少學者關(guān)注. 文[1]研究了Sturm-Liouville邊值問題

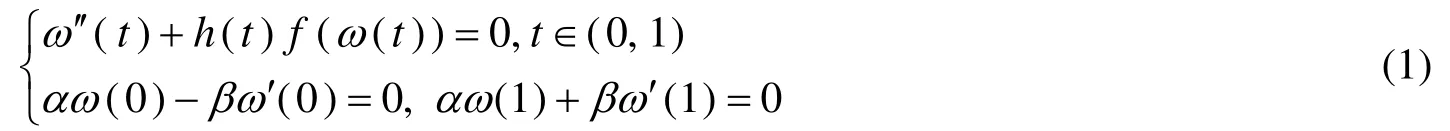
并給出了邊值問題(1)有n個對稱正解的存在性證明; 文[2]研究了如下

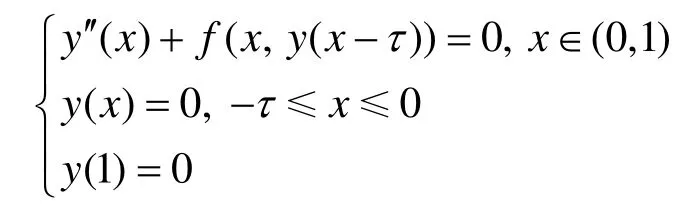
的邊值問題, 推廣了有關(guān)結(jié)果; 文[3]研究了邊值問題并采用了錐上的不動點定理給出了(2)存在三個正解的充分條件. 本文將采用不同于文[1]～[3]的方法研究時滯兩點邊值問題

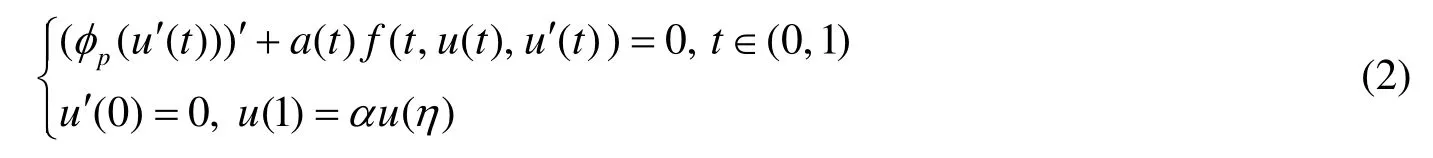
1 主要結(jié)果

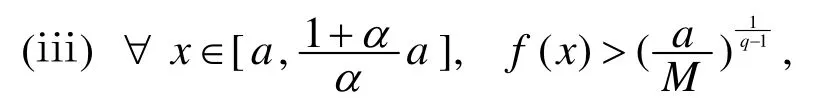
則邊值問題(3)至少有兩個正解.

2 主要結(jié)果的證明

2.1 定理1的證明

因此, 根據(jù)引理1, 邊值問題(3)至少有三個正解.

2.2 定理2的證明

[1] Yao Q.Existence and iteration of n symmetric positive solutions for a singular two-point boundary value problem[J]. Compute. Appl., 2004, 47: 1195～1200

[2] 蔣達清, 張麗莉. 二階時滯微分方程邊值問題的正解[J]. 數(shù)學學報, 2003, 46(4): 739～746

[3] 杜 波, 葛渭高. 一類具p-Laplacian算子邊值問題三個正解的存在性[J]. 數(shù)學的實踐與認識, 2008, 38(20): 201～204

[4] 郭大鈞. 非線性泛函分析[M]. 濟南: 山東科學技術(shù)出版社, 2001

Existence of Multiple Positive Solutions of Boundary Value Problems with p-Laplacian Delay Differential Equation

DING Wei-ping
(College of Mathematics, Hunan Institute of Science and Technology, Yueyang 414006, China)

By using a fixed point theory in cones, the paper studies the existence of positive solution for the two-point boundary-values problem

boundary value problem; cone; fixed point theorem; p-Laplacian operator; positive solution

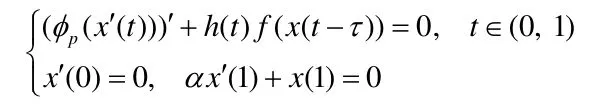
O175.8

A

1672-5298(2010)03-0017-04

2010-04-03

丁衛(wèi)平(1964- ), 男, 湖南岳陽人, 碩士, 湖南理工學院數(shù)學學院副教授. 主要研究方向: 信息論及應(yīng)用