及萬(wàn)會(huì), 黑寶驪

?

關(guān)于二項(xiàng)式系數(shù)級(jí)數(shù)恒等式

及萬(wàn)會(huì), 黑寶驪

(銀川能源學(xué)院 基礎(chǔ)部, 寧夏 銀川, 750105)

根據(jù)一個(gè)已知級(jí)數(shù), 使用裂項(xiàng)方法得到分母含有1到5個(gè)因子的二項(xiàng)式系數(shù)級(jí)數(shù). 所給出二項(xiàng)式系數(shù)級(jí)數(shù)的和式是封閉形的, 并給出二項(xiàng)式系數(shù)數(shù)值級(jí)數(shù)恒等式. 裂項(xiàng)的方法研究二項(xiàng)式系數(shù)變換是組合分析的新手段, 也是產(chǎn)生新級(jí)數(shù)的一個(gè)初等方法.

二項(xiàng)式系數(shù); 裂項(xiàng); 級(jí)數(shù); 封閉形; 恒等式

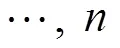
1 主要結(jié)論與證明

定理1 a. 分母含有1個(gè)因子的二項(xiàng)式系數(shù)級(jí)數(shù)恒等式.

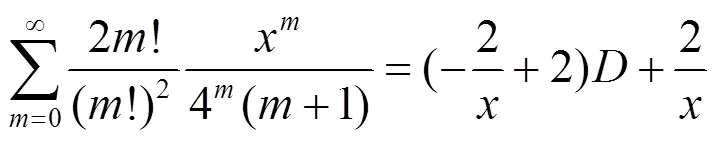
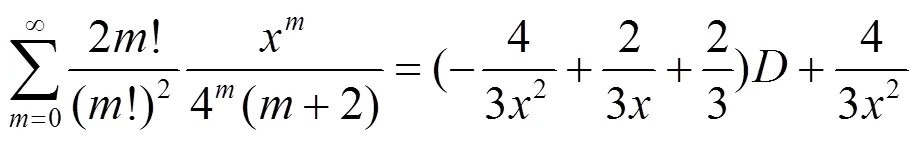
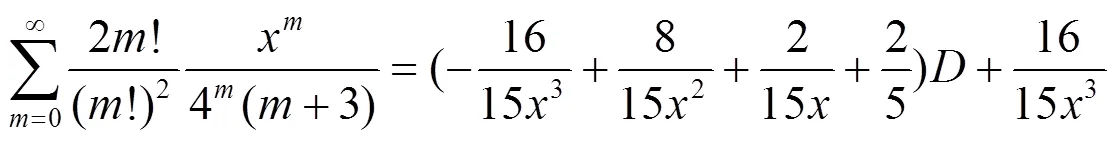
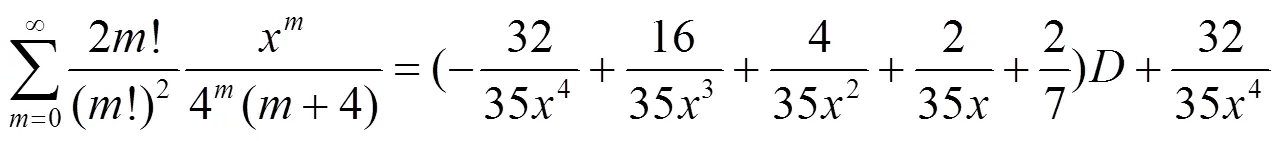
b. 分母含有2個(gè)因子乘積的二項(xiàng)式系數(shù)級(jí)數(shù)恒等式.

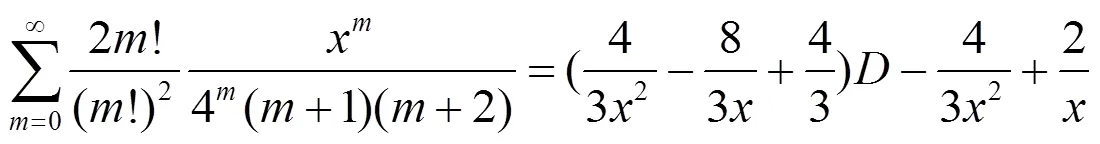
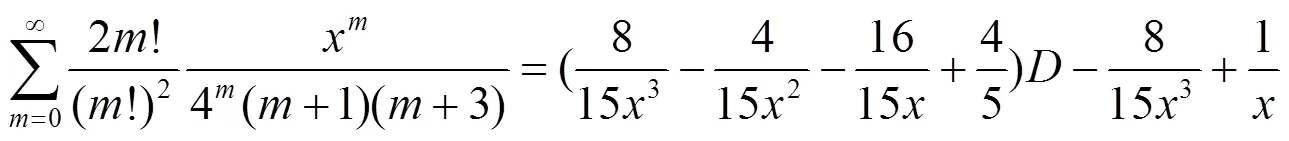
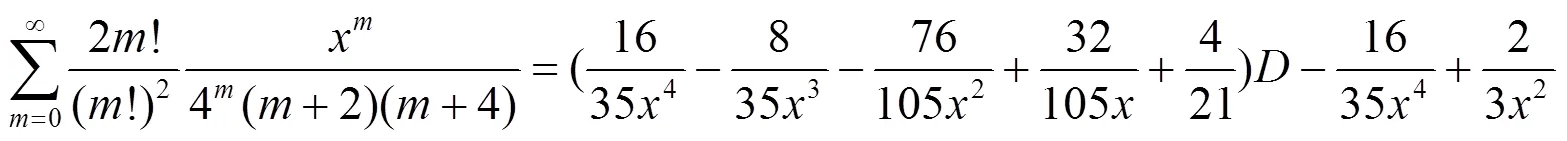
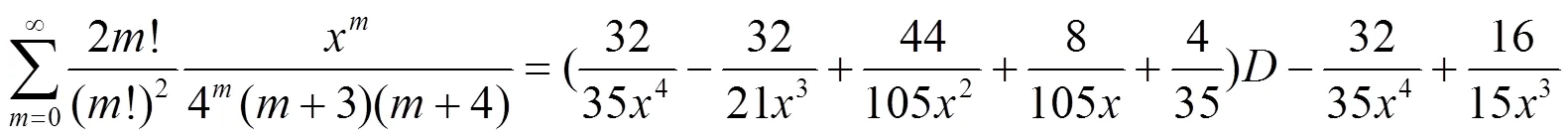
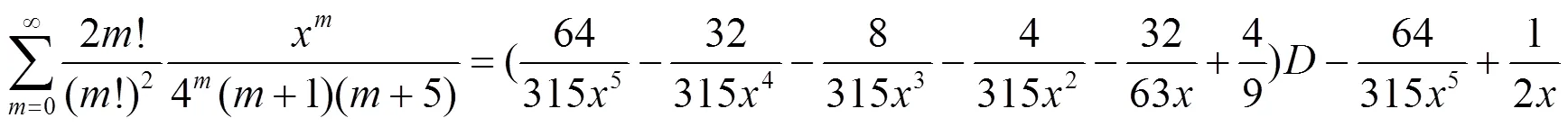
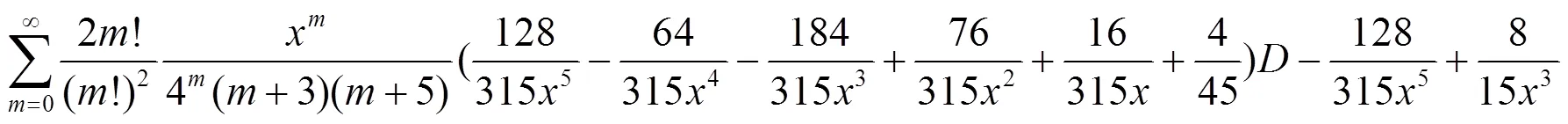
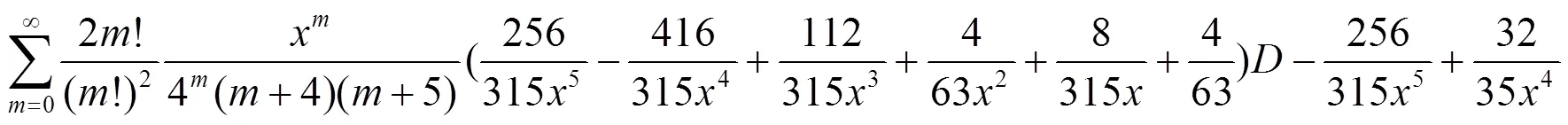
c. 分母含有3個(gè)因子乘積的二項(xiàng)式系數(shù)級(jí)數(shù)恒等式.

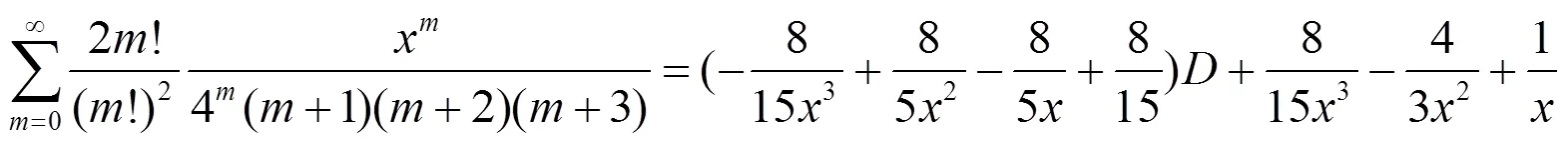
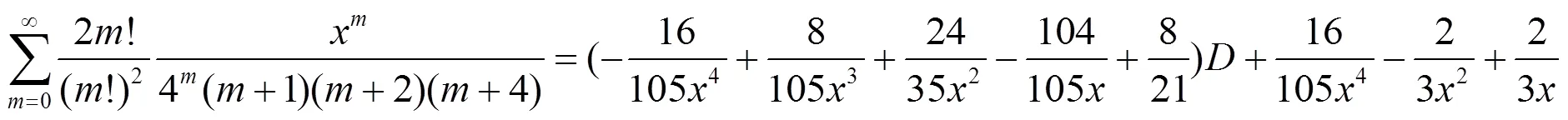
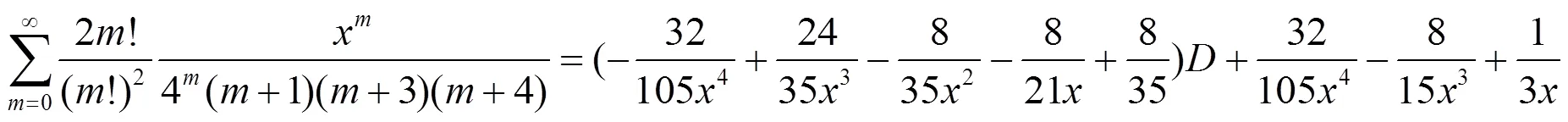
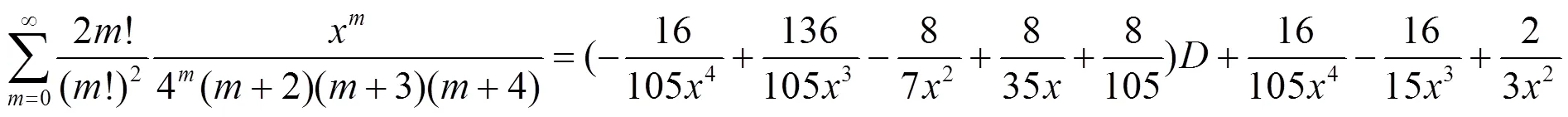
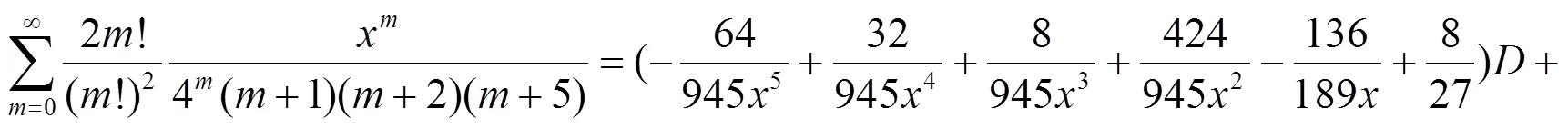
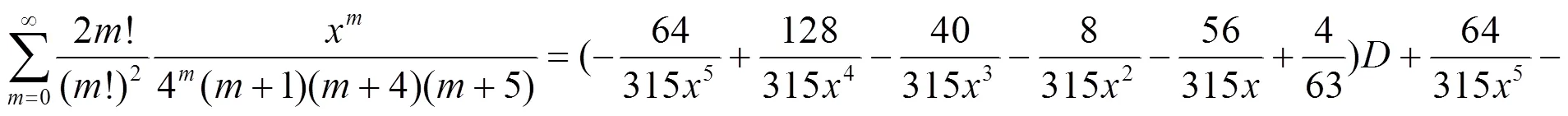
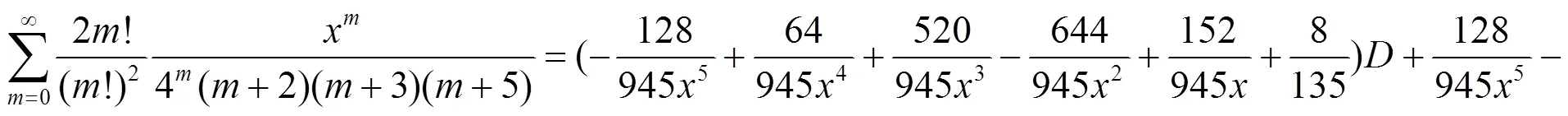
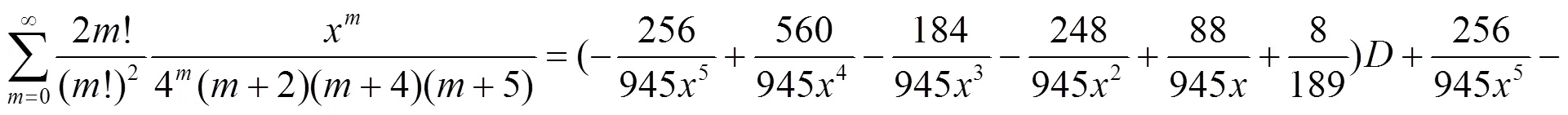
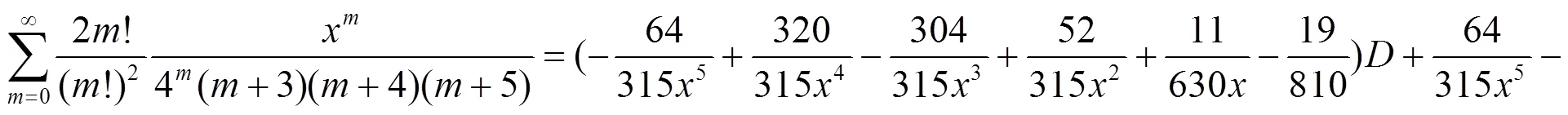
d. 分母含有4個(gè)因子乘積的二項(xiàng)式系數(shù)級(jí)數(shù)恒等式.

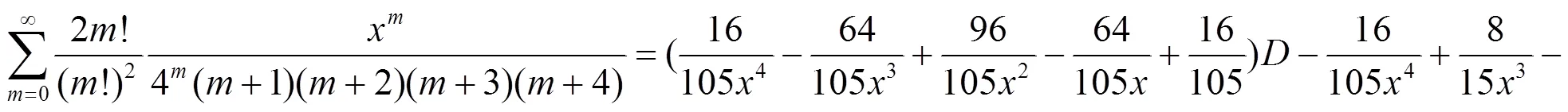
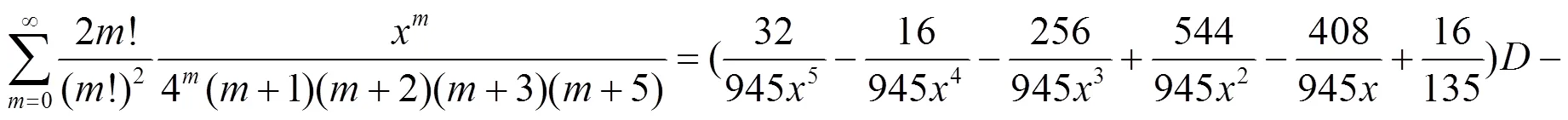
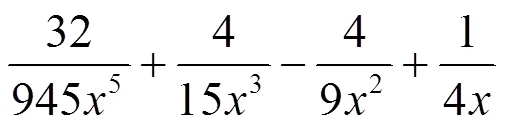
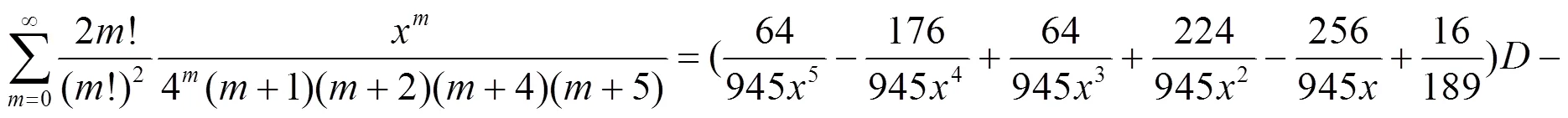
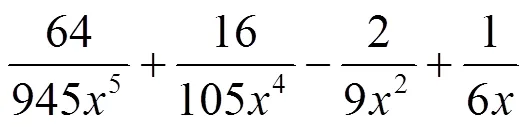
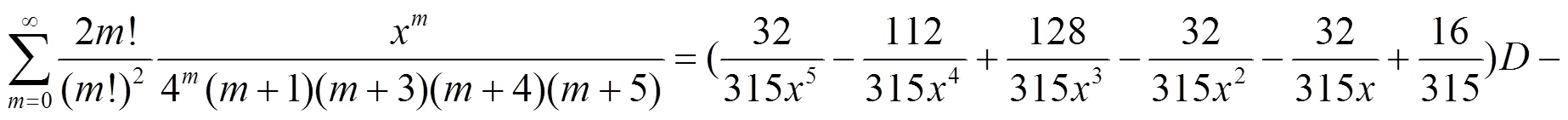
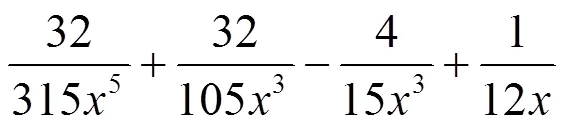
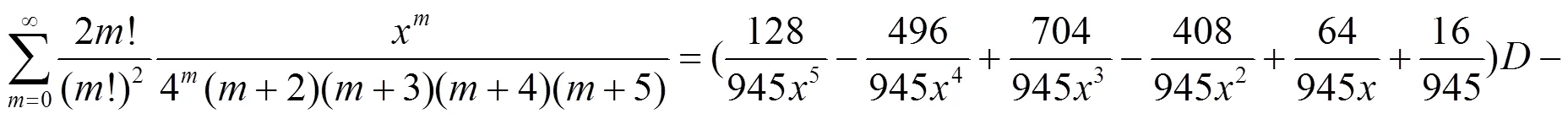
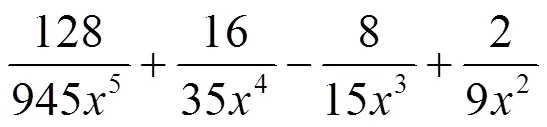
e. 分母含有5個(gè)因子乘積的二項(xiàng)式系數(shù)級(jí)數(shù)恒等式.

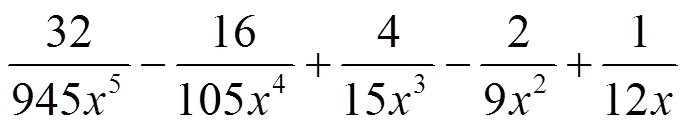
證明 a. 對(duì)級(jí)數(shù)(1)式左端裂項(xiàng):

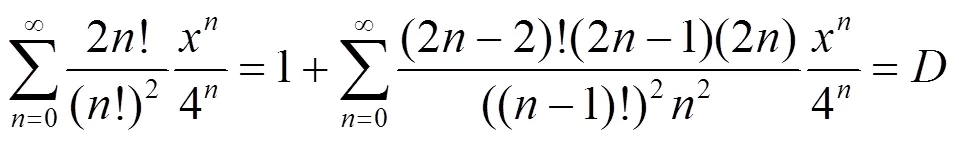
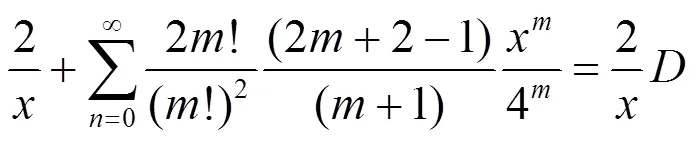
整理得到(2)式.

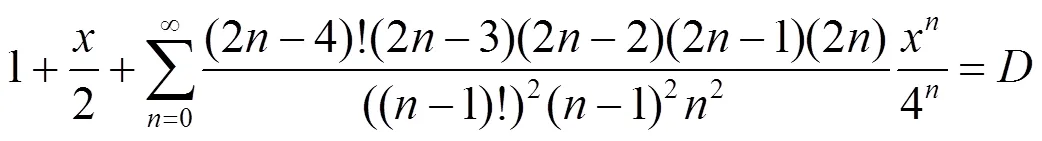
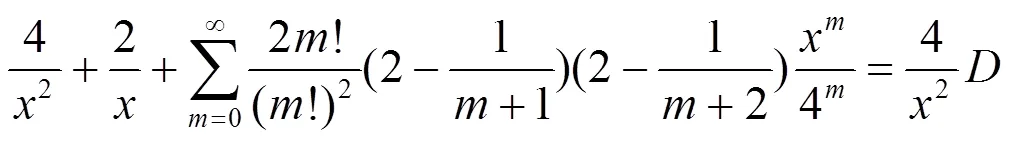
即:

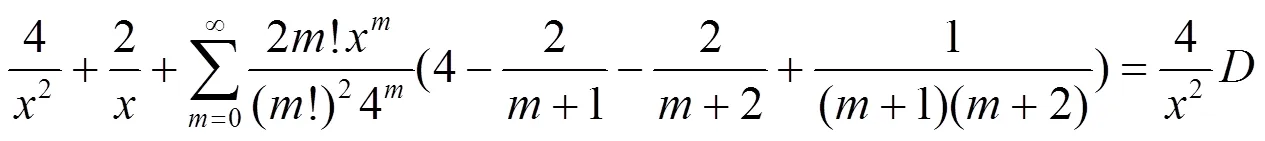
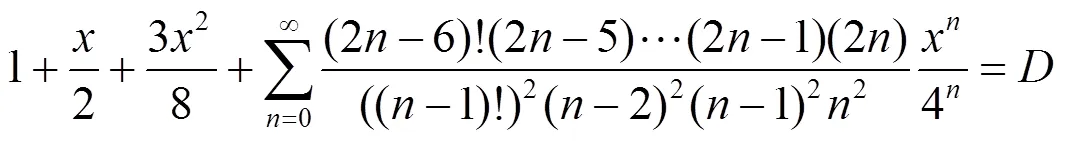
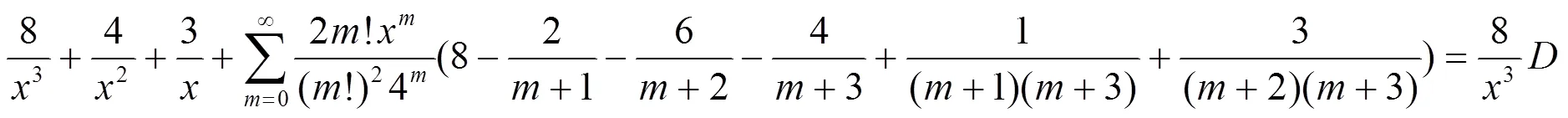
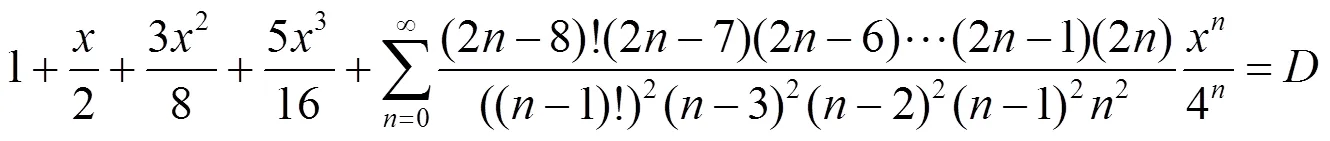
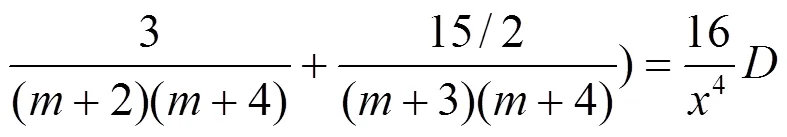
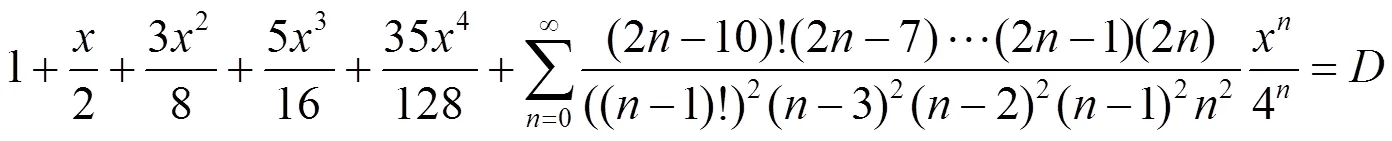
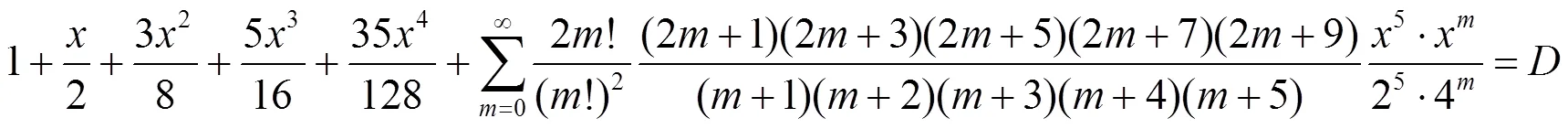
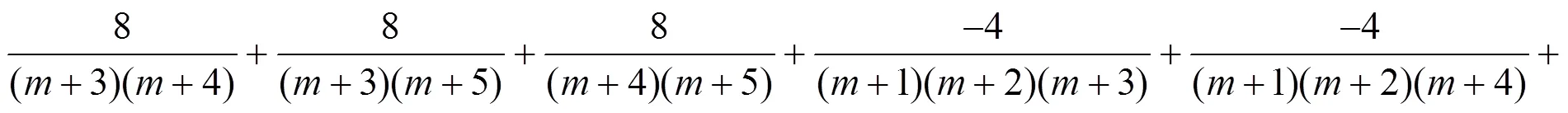
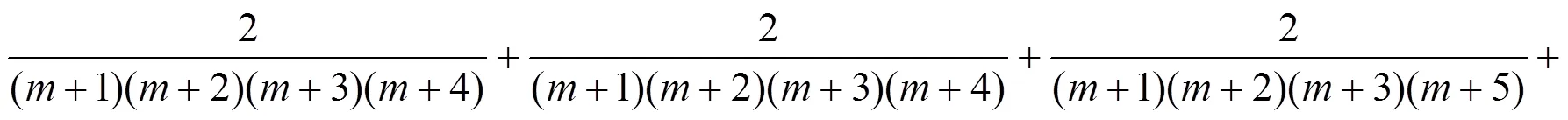
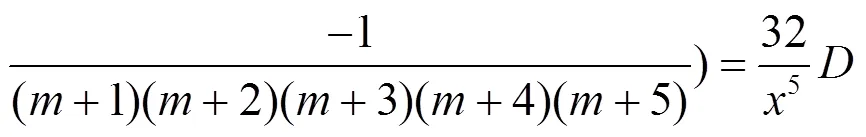
在(36)式中含有2個(gè)因子的分式10個(gè), 3個(gè)因子的分式有10個(gè), 4個(gè)因子的分式有5個(gè), 5個(gè)因子的分式1個(gè), 對(duì)(36)式實(shí)行下列運(yùn)算得到分母含有1, 2, 3, 4個(gè)因子的組合數(shù)的恒等式.

(a) 將(36)式的所有含有因子的分式化成部分分式, 得:

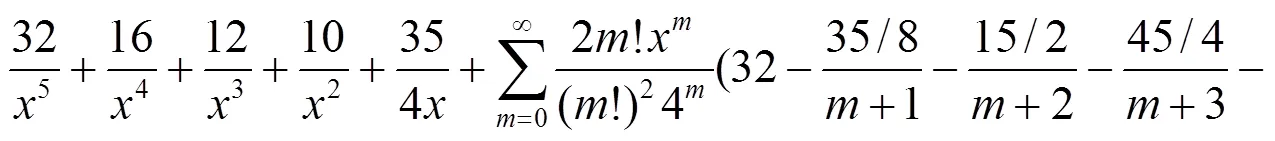
(b) 對(duì)于(36)式, 首先保留2個(gè)因子的分式, 其他分式化成部分分式.其次對(duì)這些2個(gè)因子的分式, 每次保留1個(gè), 其余化成部分分式, 得到:

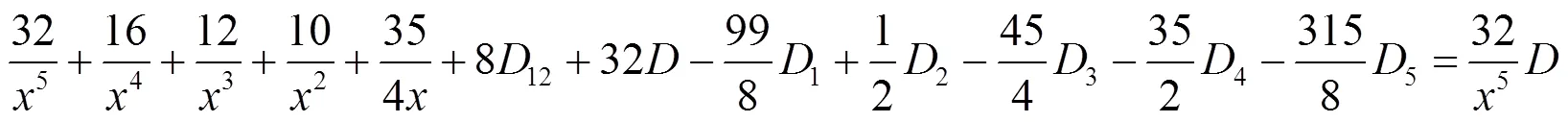
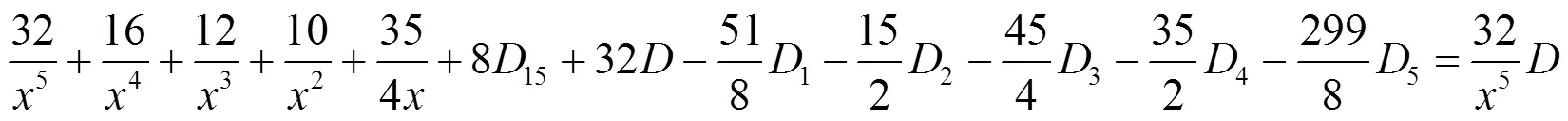
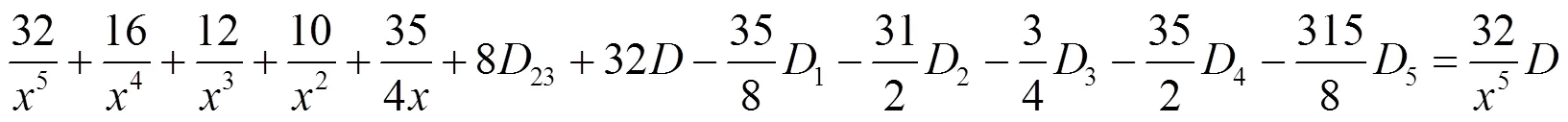
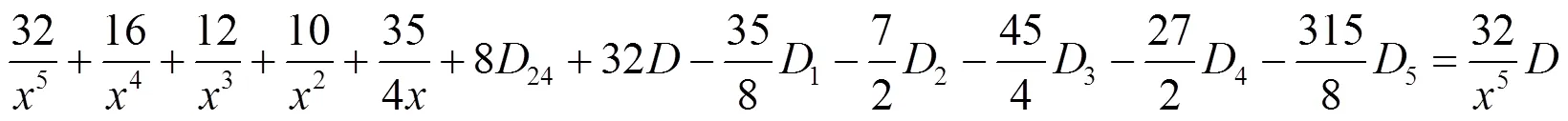
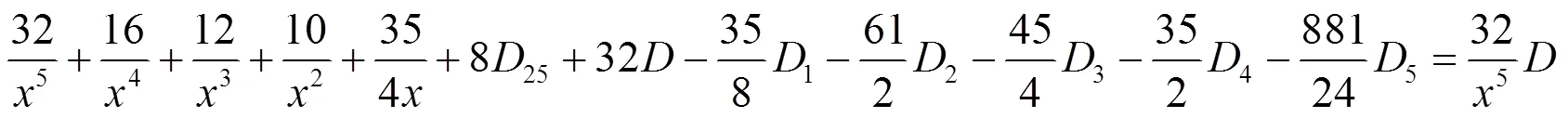
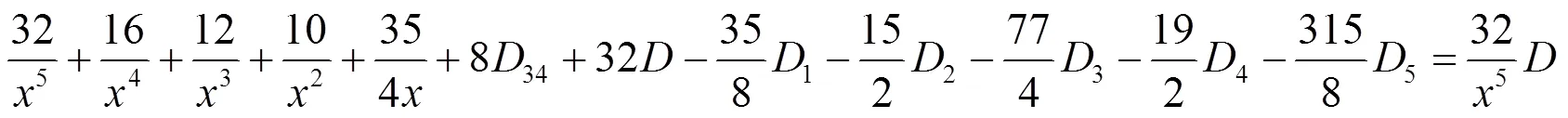
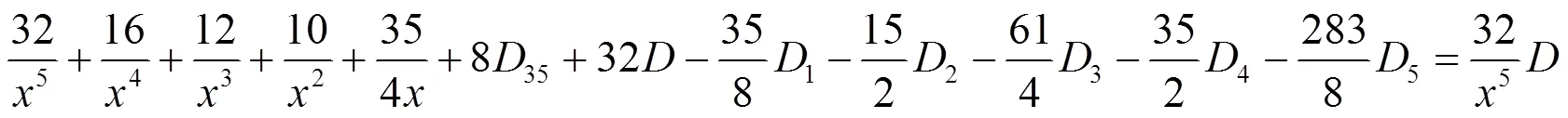
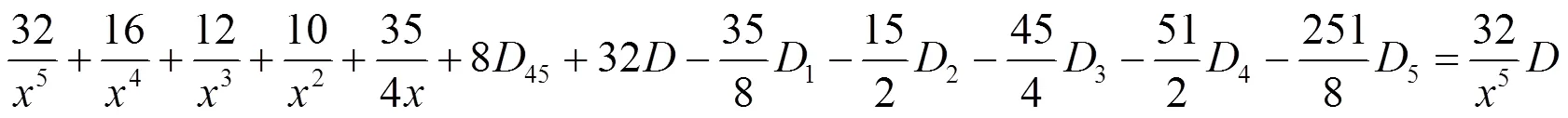
(c) 對(duì)于(36)式, 首先保留3個(gè)因子的分式, 其他分式化成部分分式. 其次對(duì)這些3個(gè)因子的分式, 每次保留1個(gè), 其余化成部分分式, 得到:

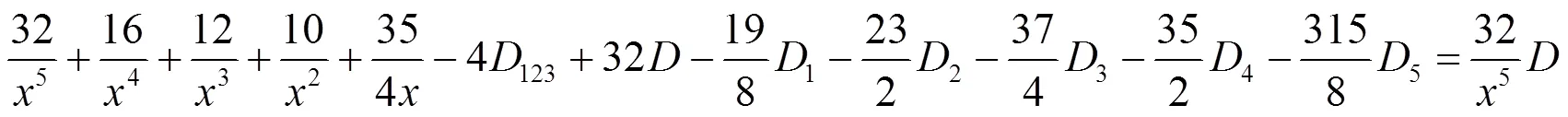
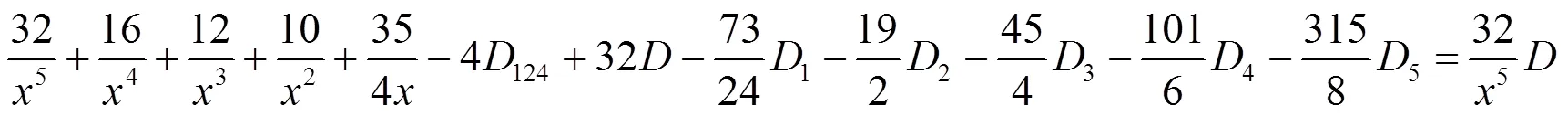
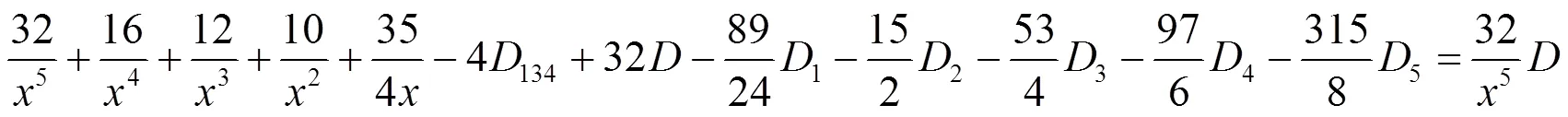
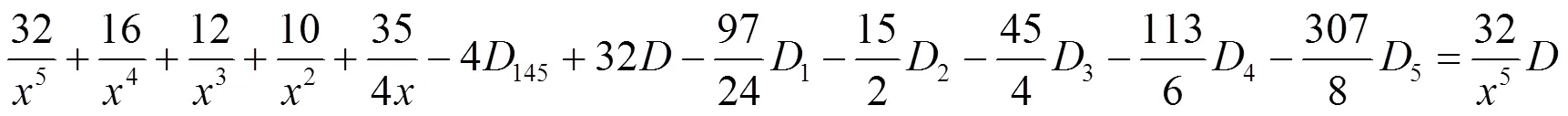
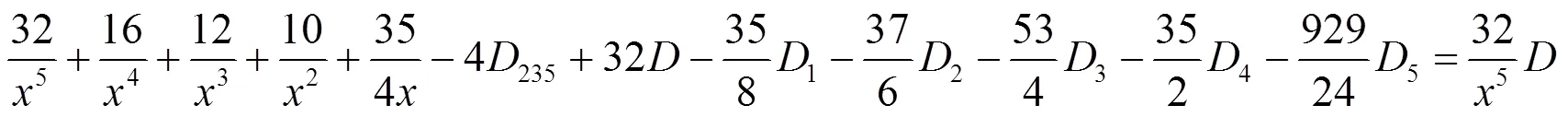
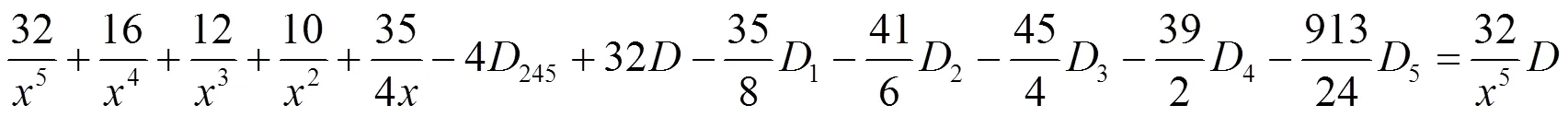
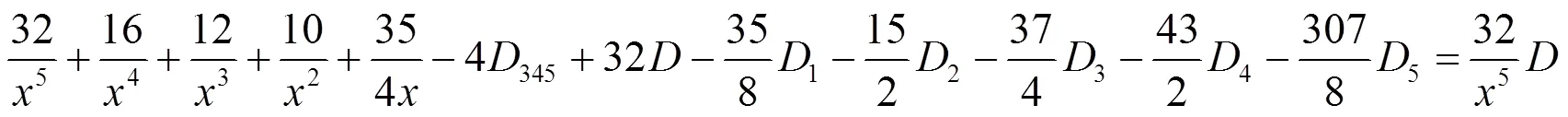
(d) 對(duì)于(36)式, 首先保留4個(gè)因子的分式, 其他分式化成部分分式. 其次對(duì)這些4個(gè)因子的分式, 依次保留1個(gè), 其余化成部分分式, 得到:

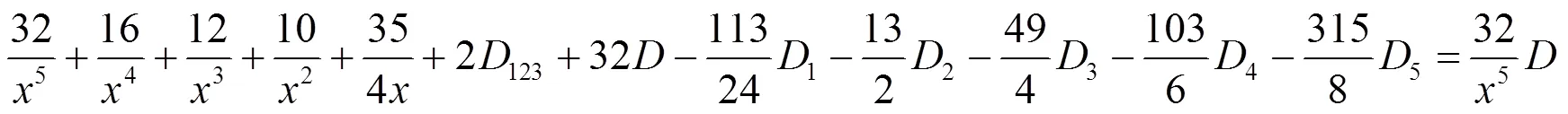
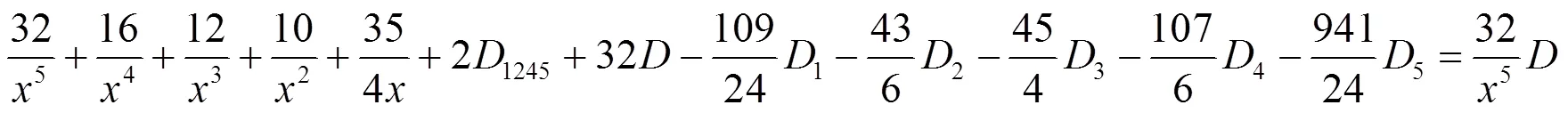
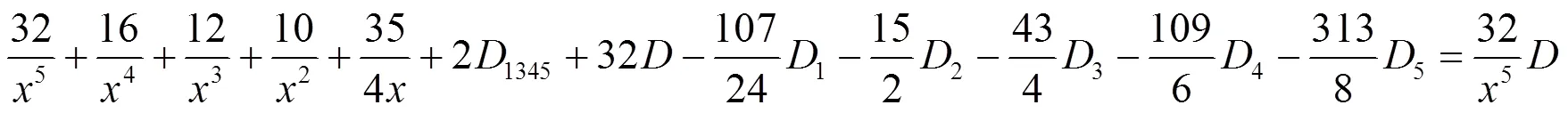
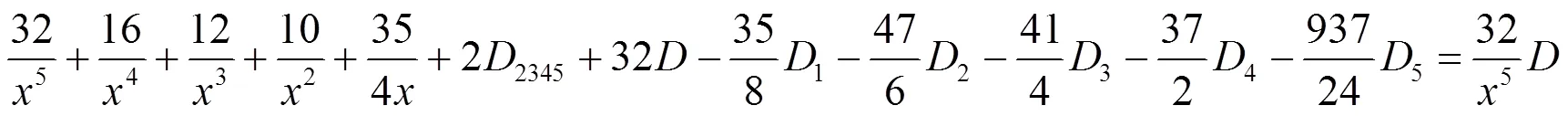
(e) 對(duì)于(36)式, 首先保留5個(gè)因子的分式, 其他分式化成部分分式, 得到:

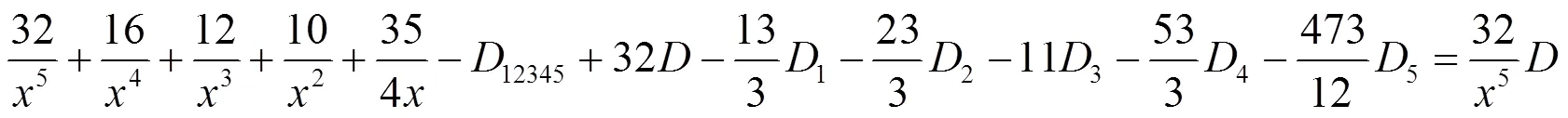
2 推論

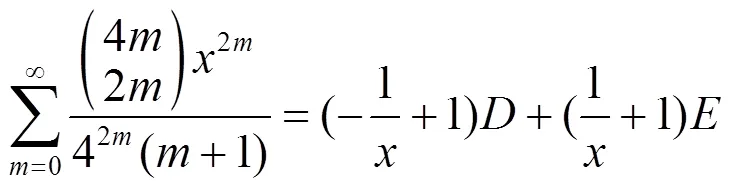
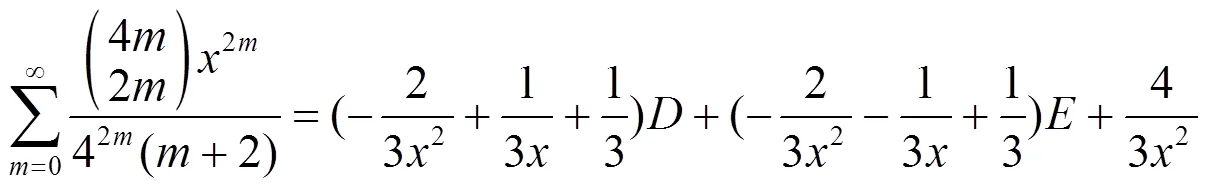
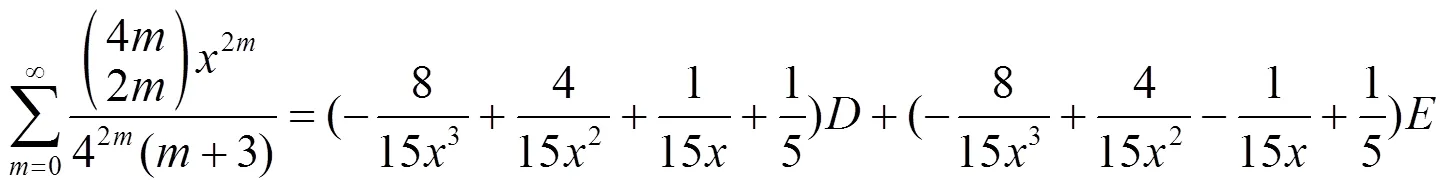
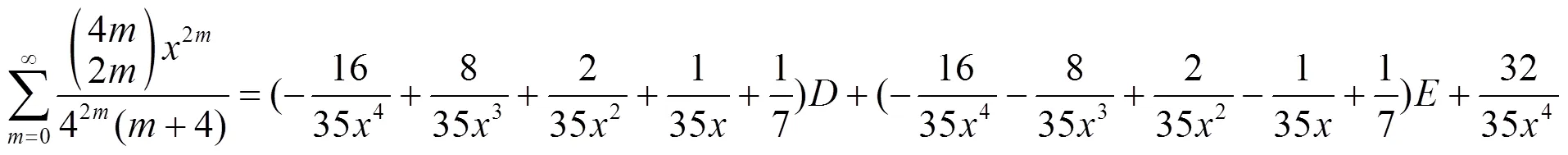
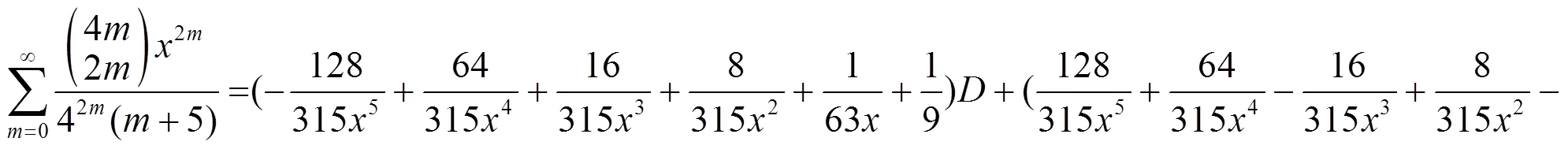
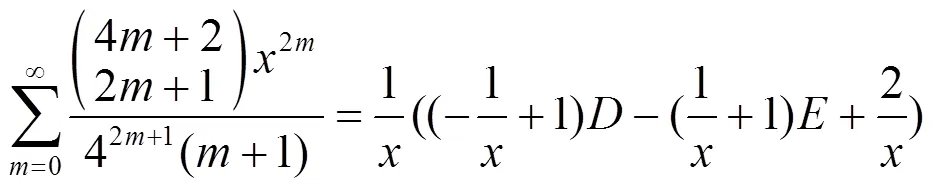
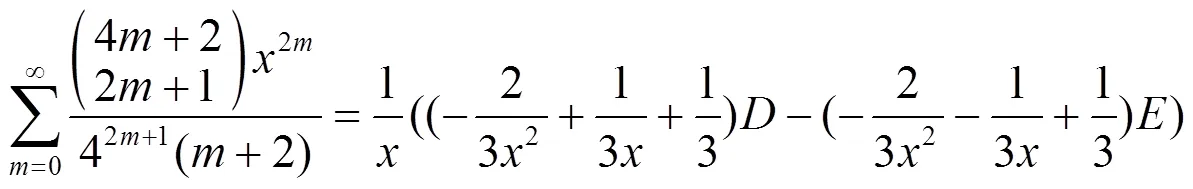
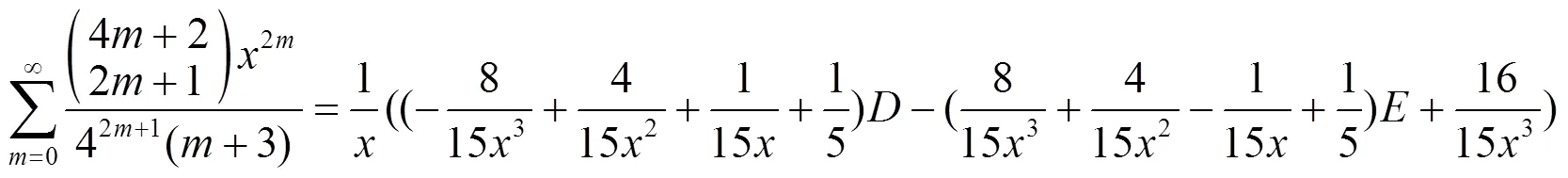
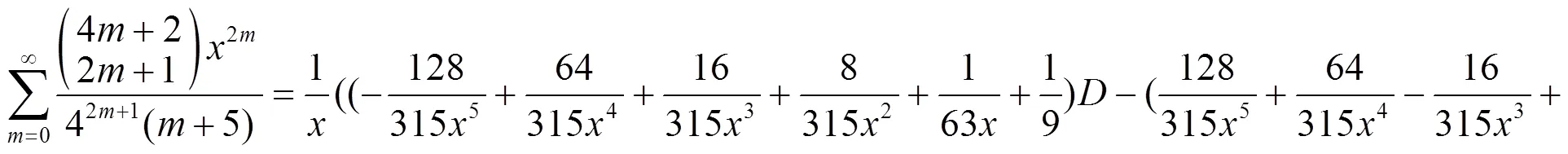
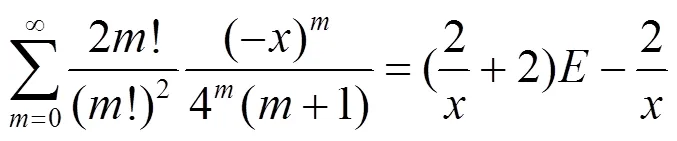
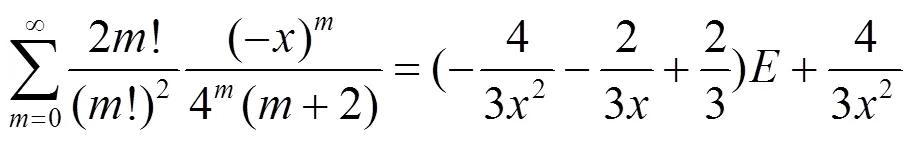
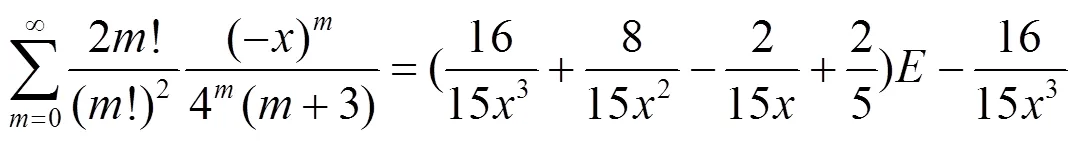
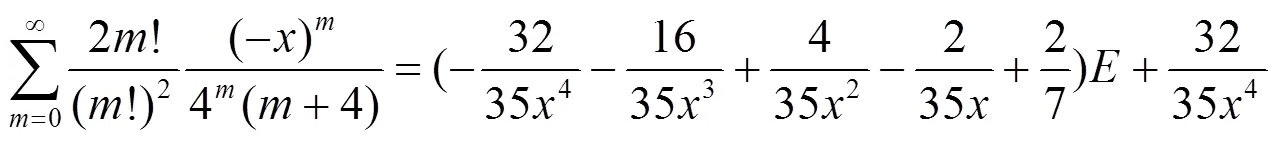
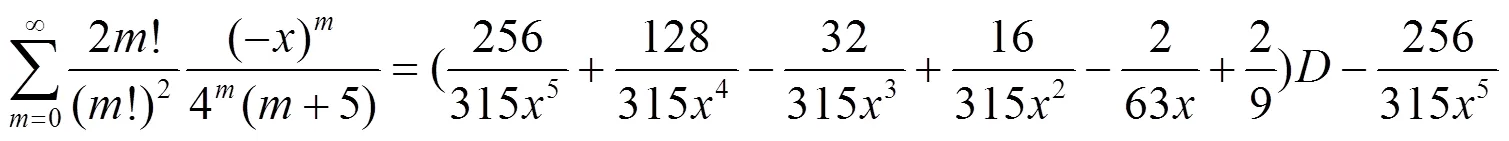
3 數(shù)例

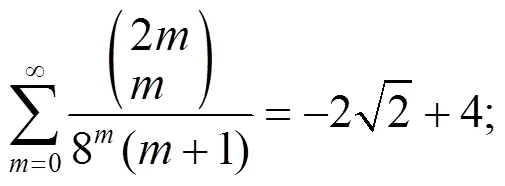
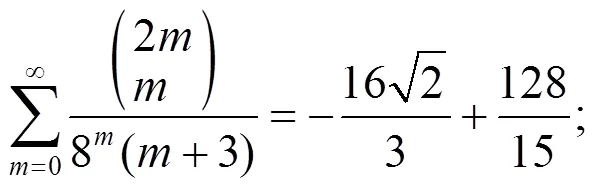
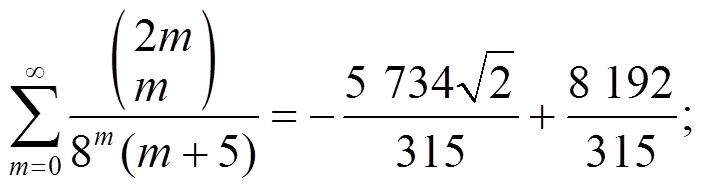
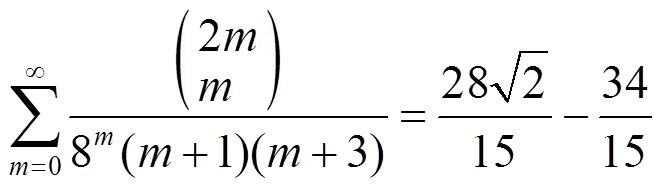
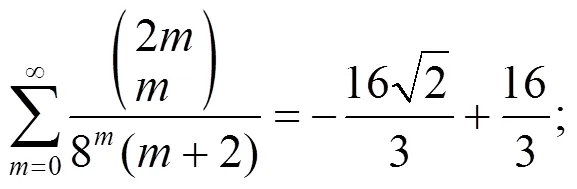
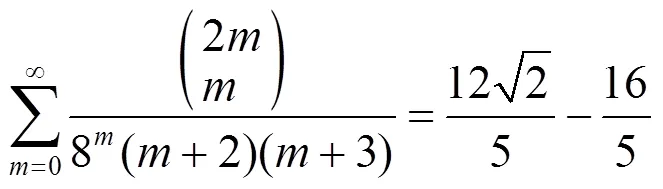
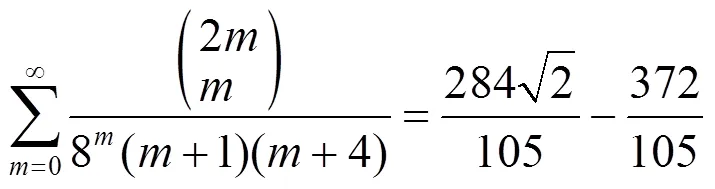
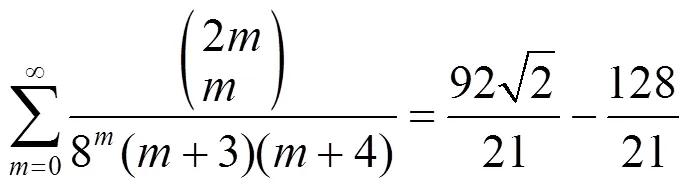
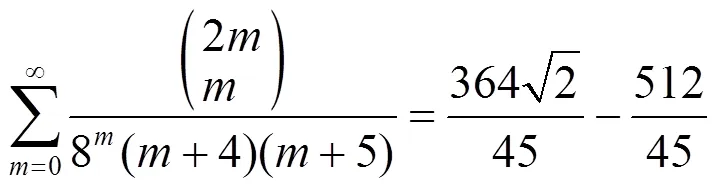
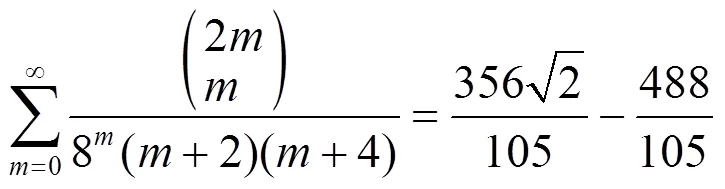
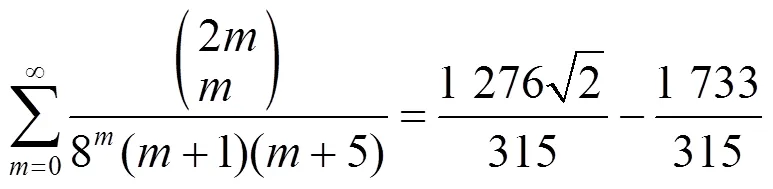
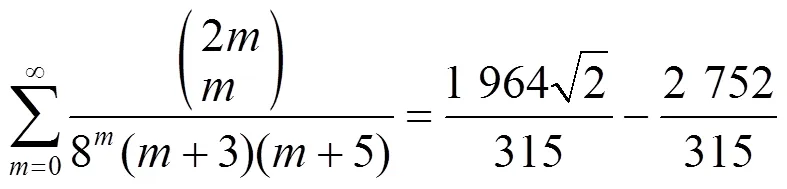
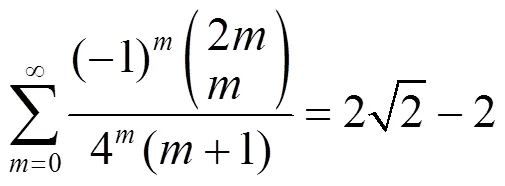
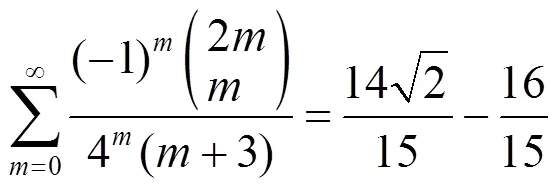
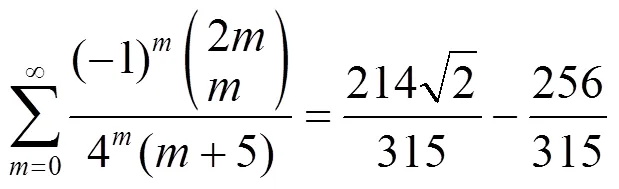
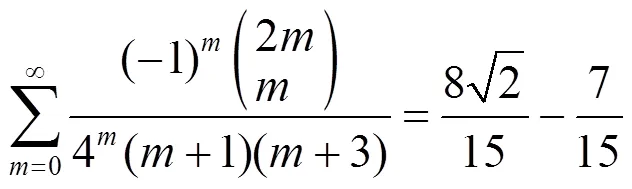
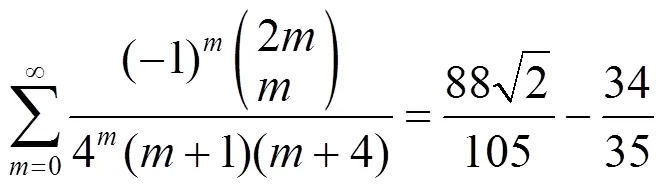
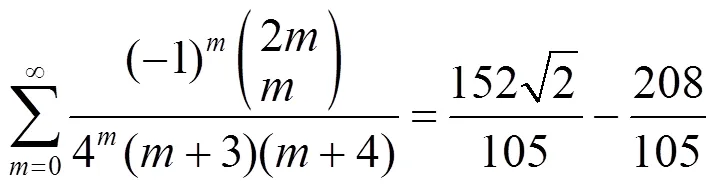
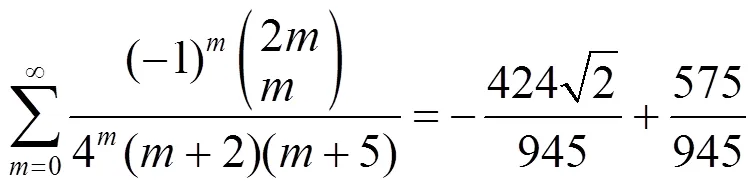
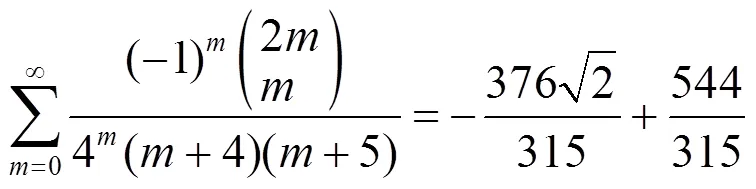
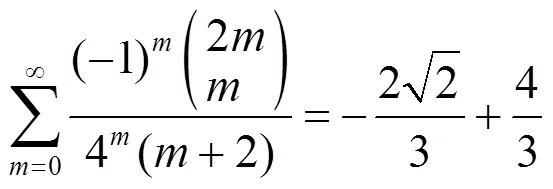
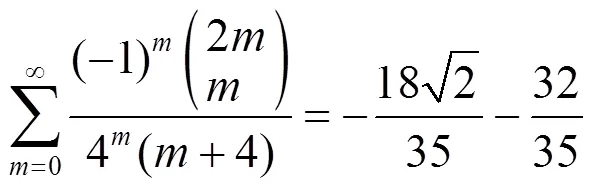
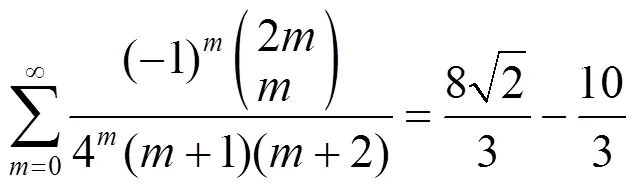
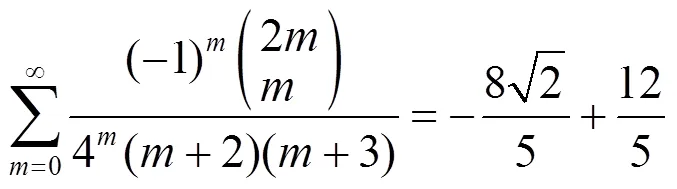
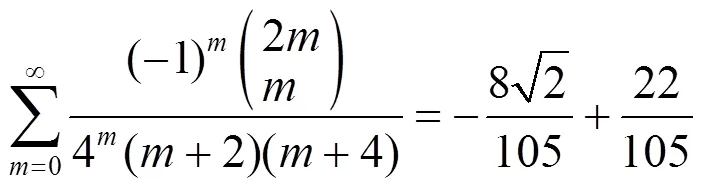
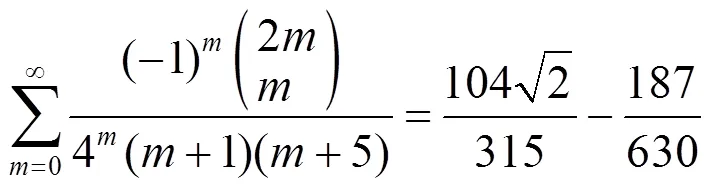
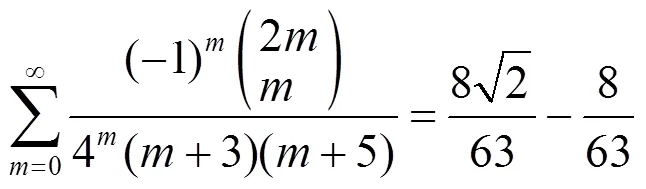
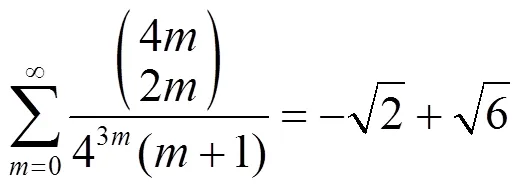
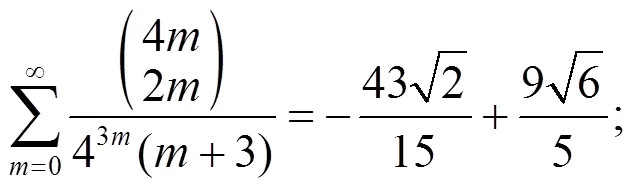
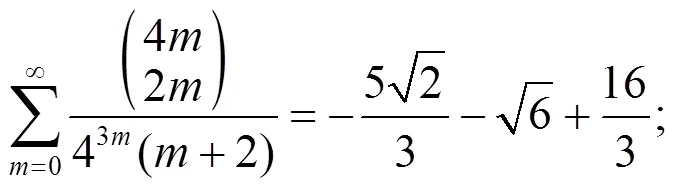
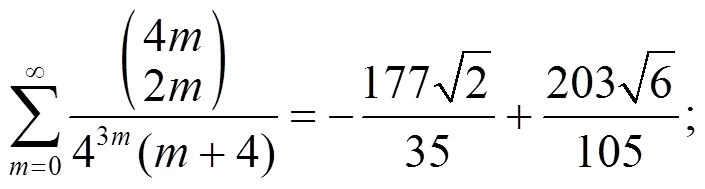
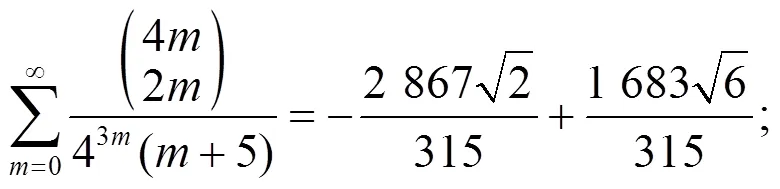
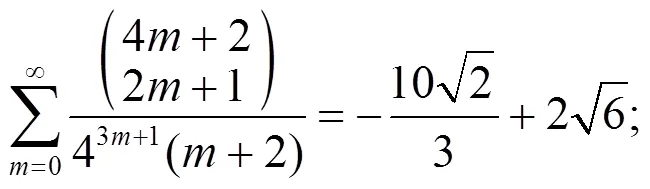
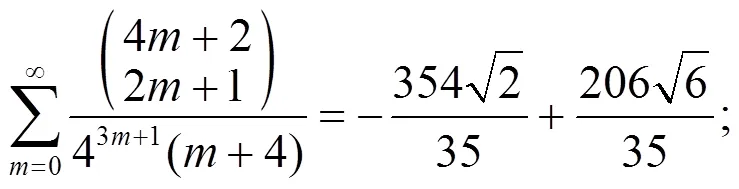
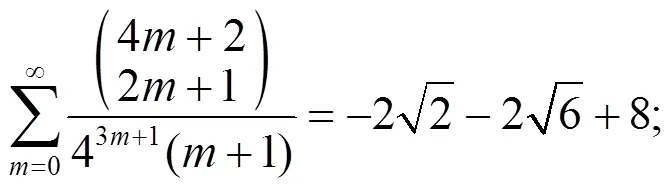
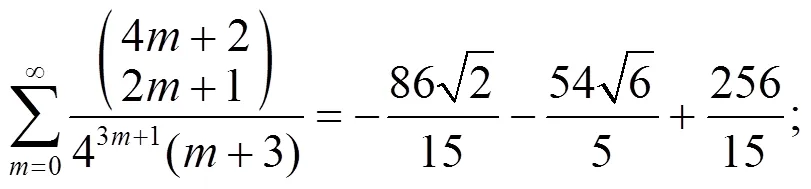
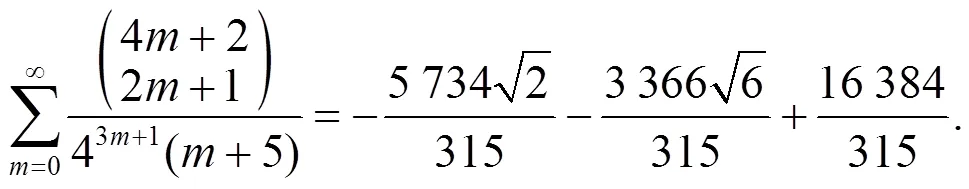
[1] Sury B, Wang T N, Zhao F Z. Some identities involving of binomial coefficients[J]. Journal of Integer Sequences, 2004, 7(2): 1-12.

[2] Sofo A. General properties involving reciprocal of Binomial coefficients[J]. Journal of Integer Sequences, 2006, 9(4): 1-13.

[3] Sprugnoli R. Sums of reciprocal of the central binomial coefficients[J]. Integral: Electronic Journal of Combinatorial Number Theory, 2006, 6: 1-17.

[4] Yang J H, Zhao F Z. Sums involving the inverses of binomial coefficients[J]. Journal of Integer Sequences, 2006, 9(4): 1-11.

[5] Borwein J M, Girgensohn R. Evaluation of binomial series[J]. Aequationens Math, 2005, 70: 25-36.

[6] Amghibech S. On sum involving Binomial coefficient[J]. Journal of Integer Sequences, 2007, 10(2): 1-4.

[7] 編寫(xiě)組. 數(shù)學(xué)手冊(cè)[M]. 北京: 人民教育出版社, 1979: 226

On one class series identities of binominal coefficients

JI Wan-hui, HEI Bao-li

(Department of Basic, Yinchuan Energy College, Yinchuan 750105, China)

Using one known series, several new series of binominal coefficients were gotten by splittingitems. These denominators of series contains different the multiplication of one to five factors and binominal coefficients, and some identities of series of numbers values of binominal coefficients were put forward. The method ofsplititems offered in this paper was a new combinatorial analysis way and a elementary method to construct new series.

binomial coefficients; split terms; series; form closed; identity

O 173

1672-6146(2012)04-0004-010

10.3969/j.issn.1672-6146.2012.04.002

2012-09-24

銀川能源學(xué)院科研基金項(xiàng)目(2011-37-15)

及萬(wàn)會(huì)(1942-), 男, 教授, 研究方向?yàn)閿?shù)論. E-mail: jiwanhui2008@163.com

(責(zé)任編校: 劉曉霞)