A210Pbexmass balance model in cultivated soils in consideration of the radionuclide diffusion?
ZHANG Yun-Qi(張云奇),ZHANG Xin-Bao(張信寶),and LONG Yi(龍翼)
1Sichuan Agriculture University,Chengdu 611830,China
2Shandong Provincial Key Laboratory of Water and Soil Conservationamp;Environment Protection,Linyi University,Linyi 276000,China
3Institute of Mountain Hazards and Environment,Chinese Academy of Sciences,Chengdu 610041,China
A210Pbexmass balance model in cultivated soils in consideration of the radionuclide diffusion?
ZHANG Yun-Qi(張云奇),1,2,?ZHANG Xin-Bao(張信寶),3and LONG Yi(龍翼)3
1Sichuan Agriculture University,Chengdu 611830,China
2Shandong Provincial Key Laboratory of Water and Soil Conservationamp;Environment Protection,Linyi University,Linyi 276000,China
3Institute of Mountain Hazards and Environment,Chinese Academy of Sciences,Chengdu 610041,China
The existing210Pbexmass balance models for the assessment of cultivated soil erosion are based on an assumption that210Pbexis quite evenly mixed within the plough layer.However,the amount of210Pbexdistributed in the soils below the plough depth,like a downward tail in the lower part of the210Pbexprofile,has been largely ignored.In fact,after the initial cultivation of undisturbed soils,210Pbexwill diffuse downward from plough layer to the plough pan layer due to the concentration gradient.Assuming210Pbexinventory is constant,the depth distribution in the two layers of the cultivated soils will achieve a steady state after continuous cultivation for 10.37 years,when210Pbexis evenly distributed in the soils of the plough layer with an exponential concentration decline with depth in the soils of the plough pan layer,and the210Pbexconcentration at any depth will be invariable with time.The work reported in this paper attempts to explain the formation of the210Pbextail in the soil profile below the plough depth by theoretical derivation of the210Pbexdepth distribution process in the two layers of the cultivated soils,propose a210Pbexmass balance model considering210Pbexdiffusion based on the existing model,and discuss the in fl uence of the210Pbextail to the existing model.
Cultivated soils,210Pbexdepth distribution,Diffusion,Mass balance model,Soil erosion
I.INTRODUCTION
Because of temporal and spatial limitations associated with the application of137Cs technique to estimating rates of soil erosion and sediment redistribution[1–4],the use of210Pbexmeasurement has attracted increasing attention,as an alternative approach[5–9].Unlike137Cs,which is an artificial radionuclide that is released into the environment as a result of atmospheric test of nuclear weapons,210Pb is a natural product of the238U decay series,derived from the decay of gaseous222Rn,the daughter of226Ra[10,11].226Ra is found naturally in most soils and rocks and the generated210Pb is in equilibriumwithitsparent.Asmallquantityof222Rndiffuses upward from the soil,introduces210Pb into the atmosphere and provides an input of this radionuclide to surface soils and sediments,which is not in equilibrium with its parent226Ra. This fallout component is termed unsupported or excess210Pb (210Pbex)[12,13].Like137Cs,210Pbexis shown to have a strong affinity for soil and sediment particles.Upon reaching the soil surface,210Pbexis quickly and strongly adsorbed by the surface soil,and the subsequent redistribution within the landscape will reflect the movement of soil and sediment particles associated with soil erosion and sediment transport processes[14].Unlike the time-dependent fallout of137Cs, the atmospheric fallout of210Pbexis essentially constant for its natural origin[15],and the210Pbexinventory in soils can be invariable because the subsequent fallout provides an input to surface soils that is in equilibrium with the radioactive decay of the existing210Pbexin the soils.
In the reported works,the profiles of soils from undisturbed land show that the maximum concentrations of210Pbexoccur at the surface horizon and decline exponentially with depth(Fig.1(a),1(c)),while in the profiles of cultivated soils the210Pbexconcentration is almost uniform throughout the plough layer as a result of mixing caused by tillage (Fig.1(b),1(d)).For the cultivated soils,it is also obvious that an amount of210Pbexdistributed in the soils below the plough depth,like a downward tail in the lower part of the profile(Fig.1(b),1(d)).The210Pbextail in the profile demonstrates that the maximum210Pbexconcentrations occur at the bottom of plough layer and decline exponentially with depth. However,the amount of210Pbexdistributed below the plough depth has been largely ignored to date,suggesting that210Pbexis quite evenly distributed and restricted to the plough layer. The existing210Pbexmass balance models for the assessment of cultivated soil losses have similarly not taken into account the amount of210Pbexdistributed below the plough depth.
Walling and He[16]developed a mass balance model for estimating erosion rates on cultivated soils from210Pbexmeasurement.Based on this mass balance model,and ignoring the freshly deposited210Pbexremoved by erosion and influence of the particle size correction factor,Zhang et al.proposed a simplified210Pbexmass balance model in the steady state for estimating erosion rates on cultivated soils[18],which can be expressed as:
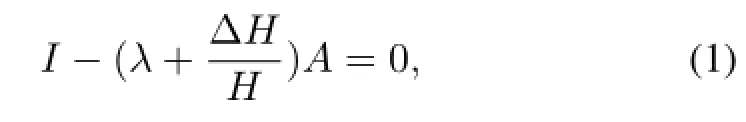
where A is the210Pbexinventory(mBq/cm2),λ is the decay constant of210Pbex(/yr),ΔH is the erosion rate(cm/yr)and H is the plough depth(cm).
The210Pbexloss proportion(l)relative to the local reference inventory(Aref,in mBq/cm2)for an eroding site can be expressed as:
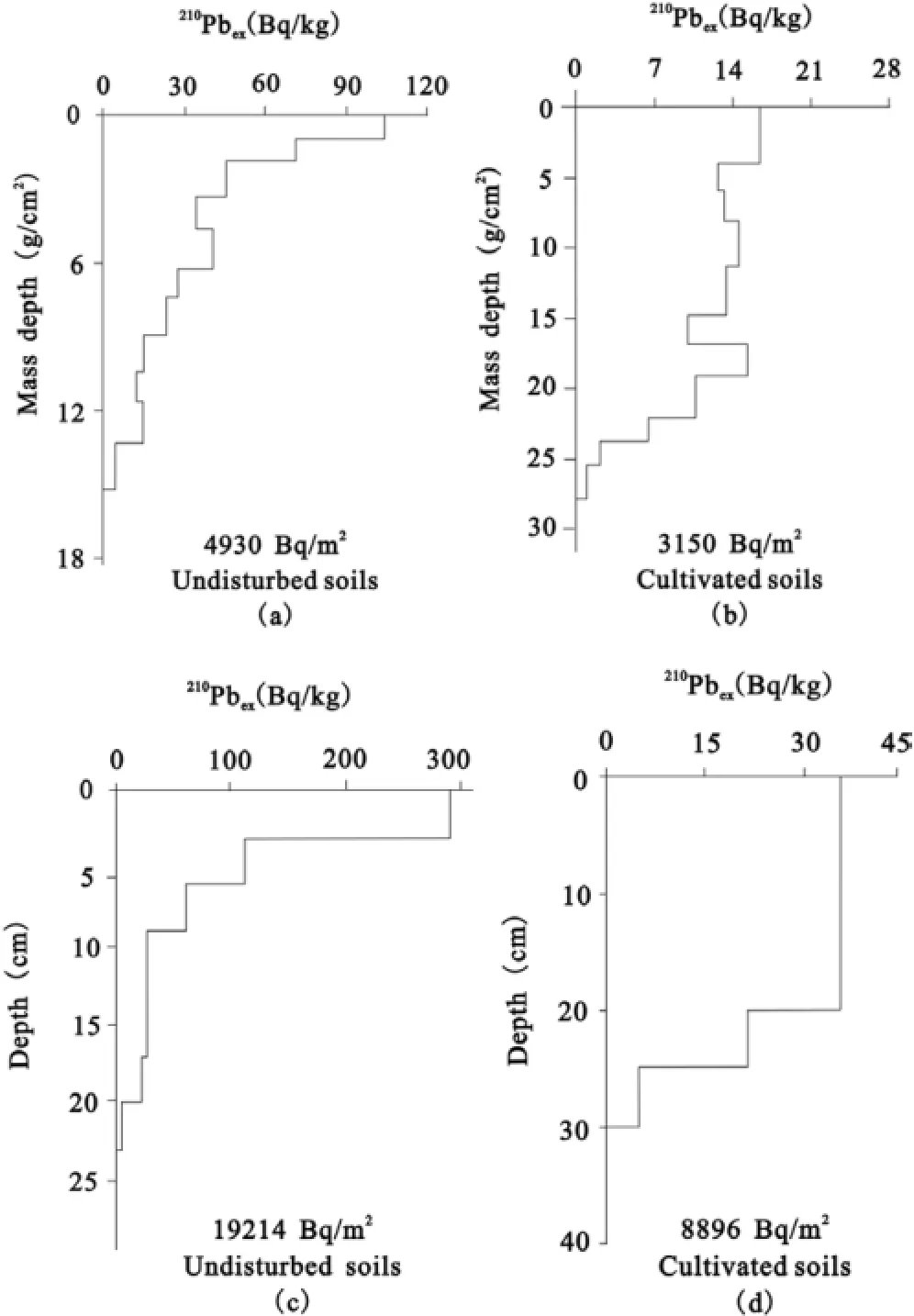
Fig.1.Typical210Pbexprofiles in undisturbed and cultivated soils. (a),(b),Moorlake catchment,UK[16];(c),(d),Sichuan Hilly Basin, China[17].
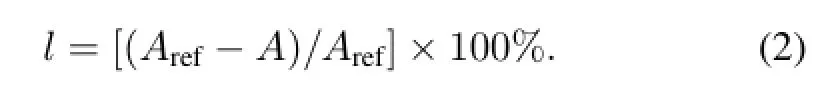
Assuming the210Pbexdeposition flux is constant,the erosionratecanbecalculatedasEq.(3)accordingtotherelationship between the deposition flux and the reference inventory (I=λAref).
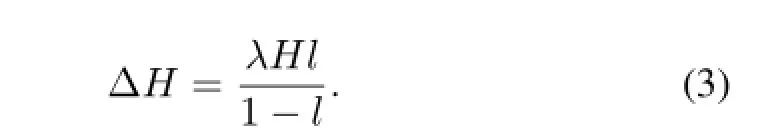
So,the existing mass balance models(existing model for short)are based on the assumption that210Pbexis quite evenly distributed within the plough depth.Nevertheless,neglecting210Pbexdistributed below the plough depth,more or less, would consequentially influence the application of existing model to soil erosion estimation.So,it is needed to know the influence level,and whether or not the existing model is applicable to soil erosion estimation as usual.In this paper,we attempt to explain the formation of210Pbextail in the lower part of profile by theoretical derivation of the210Pbexdepth distribution process in the plough and plough pan layers of the cultivated soils,propose a210Pbexmass balance model in consideration of the radionuclide diffusion(revision model for short),and discuss the influence of the210Pbexdistributed below the plough depth on the application of existing model to erosion estimation.
II.210PBEXDISTRIBUTION PROCESSES IN CULTIVATED SOILS
A.Mechanism and process analysis
In fact,210Pbexis quite evenly mixed by tillage within the plough layer,and there is little210Pbexdistributed below the plough depth at the beginning of cultivation,because the plough depth is usually no less than the measured depth range of210Pbexdistribution in the undisturbed soils before tillage. However,this initial depth distribution state is unsteady,and the radionuclide moves downward from the plough layer to the plough pan layer.Radionuclide downward movements in soil include diffusion and migration processes[19].210Pbexdiffusion in soil is the process of radionuclide movement that is caused by the concentration gradient in the ionic or molecular form,which means that the radionuclide is only able to diffuse from a high concentration to a low concentration.In210Pbexmigration in soil,its downward movement is caused by transportation via media,such as adsorbed solid particles and dissolved fluids,which means that the migration is independent of the concentration gradient,and the radionuclide is able to move either from a high concentration to a low concentration or vice versa.For a soil profile in which the maximum radionuclide occurs at the top horizon and declines monotonically with depth,diffusion can be considered the predominant radionuclide movement process over the profile,while the radionuclide migration processes can be neglected[20–24].Thus,it can be assumed that the diffusion process dominates the formation of the210Pbextail in the profile of the plough pan layer.
At the beginning of cultivation,210Pbexis quite evenly distributed within the plough layer;after the initial cultivation, the210Pbexdiffuses from the plough layer to the plough pan layer due to the concentration gradient;the210Pbexinventory of the plough layer decreases while that of plough pan layer increases;and the210Pbextail in the profile of the cultivated soils progressively comes into being closely below the interface between the two layers(Fig.1(b),1(d)).Assuming the210Pbexinventory is constant,the210Pbexconcentration at any soil depth will be invariable after a period of diffusion,and the210Pbexdepth distribution in the two layers of cultivated soils will achieve a steady state.
B.The depth distribution in the unsteady state
Assuming no soil erosion or deposition occurs,when210Pbexis evenly mixed within the plough layer by the initial cultivation of undisturbed soils.Subsequently,the210Pbexstarts moving from the plough layer to the plough pan layer. Taking the diffusion process into account,the210Pbexmovement in the soils of plough pan layer follows Fick’s second law of diffusion[25],which is:
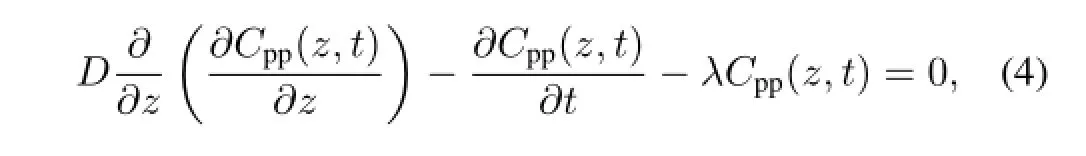
where D is the effective diffusion coefficient(cm2/yr),Cppis the210Pbexconcentration in the soils of the plough pan layer (mBq/cm3),z is the depth downward from the interface betweentheploughandploughpanlayers(cm),andtisthetime elapsed since210Pbexwas well mixed within the plough layer (yr).When z→0,the210Pbexconcentration of the interface soils is equal to the value of the210Pbexconcentration at any depth of the plough layer.
By diffusion,the total210Pbex(A,in mBq/cm2)is redistributed between the plough and plough pan layers,which means:

where Apand Appare the210Pbexinventory of the plough layer and plough pan layer,respectively.
The210Pbexinventory in the soils of the plough layer can be calculated as:

Assuming the plough pan layer can be treated as a semiinfinite homogeneous porous medium,the process of210Pbexdiffusion from the plough layer to the plough pan layer can be considered as the infinite thin layer radionuclide resource diffusing to a one-dimensional semi-infinite medium.Thus, according to the Gauss solution of the Fick’s second law[26], by combining Eqs.(4),(5)and(6),the210Pbexconcentration variation with time in the soils of the plough layer can be expressed as:
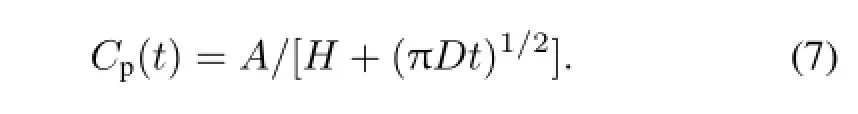
And the variation of the210Pbexconcentration with time anddepthinthesoilsoftheploughpanlayercanbeexpressed as:
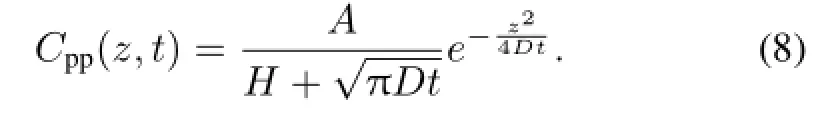
By integrating the two sides of Eq.(8)over depth z,the proportion of the210Pbexinventory of the plough pan layer to the total210Pbexinventory in the cultivated soil,namely the210Pbexdiffusion proportion,can be expressed as:
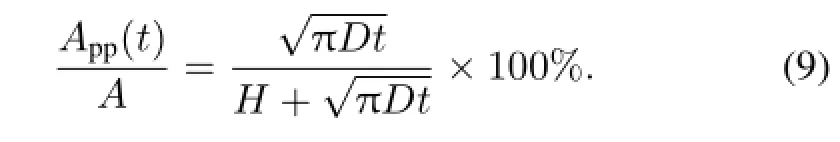
The above theoretical derivation,namely Eqs.(4)–(9),can also apply to the137Cs depth distribution process in cultivated soils.However,unlike137Cs,the210Pbexdepth distribution in the two layers of the cultivated soils finally achieves a steady state after a period of diffusion,assuming the210Pbexdeposition is constant over time.Before the steady state,the210Pbexconcentration at any depth of the two layers varies with time. If the effective diffusion coefficient D is known(which can be obtained by experiments),Eq.(7)can be used to simulate the210Pbexconcentrationvariationwithtimeinthesoilsofthe plough layer,and Eq.(8)can be used to simulate the210Pbexconcentration variation with time and depth in the plough pan layer.
C. The depth distribution in the steady state
After achieving a steady state,the210Pbexconcentration at any depth of the cultivated soils is invariable with time,viz.?Cpp(z,t)/?t=0,and Eq.(4)can be written as:
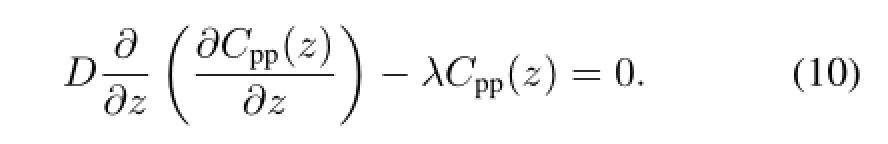
When z→ 0,Cpp(0)is the210Pbexconcentration in the interface soils between the plough and the plough pan layer, which represents the210Pbexconcentration at any depth of the ploughlayerorthe210Pbexconcentrationinthetopsoilsofthe plough pan layer.Based on this boundary condition,Eq.(10) can be solved as follows:
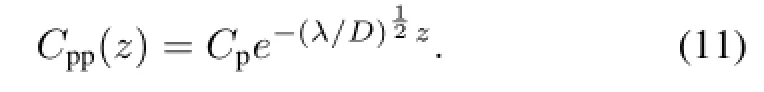
Integrating the two sides of Eq.(11)over the depth(z),the following can be derived:
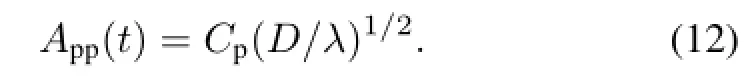
By combining Eqs.(5),(6),(11)and(12),the210Pbexconcentration in the soils of the plough layer in the steady state can be expressed as:
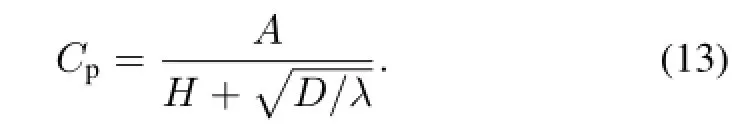
And the variation of the210Pbexconcentration with depth in the plough pan layer in the steady state can be expressed as:
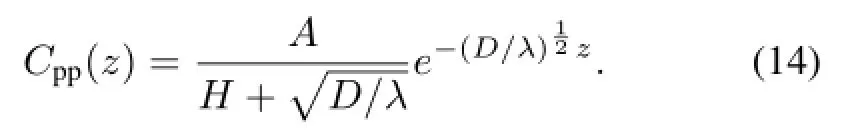
By combining Eqs.(9),(12)and(13),the time elapsed from initial cultivation to the steady state can be calculated as:
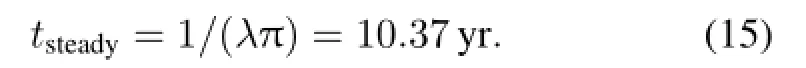
And the diffusion proportion in the steady state can be expressed as:
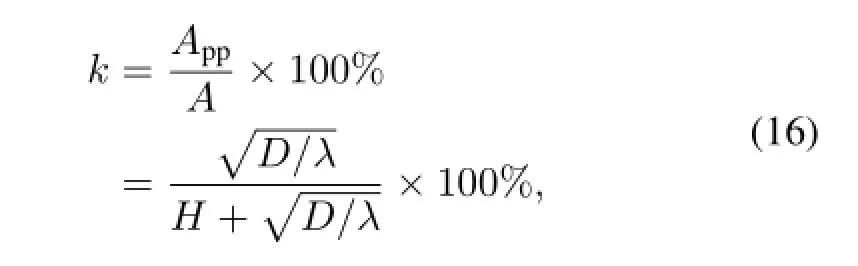
where k is the diffusion proportion in the steady state,which can be obtained by experiments.
From Eq.(16),the effective diffusion coefficient of210Pbexis:
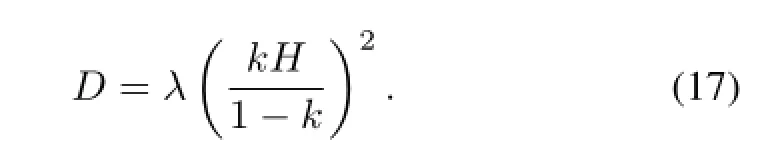
Equations(13)and(14)can be used to simulate the210Pbexdepth distribution in the steady state in the soil profile for the two layers of the cultivated land.A basic assumption of this theoretical derivation is that no erosion or deposition occurs. For an eroding soil,the210Pbexinventory would be invariable assuming the erosion rate is constant for over 100 years.Then the210Pbexdepth distribution will also be in steady state,and Eqs.(10)–(17)can be applicable as usual.
True,210Pbexdownward diffusion below the plough depth might be partially offset by soil erosion at the surface,causing the base of the plough layer to be displaced downwards, and some210Pbexthat has diffused downwards would return to the plough layer.However,the210Pbexdistributed below the plough depth,more or less,is a matter of fact.In the cultivated purple soil of the Sichuan Hilly Basin in China (Fig.1(d)),for example,ca.37%of the downwards diffused210Pbex,according to Eq.(8),returned to the plough layer at the first year of diffusion,assuming210Pbexis initially quite evenly distributed within the plough depth and the soil erosion rate is 0.5 cm/yr(the real erosion rates are usually less than this value[17]).In a word,210Pbexdiffusion in cultivated soils is a matter of fact in spite of the usual erosion.And the proportion would decrease with year and eventually tend to constant.Then,the210Pbexdistribution would achieve the steady state with a210Pbextail in the profile below the plough depth.
D.The simulated210Pbexdiffusion process in a soil core from the Yimeng mountains area
To know the210Pbexdepth distribution in cultivated soil in reality,a typical210Pbexprofile for a core from the cultivated cinnamon soils of the Yimeng mountains area in China was introduced in this study[27],based on which the210Pbexdiffusion process could be simulated for the core.The Yimeng Mountains area(ca.17180km2)is located in the central south of Shandong Province,China(34?22′to 36?13′N, 117?24′to 119?11′E),with elevations within the range of 150–1165m.The area belongs to the continental monsoon climate area of the warm temperate zone,and the cinnamon soils developed from limestone weathering crusts are wildly distributed.The core was collected from the fields situated in flat areas at the top of ploughed fields,where the disturbance except for cultivation over the past 100 years can be negligible.The profile(Fig.2(a))reflects the steady state of210Pbexdepth distribution because the soils have not been disturbed except for cultivation in nearly ca.100 years.From the profile and the field surveys it can be determined that,the plough depth(H)is ca.20cm because the210Pbexconcentrations in soils of the upper~20cm are relatively uniform,suggesting the210Pbexis mixed by tillage within the uppermost depth of~20cm,which is also in agreement with our field surveys;the210Pbexdiffusion proportion(k)is 32%,which is the ratio of the210Pbexinventory below the plough depth to the total inventory in the profile;the effective diffusion coefficient(D)is 2.72cm2/yr,which can be calculated using Eq.(17).
Equations(7)and(8)can simulate the210Pbexredistribution process in the plough and plough pan layer of cinnamon soils after the initial cultivation before the steady state(e.g. t=2,4,6,and 8 yr);Eqs.(13)and(14)can simulate the stable210Pbexdepth distribution in the two layers of the cultivated cinnamon soils(t≥10.37 yr)(Fig.2(b)).
III.MASS BALANCE MODEL IN CONSIDERATION OF THE DIFFUSION
A.Model derivation
Considering210Pbexdepth distribution in the cultivated land in view of the radionuclide diffusion from the plough to the plough pan layer,Eq.(1)can be rewritten as:
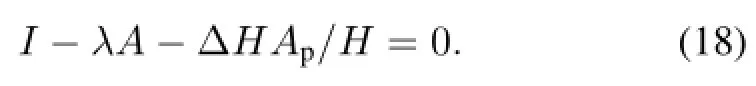
By combining Eqs.(2),(6),(13),(17)and(18),the erosion rate of the cultivated soil can be expressed as Eq.(19)based on the relationship between the210Pbexdeposition flux and the reference inventory(I=λAref).
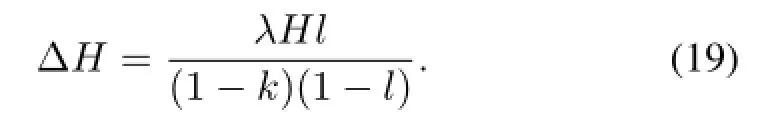
Therefore,considering the210Pbexdiffusion from the plough layer to the plough pan layer,the erosion rates can be estimated from Eq.(19).By ignoring the influence210Pbexdiffusion(k=0),Eq.(19)can be reduced to Eq.(3).
B. Comparison of the revision and existing models
As above,the difference between the revision and existing model lies in whether to take into account the amount of210Pbexdistributed below the plough depth.To compare the two models,let ΔH1and ΔH2be the erosion rates estimated using Eqs.(3)and(19),respectively,and we have:
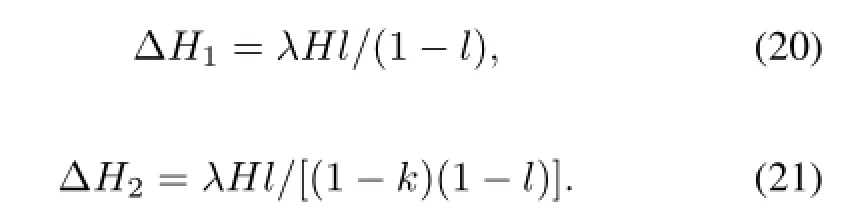
It is clear that ΔH1<ΔH2,and the difference ratio(d)of ΔH1and ΔH2is:
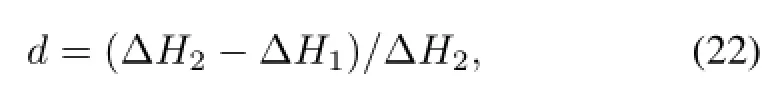
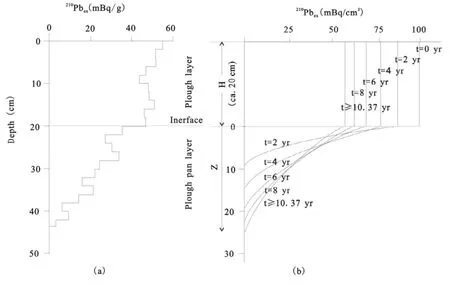
Fig.2.(a)The typical210Pbexprofile for a core from the cultivated cinnamon soils of the Yimeng mountains area[27];(b)The simulated 210Pbexredistribution process in the core after the initial cultivation.
By combining Eqs.(20)–(22),one has:

So,the difference ratio can be represented by the210Pbexdiffusion proportion in the steady state of the210Pbexdepth distribution,and the difference increases with the proportion of diffusion.
If the210Pbexdiffusion proportion(k)and loss proportion (l)are known,Eq.(19)can be used to estimate the erosion rates.These parameters can be derived from the experiments. Because the k is determined by the210Pbexprofile measurement,the accuracy of k is related to the accuracy of experiment,e.g.the study site selection,sampling method,the sampling section increments,laboratory measurement and etc.. Moreover,the recognition of the plough depth,according to the shape of the profile in conjunction with field survey,is also important to the accuracy of k.
IV.DISCUSSION AND CONCLUSION
As above,the erosion rates estimated using the revision model are higher than those estimated using the existing model by comparison.In other words,the existing model underestimates the erosion rate of the cultivated soils to some extent because of ignoring the amount of210Pbexdistributed below the plough depth.Nevertheless,the existing model involves the superiorities including compact form,fewer parameters,and convenience for application.In contrast,the revision model involves the limitations including complicated form and more parameters for application.Eq.(23)suggests that the underestimation of soil erosion from the existing model is as well less than 10%when the210Pbexdiffusion proportion(k)is lower than 10%,which could be negligible.In other words,the existing model is as usual applicable to soil erosion estimation when the diffusion proportion is lower than 10%.When the diffusion proportion ranges between 10%–15%,the existing model is also suitable if the underestimation is acceptable in some practical applications. However,the underestimation could not be neglected when the diffusion proportion is greater than 15%,and the revision model would be more applicable to erosion estimation than the existing model.No matter what the diffusion proportion is,the revision model is universal because the existing model is merely a case of the revision model(when k=0).In a word,the application scope of revision model is universal, especially for the locations where the diffusion proportions in the cores are greater than 15%.
Equation(17)indicates that the diffusion proportion is related to the diffusion coefficient(D)and the plough depth (H),viz.the diffusion proportion increases with the diffusion coefficient while decreases with the plough depth. The diffusion coefficients and the plough depths vary in different areas with different soil types and tillage systems.In the reported works,the diffusion proportions are usually less than 10%,e.g.a typical range of 5%–7%at the study sites in UK[16,19]and southern Italy[28,29].In these cases,the diffusion proportion can be negligible,and the existing model is applicable as before.For cultivated brown and cinnamon soils of the Yimeng mountains area in China,however,the diffusion proportions are greater than 15%[27],so the revi-sion model is more applicable to erosion estimation by comparison.
Neglecting the210Pbexbelow the plough depth,as above, will always underestimate soil erosion,nevertheless,it can also lead to an overestimation in some cases,e.g.,erosion of uncultivated soils might be overestimated when the reference site is selected in a cultivated soil if the210Pbexbelow the plough depth is neglected,viz.the sampling depth is restricted to the plough depth.
[1]Golosov V.Soil Till Res,2003,69:85–98.
[2]Walling D E,Collins A L,Sichingabula H M.Geomorphology, 2003,52:193–213.
[3]Zhang X B,Qi Y Q,He X B,et al.Catena,2006,68:1–9.
[4]Mabit L,Benmansour M,Walling D E.J Environ Radioactiv, 2008,99:1799–1807.
[5]Zapata F.Soil Till Res,2003,69:3–13.
[6]OndaY,Kato H,Tanaka Y,etal.JHydrol,2007,333,124–132.
[7]Wakiyama Y,Onda Y,Mizugaki S,et al.Geoderma,2010,159: 39–52.
[8]Gaspar L,Navas A,Mach′?n J,et al.Soil Till Res,2013,130: 81–90.
[9]Fang H Y,Sheng M L,Tang Z H,et al.Soil Till Res,2013, 133:85–92.
[10]Abbady A,Ahmed N K,El-arabi A M,et al.Nucl Sci Tech, 2006,17:118–122.
[11]Le R C and Wang X Q.Nucl Sci Tech,2006,17:92–96.
[12]Robbins R A.Geochemical and geophysical application of radioactive lead.In:Nriagu J O(Ed),The Biogeochemistry of Lead in the Environment.Amsterdam(Holland):Elsevier, 1978,286–383.
[13]Appleby P G and Oldfield F.Application of Lead-210 to sedimentation studies.In:Ivanovich M,Harma,R S(Eds), Uranium-Series Disequilibrium:Application to Earth,Marine and Environmental Sciences.Oxford(UK):Clarendon Press, 1992,731–738.
[14]Van Hoof P I and Andren A W.J Great Lakes Res,1989,15: 498–509.
[15]Nozaki Y,DeMaster D J,Lewis D M,et al.J Geophys Res, 1978,83:4047–4051.
[16]Walling D E and He Q.Soil Sci Soc Am J,1999,63:1404–1412.
[17]Zheng J J,He X B,Walling D E,et al.Pedosphere,2007,17: 273–283.
[18]Zhang X,Walling D E,Feng M.et al.Chinese Sci Bull,2003, 48:813–818.
[19]He Q and Walling D E.Appl Radiat Isotopes,1997,48:677–690.
[20]Schuller P,Ellies A,Kirchner G.Sci Total Environ,1997,193: 197–205.
[21]Szerbin P,Koblinger-Bokori E,Koblinger L,et al.Sci Total Environ,1999,227:215–227.
[22]Bossew P and Strebl F.J Environ Radioactiv,2001,53:199–213.
[23]Shinonaga T,Schimmack W,Gerzabek M H.J Environ Radioactiv,2005,79:93–106.
[24]Zhang X B,Long Y,He X B,et al.J Environ Radioactiv,2008, 99:1242–1246.
[25]Gank J.The Mathematics of Diffusion.Oxford(UK):Clarendon Press,1956,155–162.
[26]Shewmn P G.Diffusion in Solids.New York(USA):McGraw-Hill,1963,59–65.
[27]Zhang Y Q,Long Y,Yu X X,et al.Chin J Geochem,2014,33: 155–162.
[28]Porto P and Walling D E.J Environ Radioactiv,2012,106:47–57.
[29]Porto P and Walling D E.Appl Radiat Isotopes,2012,70: 2451–2459.
(Received November 27,2013;accepted in revised form February 21,2014;published online August 18,2014)
10.13538/j.1001-8042/nst.25.040303
?Supported by National Natural Science Foundation of China(Nos. 41102224 and 41101259)
?Corresponding author,yunqi768@163.com
 Nuclear Science and Techniques2014年4期
Nuclear Science and Techniques2014年4期
- Nuclear Science and Techniques的其它文章
- Simulation studies on two-frequency RF gun
- Tidal effects on hydrostatic leveling system used in high precision alignment of particle accelerator?
- External micro-PIXE analysis of Nd3+accumulation in Euglena gracilis?
- Mass thickness measurements for dual-component samples utilizing equivalent energy of X-rays?
- Synthesis of N,N′-dimethyl-N,N′-didecyl-3-oxa-diglycolamide for extraction of lanthanides?
- A K-CELL injection system for SH-PermEBIT?
