A new vortex sheet model for simulating aircraft wake vortex evolution
Mengda LIN,Guixiang CUI,Zhaoshun ZHANG
School of Aerospace,Tsinghua University,Beijing 100084,China
A new vortex sheet model for simulating aircraft wake vortex evolution
Mengda LIN,Guixiang CUI*,Zhaoshun ZHANG
School of Aerospace,Tsinghua University,Beijing 100084,China
A new vortex sheet model was proposed for simulating aircraft wake vortex evolution.Rather than beginning with a pair of counter-rotating cylindrical vortices as in the traditional models,a lift-drag method is used to initialize a vortex sheet so that the roll-up phase is taken into account.The results of this model report a better approximation to a real situation when compared to the measurement data.The roll-up induced structures are proved to influence the far-field decay.On one hand,they lead to an early decay in the diffusion phase.On the other hand,the growth of linear instability such as elliptical instability is suppressed,resulting in a slower decay in the rapid decay phase.This work provides a simple and practicable model for simulating wake vortex evolution,which combines the roll-up process and the far-field phase in simulation.It is also proved that the roll-up phase should not be ignored when simulating the far-field evolution of an aircraft wake vortex pair,which indicates the necessity of this new model.
?2017 Production and hosting by Elsevier Ltd.on behalf of Chinese Society of Aeronautics and Astronautics.This is an open access article under the CC BY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).
1.Introduction
The wakes of heavy aircraft pose danger to the following light planes,which calls for studies on the evolution of wake vortices.The lifetime of a wake vortex pair can be divided into the near-field evolution(or roll-up process),during which the vortex sheet shedding from the wing rolls up to a pair of counter-rotating vortices,and the far-field evolution,during which the vortices decay in the atmosphere.These two phases have been studied respectively by investigators using experimental or numerical methods.
For aviation safety,fast-time wake prediction models have been developed to predict the wake vortex evolution.1–3Large eddy simulation(LES)plays an important role in testing and calibration of these models.In most present LES,wake vortices are initialized with a vortex model such as the Lamb-Oseen model,Burnham-Hallock model,4or Proctor model,5with the roll-up process ignored.These simulations can be called non-roll up(NRU)simulations since the roll-up phase is skipped and only the far-field phase is simulated.However,the roll-up process does not end with a clean pair of vortices,but with turbulent structures as well.These structures may act in the following process and influence the far-field decay of the primary vortices.As this potential influence is ignored by NRU simulations,white noise is added to the initial vortices in some simulations6,7to deal with this conflict.A more direct approach is to combine the roll-up and far-field phases in a numerical simulation.However,the simulation of these two phases usually needs different numerical methods.In the simulation of the roll-up phase,Reynold averaged Navier–Stokes(RANS)8is appropriate and the mesh should distinguish the shape of an aircraft.In the far-field phase,LES is the most appropriate method.Misaka et al.9achieved this aim by coupling a high-fidelity RANS to LES.In Ref.9,the RANS result of flow field around a wing-body configuration and a high-lift configuration was inserted into the LES domain to initialize the wake,by which the influences of the roll-up process as well as the complex flow structures around aircraft components such as wing,fuselage,and flaps were taken into account.However,this method is far from practical application because of the large requirement of calculation amount.Therefore,a more practical numerical model is needed.
In this paper,a new lift-drag model is developed to simulate the whole lifetime of wake vortices from a vortex sheet to farfield decay.In this model,the action to the air by the aircraft is simplified to lift and drag in an elliptical distribution.This is a practicable engineering wake simulation model which takes the roll-up phase into account with the calculation amount similar to those of NRU models.The accuracy of this model is proved by a comparison to the measurement data.The results are also compared to those of NRU cases initialized with a cylindrical vortex pair to investigate the influence of the roll-up process to prove the inadequacy of NRU models.The conditions of moderate and strong atmospheric turbulences are both studied.This paper is organized as follows.The numerical methods as well as the initialization of the vortex sheet and ambient turbulence are introduced in Section 2.The result in the roll-up phase is discussed in Section 3,and the far-field evolution with analysis of the decay mechanism is described in detail in Section 4.Finally,in Section 5,the main conclusions are summarized.
2.Methodology
2.1.Numerical methods
The LES code self-Adaptive Tsinghua Turbulence lab Large Eddy Simulation (ATTLES)10solves the Boussinesqapproximated Navier-Stokes equations as follows:
whereuiis the velocity component in directioni(i=1,2,3)andxiis the coordinate.pis the pressure,tis the time,and ρ is the density.ν and νtare the molecular kinematic viscosity and sub-grid kinematic viscosity,respectively.ATTLES solves equations on a movable cartesian grid to deal with the requirement for high resolution in the vortex cores.The movement of the mesh is controlled by a self-adaptive algorithm which is developed from the spring analogy method by Gnoffo11and Nakahashi and Deiwert.12The details of the numerical method are described in Refs.10,13,14.
2.2.Vortex sheet initialization
The vortex sheet shedding from an elliptic wing is generated by imposing a body force in a flakiness region.As shown in Fig.1,withx,y,zbeing the fight direction(or axial direction of vortices),span-wise direction,and vertical direction,respectively,the flakiness region has a width equal to the wing spanB,and a thickness ofh<<B,while the length of this region is throughout the simulation domain in the flight direction.A periodic boundary condition is applied inx-direction to simulate the temporal evolution of the wake vortex,equivalent to a vortex sheet with an infinite length in the flight direction.The distribution of the body force is set to
where τ is the duration of the body force and Γ0is the maximum circulation.In this paper,the thickness of the vortex sheeth=0.677 m is employed.The duration of the body force τ should be as short as possible to ensure accuracy,while too short a τ,or too large anfz,would lead to numerical instability.We employ τ=0.1 s after balance.Considering the axial vorticity transport equation,
where ω is the vorticity vector.As τ is small,the third term on the right of Eq.(4)is much larger than the other two.Thus the intensity of the vortex sheet γ can be calculated as
which is exactly the intensity distribution of a vortex sheet from an elliptic wing.The physical meaning offzis the reactive force acting on the air by the wing.Therefore,fzis numerically equal to the local lift,which is in an elliptical distribution.In this paper,an approaching A340 aircraft is considered with a wing spanB=60.3 m,lift coefficientCL=1.40,aspect ratio λ=9.5,and approaching speedVA=75 m/s,corresponding to the wake parameters Γ0=424 m2/s,initial vortex separationb0=πB/4=47.4 m,initial vortex descending speedw0= Γ0/(2πb0)=1.42 m/s,and characteristic time scalet0=w0/b0=33.3 s using an elliptically loaded wing assumption.
Moreover,to take the influence of the flight drag into account,a body force in the flight directionfxis also added into the model.Similar tofz,fxis numerically equal to the local drag.Although the span-wise distribution of drag is complex for a real aircraft with a high lift configuration such as flaps and slats,it is simplified to an elliptical distribution as
whereL/Dis the lift to drag ratio,which is set to 12 according to the RANS of a high lift configuration by Keye.8This model is called lift-drag model because the action to the air by the aircraft is simplified tofzandfxin elliptical distributions.
2.3.Ambient turbulence initialization
The initialization of the ambient turbulence is similar to Lin et al.’s work.10An isotropic turbulence field with a given eddy dissipation ε is obtained with Rogallo’s method.15The turbulent velocity in spectral space is
in which θ1,θ2,and φ are the uniform random numbers in[0,2π].E(k)is the prescribed kinetic energy spectrum,which is a modified Von Karman spectrum16as
kp=(1/25)m-1is employed in this paper corresponding to a turbulence integral length of 25 m.kkolis the Kolmogorov wave number defined by(ε/ν3)1/4,andK0is determined from the following relation indirectly:
The turbulence field is at first produced on a uniform mesh of 256,3and then interpolated onto the computational grid.The details are described in Ref.10.
2.4.Set-up of simulation cases
In this paper,the temporal evolution of a wake vortex is simulated in two cases named roll-up 1(RU1)and roll-up 2(RU2)with two different ambient turbulences,ε=10-4m2/s3and ε=10-3m2/s3,corresponding to non-dimensional turbulence dissipation rates ε*=(εb0)1/3/w0=0.12 and 0.25.The simulation domain size isLx×Ly×Lz=8×5×with the grid number 480×320×200.The axial(x)length of the domain is set to be 8b0,the scale of Crow’s long wave instability.17In the axial direction,a uniform mesh space of⊿x=0.0167 is employed.In the other two directions,the span-wise direction(y)and the vertical direction(z),an adaptive grid method described in Ref.10is applied,with the initial core resolution being ⊿core=⊿y=⊿z=0.003b0.Periodic boundary conditions are applied to all three directions and a Lagrangian dynamic Smagorinsky model18is used.First of all,an ambient turbulence is initialized in the domain.Afterwards,a vortex sheet is generated(shown in Fig.2)and rolls up to form a pair of counter-rotating vortices,and then undergoes far-field decay.A fixed time step of Δt=t0/10000 is used throughout the computation.
Two cases,non-roll-up 1(NRU1)and non-roll-up 2(NRU2),are simulated in order to investigate the influence of the roll-up process to far-field decay.These two cases have the same settings as those of cases RU1 and RU2,respectively,except for the initialization of vortices.In NRU1 and NRU2,a pair of cylindrical vortices is overlaid to the ambient turbulence as
wherevtan(r)andu(r)are the tangential and axial components at radiusr,respectively,and the subscript‘roll_up’means the result from the roll-up cases.The initial vortex positions are also the same as those in the roll-up cases.These cases are listed in Table 1.
3.Roll-up process
In the early time of the simulation,the vortex sheet rolls up to form a pair of wake vortices.This phase can be regarded as the roll-up process.Fig.3 shows the roll-up process with thecontour of an absolute value of normalized axial vorticity= ωxt0in case RU1(ε*=0.12).At the dimensionless timet*=0.006,the vortex sheet begins to roll up at the wing tips(Fig.3(a)).At the timet*=0.4,the wake vortices take shape with a separation ofb0approximately,which is in agreement with the elliptical wing theory.
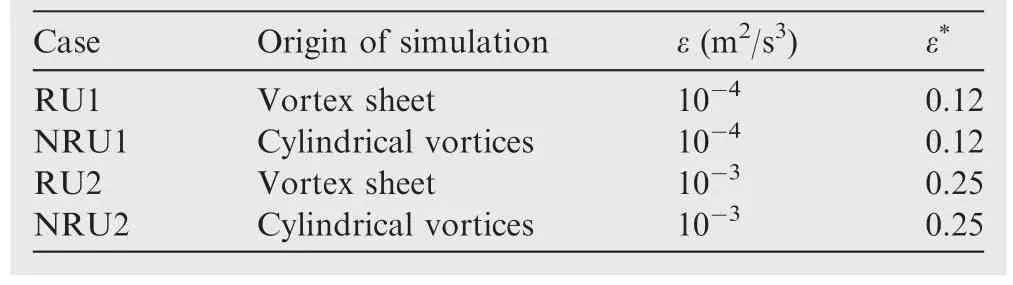
Table 1 List of cases.
In a real case,a multi-vortex system is obtained including the wing tip vortices,flap vortices,and nacelle vortices.The flap-tip vortex dominates and the other vortices blend into it or turn into turbulence surrounding at last.9The tangential velocity profiles near the center of outer flap vortex(OFV)with a wind tunnel experiment19are shown in Fig.4.The profiles near the center of the vortex of the current LES are also shown,and the distance from the aircraft is transformed to the time witht=x/VA=x/uinf,whereuinf=VA.It can be seen that the LES results agree with the experiment ones on the whole,and the results of cases RU1 and RU2 are close to each other.The simulated results appear to be smoother than the measured ones,which is a result of the exclusion of the complex configuration of the wing.
As the ultimate purpose of the lift-drag model is to predict the far-field evolution,the performance of the model should be evaluated by the final generated vortex.The evolution of vortex parameters is shown in Fig.5.The circulation is calculated with
The 5–15 m averaged circulation is defined as and Γb0/2is defined as Γb0/2= Γ(b0/2).Fig.5(a)shows the evolutions ofis equal to 1.0 at the very beginning and slightly decays to approximately 0.96 att*=0.3,whileis valued 0.78 att*=0 and slightly increases as the vorticity is rolled into the vortex core.The difference between RU1 and RU2 is small,which indicates that the ambient turbulence hardly affects the circulation during roll-up.The separation of two vortices decreases from the wing spanBto vortices separationb*=0.95(Fig.5(b)),which is approximately in agreement with the elliptical wing theory(b*=1.0).Fig.5(c)shows the dimensionless core radiusdefined as the radius at the peak of the tangent velocity.The core radius is approximately 2%ofb0,which is smaller than Misaka’s result,9which is due to the finer mesh in the vortex core compared to Misaka’s simulation.Misaka compared results of cases with different mesh spaces and indicated that a finer mesh size would result in a smaller core size.
The influence of the drag in Eq.(7)can be described with the evolution of the axial velocityu*,which is displayed in Fig.6.Att*=0.05,the axial velocity is produced by the flight drag in the vortex sheet.Att*=0.5,the axial movement decays except for a peak in the core regions.The peak values in the vortex center are 4.13 and 4.04 for the left and right vortices,respectively,which are similar to Misaka’s simulation result9withu*=4.0.Although in the current work the merging process of the multi-vortex system is not taken into account,the final axial velocity is similar.
The axial velocity surrounding the vortex core turns into secondary structures.Fig.7 displays the iso-surface of λ2=-0.3.20At the beginning of roll-up(t*=0.08),a large amount of small-scale vortices take shape in the vortex sheet as a result of the combined action of the flight drag and the vortex sheet.As the rolling-up proceeds,these vortices are rolled up and evolve into rib-like vortices surrounding the primary vortex.To quantify the strength of these secondary structures,the tangential component of entropy is calculated withwhere ‘〈〉’stands for taking average between radius from 10 m to 20 m and then further averaged along the vortex center line.The evolution ofis shown in Fig.8.To show the relationship ofto the drag force,an additional case RU1B with the same condition as that of case RU1 except for a smaller drag(L/D=20)is displayed in Fig.8.in case RU1B is smaller than that in RU1,which indicates that the non-axial vorticities are mainly induced by the flight drag.This can be explained by the axial velocity.The sheer of the axial velocity(Fig.6(a))produces span-wise vorticities,which are rolled into the wake vortex and develop into tangential vorticities.At firstis strong due to the axial velocity sheer(Fig.6).The tangential vorticities decay as the axial velocity turning into turbulence untilt*=0.15 and then maintaining its value.These rib-like structures are believed to play an important role in future vortex decay by Holza¨pfel6and Misaka et al.21.In their work,these secondary structures come from environmental turbulence and baroclinic vorticity,while the current study indicates that the flight drag could be another source of the secondary vorticity.These structures could accelerate the vortex decay from the very beginning,which will be discussed in the following section.
The radial distributions of the axial-averaged tangent velocityand the axial velocityu*of the left vortex are illustrated in Fig.9.It can be seen that the radial velocity profile is similar for cases RU1 and RU2 except that the peak ofu*in case RU1 is slightly higher than that in case RU2,indicating that the strength of ambient turbulence does not influence the final vortex obviously.This velocity profile is used as the initial condition of the non-rollup cases NRU1 and NRU2.The Burnham-Hallock(BH)model4with circulation Γ =0.95Γ0and the vortex core radiusrc=0.03b0is also displayed.The difference between the current result and that of the BH model may be due to the elliptical wing assumption,which has certain discrepancy with a modern aircraft.
4.Far-field evolution
4.1.Evolution of vortex parameters
After the roll-up process,the vortices undergo their far-field decay.Fig.10 shows the evolution of vortex parameters in the far-field decay.In Fig.10(a),the decay of a 5–15 m average circulation normalized by its initial value Γ5-15,0=350 m2/s is illustrated.The circulation is further averaged along the vortex centerline,and its axial maximum and minimum values are also shown by the dashed line in Fig.10(a).The mean of a series of Lidar measurement under ε=(0.5–2.0)× 10-4m2/s3in Tarbes,France22is also shown in Fig.10(a).For case RU1,the two-phase decay,1the diffusion phase(DP)and the rapid decay phase(RDP),can be observed and the onset time of the RDP is approximatelyt*=3.The decay rate in the DP and the onset time of the RDP are in agreement with the Lidar measurement,while the decay rate of the RDP appears slightly higher.This agreement supports the rationality of the current model.For case NRU1(Fig.10(a),solid line with symbols),the circulation hardly decays in the DP,and it turns into the RDP att*=3.0,which is similar to those in RU1 and the Lidar measurement.The decay rate of the RDP in NRU1 appears greater than those in both RU1 and the measurement,which indicates that the instability in NRU1 develops faster.The analysis of this phenomenon will be presented in Section 4.2.The result of case RU1B with a smaller flight drag is also shown in Fig.10(a)as the thin black solid line.The result in RU1B is similar to that in case NRU1 except for a slightly earlier RDP.This indicates that the NRU model is appropriate in the wake simulation of low-drag conditions such as a cruising aircraft.This viewpoint is also supported by Misaka et al.’s LES study.9However,the divergence with the Lidar measurement indicates that the NRU model is less accurate in the simulation of landing aircrafts with a larger drag.This result also reveals that the far-field evolution is sensitive to the flight drag,which supports the necessity of bringing in the drag influence to this model by Eq.(7).
In cases RU2 and NRU2 with a stronger ambient turbulence(Fig.10(b)),the onset time of the RDP for measurement(ε=(0.5–2.0)× 10-3m2/s3)in both RU2 and NRU2 is approximatelyt*=2.Both simulation cases report a lower decay rate in the DP and a higher decay rate in the RDP compared to the measurement,but their onset time of the RDP is in agreement.The difference between RU2 and NRU2 lies in the decay rate in the DP,during which RU2 decays faster due to the tangential vorticity generated in the roll-up phase.Besides,the decay rate in the RDP appears similar for both cases.The influence of the roll-up process appears less important in stronger ambient turbulence cases,for the ambient turbulence becomes the emphasis in the initial perturbation.
Fig.10(c)shows the vortex descending under the mutual induction.For all cases,the initial descending rate is approximately 1.After that,the descending slows down as the circulation decays.Vortices descend slower in roll-up cases(RU1 and RU2)as a result of a smaller circulation.Besides,the increase of vortex separation shown in Fig.10(d)could be another reason of this deceleration,for the mutual induction between two vortices is weakened.For case RU1B,the descending and vortex separation show no obvious difference from cases RU1 and NRU1.
Fig.11 shows the growth of the core radius.The core expands from then beginning 0.02b0to approximately 0.04b0during the DP.For the lack of turbulence in the solid rotating core,the diffusion of core vorticity is very slow as the molecular viscous is negligible.Therefore,the expanding in the current case is caused by numerical diffusion.According to Misaka9and Hennemann et al.,7this expanding hardly influences the vortex parameters such as 5–15 m average circulation and descending if controlled in a small range.It can be seen in Fig.11 that the core radii in all cases are no more than 0.05b0,which is smaller than Misaka’s result9because a smaller core mesh space is used in this paper.The core radius decrease happens at the onset time of the RDP.The circulation at the core radius Γcis shown in Fig.12.Except for a short increase att*<1,is a constant of approximately 0.4 in the DP although the core radius increases under the numerical diffusion.The decay ofmeets with the rapid decay of(Figs.10(a)and(b))and the decrease of the core radius(Fig.11),which indicates that the rapid decay begins with the destruction of the vortex core.
Fig.12 also shows the circulation decay at different radii.For the roll-up cases RU1 and RU2,the circulation at larger radii(10 m,15 m,25 m)begins to decay immediately while the 5 m circulation stays constant until the rapid decay,which is also observed by Hallock and Burnham’s Lidar measurement.23This indicates that the vorticity at larger radii decays earlier.For the non-roll up cases NRU1 and NRU2,the circulation decay at larger radii appears to stay constant at the beginning due to the lack of roll-up turbulence shown in Fig.7(c).The analysis on the evolution of vortex parameters indicates that the new model reports a better approximation to the real situation compared to the NRU models.
4.2.Analysis of decay mechanism
In the analysis of vortex parameters,the RU cases show better agreements with the real case measurements.The NRU cases report a different way in circulation decay especially in weaker ambient turbulence cases.In NRU1,the circulation tends to stay as a constant in the DP and undergoes a sudden crash in the RDP(Fig.10(a)),while in RU1,the circulation undergoes a slow decay immediately after the roll-up,and the decay rate in the RDP is moderate.
The decay mechanism of RU1 and NRU1 can be explained by the growth of instability.Fig.13 displays the iso-surface of λ2=-1 in case NRU1.Att*=3.0,the onset time of the RDP,tangential vorticity around the primary vortices can be seen,leading to a local decay of circulation in the corresponding region(x*=2–4,t*=3.0,Fig.14).This decay may be a result of the growth of elliptical instability.24Fig.15 shows a closer photograph of the local decay region att*=3.0,in which elliptical instability can be observed by the iso-surface of λ2=-40 with a wave length of λe≈ 0.17b0,which is approximately 4.25 times the core radius and agrees with Leweke’s measurement.24Crow’s instability17can be recognized aftert*=4.0,but the vortices have failed to link because their strengths have been greatly weakened by the development of elliptical instability.This indicates that elliptical instability dominates the decay rather than Crow’instability in the current case.This phenomenon can be proved reasonable with an estimation of the strength of initial perturbation on the wave lengths of two instability modes.As the isotropic turbulence model is used in this paper,elliptical instability will dominate under this condition according to Laporte and Corjon.25To inspect the growths of both long wave and elliptical instabilities,the axial average kinetic energy spectrumEa(λe)is calculated with the same method used by Laporte and Corjon25as follows:
Eq.(18)means that the spectrum is averaged in a cylindrical area with a radius ofR=10 m in this paper.The initialof case NRU1(t*=0.5)is shown in Fig.16 with the dashed line,which is equal to the initial ambient turbulence.The temporal growth ofis shown in Fig.17.In case NRU1(the dashed line),an obvious linear growth in the elliptical mode can be observed with a growth rate of σ=0.89,which is slightly smaller than Leweke and Williamson’s measurement,24i.e.,σ=0.94±0.12.
The initialEλ*of case RU1(t*=0.5)is also shown in Fig.16 with the solid line.The energy spectrum in case RU1 is much stronger than that in case NRU1 by approximately 10 times,which is not surprising because of the roll-up process.The spectrum can be observed departing from the 5/3 law by an increase near λ*=0.1,which may be a result of the roll-up induced structures shown in Fig.7(c).The growth of elliptical instability energyin case RU1 is shown in Fig.17 by the solid line,in which a growth in the elliptical mode can be observed,but the linear growth is not obvious.The energy turns to decrease right aftert*=3.0,the rapid decay time.Although the initial value ofis higher in RU1 than that in NRU1,the peak of RU1 is lower.It indicates that the roll-up induced structures suppress the growth of linear instability with some non-linear mechanism,and this can explain the smaller RDP decay rate in RU1 than that in NRU1.Fig.18 shows the evolution ofiso-surface of λ2=-1 in case RU1.The tangential vorticity(t*=1.0)generated in the roll-up process acts on the primary vortices from the very beginning,leading to a decay in the diffusion phase(Fig.10(a)).As elliptical instability grows into saturation,Crow’s long wave instability can be distinguished aftert*=4.025.On the contrary,in case NRU1,the vortices maintain some of their strengths so that Crow’s instability is able to develop obviously.The two vortices are about to link att*=8.0(last picture in Fig.18).
In the cases with a stronger ambient turbulence,the difference in decay mechanism between RU2 and NRU2 is relatively smaller.The evolution ofiso-surface of λ2is shown in Fig.19.It can be seen that the roll-up induced structures contribute to the early decay in the DP in case RU2.At the onset time of RDP(t*=2.0),large amount of secondary vorticity can be observed in both RU2 and NRU2.Although Crow’s long wave instability can be seen att*=4.0,the vortices have no chance to link because the vortices have lost most of their strengths by then.
5.Conclusions
Large Eddy simulation of the evolution of an aircraft wake vortex from roll-up to far-field decay was conducted with a new lift-drag model.A transience downward force is imposed to a slice-shaped region to generate a vortex sheet,and the influence of the flight drag is also simulated by an axial body force.A lift-to-drag ratio of 12.0 is employed to simulate a landing aircraft.
Thelift-drag vortex generation modelsimplifiesthe rounded flow structure surrounding an aircraft into two parts:a vortex sheet from the trailing edge of the wing and an axial movement induced by the flight drag,which are approached with the lift forcefzand the drag forcefx,respectively.The far-field simulation results show that this approach is reasonable.The results of this model are compared to those of the traditional counter-rotating cylindrical vortices model(NRU cases).The new model appears to report a better approximate to a real situation,which is supported by the following facts.The result of the new model reports a better agreement with the Lidar measurement.In the roll-up cases,the circulation at larger radii begins to decay immediately after roll-up,which is confirmed by Burnham’s measurement.23Besides,a more moderate RDP decay rate is reported by the roll-up case compared to the non-roll-up case in a weak ambient turbulence condition,which is also supported by the measurement.22
Furthermore,how the roll-up process influences the decay mechanism is discussed.In the roll-up process,the ambient turbulence hardly influences the resulting vortices in circulation,separation,and core radius.The axial velocity is induced by the flight drag and rolled into the core resulting in a peak in the vortex center.The tangential vorticity is also induced by the flight drag,and then translated into the secondary vortices surrounding the primary vortex.These roll-up induced structures play an important role in far-field decay.On one hand,they lead to a decay in the DP,by decreasing the circulation in larger radii.On the other hand,in the case with weaker ambient turbulence,the growth of linear instability such as elliptical instability is suppressed by the roll-up induced structures,resulting in a smaller decay rate in the RDP.Besides,the roll-up process brings less difference in case of strong ambient turbulence,for the ambient turbulence becomes the emphasis.
This paper proves that the roll-up process should not be ignored in the numerical simulation of a landing aircraft especially in weak atmospheric turbulence.This work provides a simple and practicable engineering model to combine the roll-up process and the far-field phase.Nevertheless,there is potential improvement for this model.The distribution of the lift and drag can be modified rather than simply taking an elliptically loaded wing assumption for a better approach to a real aircraft.Besides,the influence of the lift-to-drag ratio also needs further investigation.These problems are currently studied.
Acknowledgement
The work was supported by the Boeing-COMAC Aviation Energy Conservation and Emissions Reduction Technology Center(AECER).
1.Holza¨pfel F.Probabilistic two-phase wake vortex decay and transport model.J Aircraft2003;40(2):323–31.
2.Proctor FH,Hamilton DW,Switzer GF.TASS driven algorithms for wake prediction.Proceedings of 44th AIAA aerospace sciences meeting and exhibit;2006 Jan 09–12;Reno(NV),USA.Reston(VA):AIAA;2006.
3.Ahmad NN,VanValkenburg RL,Pruis M.NASA AVOSS Fast-Time Wake prediction models:user’s guide.Washington,D.C.:NASA.Report No.:NASA/TM-2014-218152;2014.
4.Burnham DC,Hallock JN.Chicago monostatic acoustic vortex sensing system.Volume IV.Wake vortex decay.Report No.:DOT/FAA/RD-79-103 IV;1982.
5.Shen SH,Ding F,Han J,Lin YL,Arya SP,Proctor FH.Numerical modeling studies of wake vortices:real case simulations.Proceedings of 37th aerospace sciences meetingamp;exhibit;1999 Jan 11–14;Reno(NV),USA.Reston(VA):AIAA;1999.
6.Holza¨pfel F,Gerz T,Baumann R.The turbulent decay of trailing vortex pairs in stably stratified environments.Aerospace Sci Technol2001;5(2):95–108.
7.Hennemann I,Holza¨pfel F.Large-eddy simulation of aircraft wake vortex deformation and topology.Proc Inst Mech Eng,Part G:J Aerospace Eng2011;225(12):1336–50.
8.Keye S.Fluid-structure coupled analysis of a transport aircraft and flight-test validation.J Aircraft2011;48(2):381–90.
9.Misaka T,Holza¨pfel F,Gerz T.Large-eddy simulation of aircraft wake evolution from roll-up until vortex decay.AIAA J2015;53(9):2646–70.
10.Lin MD,Cui GX,Zhang ZS.Large eddy simulation of aircraft wake vortex with self-adaptive grid method.Appl Math Mech2016;37(10):1289–304.
11.Gnoffo PA.A finite-volume,adaptive grid algorithm applied to planetary entry flowfields.AIAA J1983;21(9):1249–54.
12.Nakahashi K,Deiwert GS.Self-adaptive-grid method with application to airfoil flow.AIAA J1987;25(4):513–20.
13.Shi RF,Cui GX,Wang ZS,Xu CX,Zhang ZS.Large eddy simulation of wind field and plume dispersion in building array.Atmos Environ2008;42(6):1083–97.
14.Liu YS,Cui GX,Wang ZS,Zhang ZS.Large eddy simulation of wind field and pollutant dispersion in downtown Macao.Atmos Environ2011;45(17):2849–59.
15.Rogallo RS.Numerical experiments in homogeneous turbulence.Washington,D.C.:NASA.Report No.:NASA-TM-81315;1981.
16.Bechara W,Bailly C,Lafon P,Candel SM.Stochastic approach to noise modeling for free turbulent flows.AIAAJ1994;32(3):455–63.
17.Crow SC.Stability theory for a pair of trailing vortices.AIAA J1970;8(12):2172–9.
18.Meneveau C,Lund TS,Cabot WH.A Lagrangian dynamic subgrid-scale model of turbulence.J Fluid Mech1996;319:353–85.
19.Ko¨nig J,Hansen H,Coustols E,Dobrzyinski W.New technologies in low speed aerodynamics—wind tunnel and flight tests demonstrated in AWIATOR.In:Neittaanma¨ki P,Rossi T,MajavaK,PironneauO,EstolaKP,BonaGD,editors.ECCOMAS 2004-European congress on computational methods in applied sciences and engineering;2004.
20.Jeong JH,Hussain F.On the identification of a vortex.J Fluid Mech1995;285:69–94.
21.Misaka T,Holza¨pfel F,Hennemann I,Gerz T,Manhart M,Schwertfirm F.Vortex bursting and tracer transport of a counterrotating vortex pair.Phys Fluids2012;24(2),025104-1-21.
22.Ko¨pp F,Rahm S,Smakikho I,DolfiA,Cariou JP,Harris M,et al.Comparison of wake-vortex parameters measured by pulsed and continuous-wave lidars.J Aircraft2005;42(4):916–23.
23.Hallock JN,Burnham DC.Decay characteristics of wake vortices from jet transport aircraft.Reston(VA):AIAA.Report No.:AIAA-1997-0060;1997.
24.Leweke T,Williamson CHK.Cooperative elliptic instability of a vortex pair.J Fluid Mech1998;360(1):85–119.
25.Laporte F,Corjon A.Direct numerical simulations of the elliptic instability of a vortex pair.Phys Fluids2000;12(5):1016–31.
31 May 2016;revised 22 September 2016;accepted 3 November 2016
Available online 8 June 2017
*Corresponding author.
E-mail address:cgx@tsinghua.edu.cn(G.CUI).
Peer review under responsibility of Editorial Committee of CJA.
Production and hosting by Elsevier
http://dx.doi.org/10.1016/j.cja.2017.04.015
1000-9361?2017 Production and hosting by Elsevier Ltd.on behalf of Chinese Society of Aeronautics and Astronautics.
This is an open access article under the CC BY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).
Aircraft;
Aerodynamics;
Large eddy simulation;
Vortex sheet;
Wake vortex far field decay
 CHINESE JOURNAL OF AERONAUTICS2017年4期
CHINESE JOURNAL OF AERONAUTICS2017年4期
- CHINESE JOURNAL OF AERONAUTICS的其它文章
- Guide for Authors
- Simulation and experimental investigation on a dynamic lateral flow mode in trepanning electrochemical machining
- Investigation of high-speed abrasion behavior of an abradable seal rubber in aero-engine fan application
- Dynamic performance of a C/C composite finger seal in a tilting mode
- A unifying approach in simulating the shot peening process using a 3D random representative volume finite element model
- Approach and landing guidance design for reusable launch vehicle using multiple sliding surfaces technique
