SPECTRA OF COMPOSITION GROUPS ON THE WEIGHTED DIRICHLET SPACE OF THE UPPER HALF-PLANE?
M.O.AGWANG J.O.BONYO
Department of Pure and Applied Mathematics,Maseno University,
P.O.BOX 333-40105,Maseno,Kenya
E-mail:omeshackagwang@yahoo.com;jobbonyo@maseno.ac.ke
Abstract We prove that the group of weighted composition operators induced by continuous automorphism groups of the upper half plane U is strongly continuous on the weighted Dirichlet space of U,Dα(U).Further,we investigate when they are isometries on Dα(U).In each case,we determine the semigroup properties while in the case that the induced composition group is an isometry,we apply similarity theory to determine the spectral properties of the group.
Key words one-parameter semigroup;composition operator semigroups;strong continuity;infinitesimal generator;spectra
1 Introduction
For an open subset?of C,let H(?)denotes the Fr′echet space of analytic functions on?endowed with the topology of uniform convergence on compact subsets of?.In this note,?can be either the open unit disk D or the upper half plane U.If?is a self analytic map on?,then the induced composition operatorC?acting on H(?)is defined byC?f=f??,with the corresponding weighted composition operator on H(?)given byS?=(?′)γC?for some appropriately chosen weightγ.
Composition operators on spaces of analytic functions on the unit disc H(D)have been extensively studied in the literature comparatively to their counterparts on the analytic spaces of the upper half plane H(U).Even though there are isomorphisms between the corresponding spaces of D and of U,composition operators act differently in the two cases.For instance,unlike the case of Hardy or Bergman spaces of D,not every composition operator is bounded on Hardy or Bergman spaces of U,see[1,2].It has also been proved in[3,4]that there are no non-trivial(i.e.with symbol not constant)compact composition operators on the Hardy spaceH2(U)or the weighted Bergman spacewhich is not the case forH2(D)or.
Unlike the Hardy and Bergman spaces of D cases,composition operators on the(weighted)Dirichlet space of the unit disk Dα(D)are not necessarily bounded;an indication that the action of composition operators on the weighted Dirichlet space of the upper half plane Dα(U)is very much complicated.Recent attempts to study composition operators on Dα(U)can be found in[5,6].Schroderus[5]obtained the spectrum of composition operators induced by linear fractional transformations(LFTs)of the upper half plane U;while Sharma,Sharma and Raj[6]characterized boundedness and compactness of composition operators on D0(U).In particular,it is proved in[6]that every LFT of U induces a bounded composition operator on D0(U).This therefore implies that continuous groups(?t)t∈Rof automorphisms of the upper half plane may induce a bounded group of(weighted)composition operatorsTt:=S?ton Dα(U).It is important to note that the study of composition semigroups on the Dirichlet spaces has scantly been considered with the only reference[7]being on the Dirichlet space of the unit disk.
In this paper,we extend the study carried out by the second author with his co-authors in[8]on Hardy and weighted Bergman spaces of U to the setting of the weighted Dirichlet space of U.In particular,we prove that the group(Tt)t∈Rof weighted composition operators is strongly continuous on Dα(U).Using the classification theorem of continuous groups of automorphisms of U[8,Proposition 2.3],we consider the corresponding weighted composition operator groups(Tt)t∈Ron Dα(U)and investigate when they are isometries.It turns out that the scaling and translation groups do not induce weighted composition operator groups on Dα(U)which are isometries as is the case for Hardy and Bergman spaces of U[8].The infinitesimal generators of the composition groups are calculated and their properties discussed.For the rotation automorphism group,(Tt)t∈Rturns out to be a group of isometries on Dα(D).In this case therefore,we determine both its semigroup and spectral properties.
2 Preliminaries and Definitions
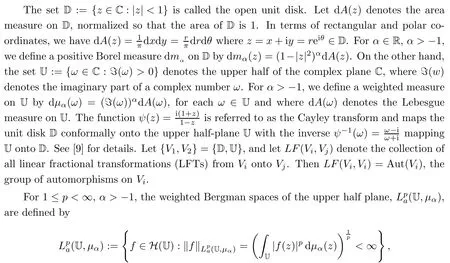

We refer to[15–17]for details on the theory of semigroups.
IfXandYare arbitrary Banach spaces,we denote the Banach space of bounded linear operators fromXtoYby L(X,Y).We shall write L(X)instead of L(X,X).LetTbe a closed operator onX.The resolvent setρ(T)ofTis given byρ(T)={λ∈C:λI?Tis invertible}and its spectrum isσ(T)=Cρ(T).The spectral radius ofTis defined byr(T)=sup{|λ|:λ∈σ(T)}and satisfies the relationr(T)≤‖T‖.The point spectrumσp(T)={λ∈C:T x=λxfor some 0≠x∈dom(T)}.Forλ∈ρ(T),the operatorR(λ,T):=(λI?T)?1is by the closed graph theorem a bounded operator onXand is called the resolvent ofTat the pointλor simply the resolvent operator.See[15,16,18]for details.
IfXandYare arbitrary Banach spaces andU∈L(X,Y)is an invertible operator,then clearly(At)t∈R?L(X)is a strongly continuous group if and only ifBt:=UAtU?1,t∈R,is a strongly continuous group in L(Y).In this case,if(At)t∈Rhas a generatorΓthen(Bt)t∈Rhas generator?=UΓU?1with domain

Moreover,σp(?,Y)=σp(Γ,X)andσ(?,Y)=σ(Γ,X).Ifλis in the resolvent setρ(Γ,X):=Cσ(Γ,X),we have thatR(λ,?)=UR(λ,Γ)U?1.For more details on the theory of similar semigroups,we refer to[15–17].
All self analytic maps of U were identified and classified in[8]into three distinct groups according to the location of their fixed points,namely;scaling,translation and rotation groups.Specifically we give the following classification theorem:
Theorem 2.1(see[8,Proposition 2.3])Let?:R→Aut(U)be a nontrivial continuous group homomorphism.Then exactly one of the following cases holds:
1.There existsk>0,k≠1,andg∈Aut(U)so that?t(z)=g?1(ktg(z))for allz∈U andt∈R.
2.There existsk∈R,k≠0,andg∈Aut(U)so that?t(z)=g?1(g(z)+kt)for allz∈U andt∈R.
3.There existsk∈R,k≠0,and a conformal mapping g of U onto D such that?t(z)=g?1(eiktg(z))for allz∈U andt∈R.Equivalently,there existθ∈R 高碑店市| 望奎县| 碌曲县| 江城| 忻城县| 西平县| 徐水县| 孟州市| 石嘴山市| 阳新县| 牟定县| 阿拉善盟| 江孜县| 灵石县| 福鼎市| 什邡市| 揭西县| 隆安县| 罗田县| 通山县| 界首市| 浑源县| 平凉市| 临澧县| 胶州市| 房山区| 长沙市| 扬州市| 汉寿县| 西宁市| 辉县市| 北辰区| 依兰县| 双峰县| 专栏| 厦门市| 那坡县| 蒙自县| 遵义市| 凯里市| 开阳县|
