Angular engineering strategy of an additional periodic phase for widely tunable phase-matched deep-ultraviolet second harmonic generation
Mingchuan Shao,Fei Liang,Haohai Yu?and Huaijin Zhang?
1State Key Laboratory of Crystal Materials and Institute of Crystal Materials,Shandong University,Jinan 250100,China
Abstract Manipulation of the light phase lies at the heart of the investigation of light-matter interactions,especially for efficient nonlinear optical processes.Here,we originally propose the angular engineering strategy of the additional periodic phase(APP)for realization of tunable phase matching and experimentally demonstrate the widely tunable phasematched second harmonic generation(SHG)which is expected for dozens of years.With an APP quartz crystal,the phase difference between the fundamental and frequency-doubled light is continuously angularly compensated under this strategy,which results the unprecedented and efficient frequency doubling at wavelengths almost covering the deep-UV spectral range from 221 to 332 nm.What’s more,all the possible phase-matching types are originally realized simultaneously under the angular engineering phase-matching conditions.This work should not only provide a novel and practical nonlinear photonic device for tunable deep-UV radiation but also be helpful for further study of the light-matter interaction process.
Introduction
Phase is a “memory” of motion in the classical and quantum wave functions1-3and can record the trajectory and states of particles and waves in time and space simultaneously4,5.Phase engineering of a wave function influences and determines the tendency and even results of physical processes,which could also give rise to abundant fascinating phenomena,such as the soliton,Moiré lattices and topological charge6-8,thus boosting the development of integrated photonics,phononics and electronics9-11.For the electromagnetic wave,its behaviour can be well described by Maxwell’s equation,and manipulation of the phase results in weak-field linear optics,including reflection,refraction,scattering and interferometric control of absorption12-15.Under strong electromagnetic fields with ultrahigh electric field intensity,the interacting matter could respond nonlinearly and generate nonlinear polarization and nonlinear optics16,whose efficiency also depends on the phase relationship,with manifestation of momentum conservation.For example,the energy flow could be inverted when the phase difference reaches π in the second harmonic generation(SHG)process if the phase is mismatched.To realize highly efficient optical conversion,a phasematching condition between the fundamental and harmonic electromagnetic waves is required.This prerequisite was realized in many anisotropic crystals with inherent birefringence,which broadens the available wavelength of laser sources17,18but concurrently precludes many nonlinear materials without suitable birefringence.To break the strict birefringent limitation,the quasi-phase-matching(QPM)technique was proposed in 1962 by employing periodic inversion of the polarization direction of nonlinear crystals19,which has been demonstrated in many ferroelectric materials20-22and applied in classical and quantum optics fields for the generation of frequency conversion,nonlinear imaging,quantum information generation and computation23-25.However,there are also some“clouds”in phase-matching nonlinear optics,e.g.,crystals without suitable birefringence and invertible ferroelectric domains were ruled out,which constrains the further development and application of nonlinear photonics in the broad spectral range,especially in the ultraviolet(UV)region.In addition,the well-designed frequency converters are wavelength- and polarization-dependent,which means that the typical wavelength requires a particular frequency converter with particular phase-matching type.The novel strategy for the efficient tunable frequency conversion and universal nonlinear crystals are expected for dozens of years.
Recently,we proposed a novel and universal phasematching strategy associated with phase manipulation26,named as additional periodic phase(APP)phase matching.Such phase-matching condition is attributed to the artificial manipulation of the optical phase in the periodic ordered/disordered alignments.Meanwhile,the light phase is related to its propagation length in the crystal,thereby giving rise to the possibility of the generation of tunable phase matching if the phase could be angularly engineered in angular APP phase matching,especially in the deep-UV region where the practical nonlinear optical crystals are rare and the available laser wavelengths are discrete up to now.The angular APP phase matching would provide a new way for the development and design of nonlinear optical materials without suitable birefringence but with excellent nonlinear optical properties(e.g.,short UV cut-off edge or large nonlinear coefficient)in the deep-UV region.
Results
Herein,we originally theoretically investigated angular engineering of the additional periodic phase in APP phase matching and experimentally realized efficient tunable SHG(221-332 nm)covering almost the entire deep-UV spectral range with a tunable range of 111 nm for the first time to our knowledge.In addition,all possible phase-matching types are also originally simultaneously achieved with the participation of phase variation induced by the distribution of the grating period within a certain range.This novel and practicable work should be further development of APP phase matching and could inspire further studies in nonlinear optics,photonics and even physics.
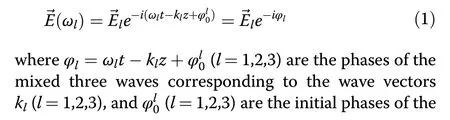
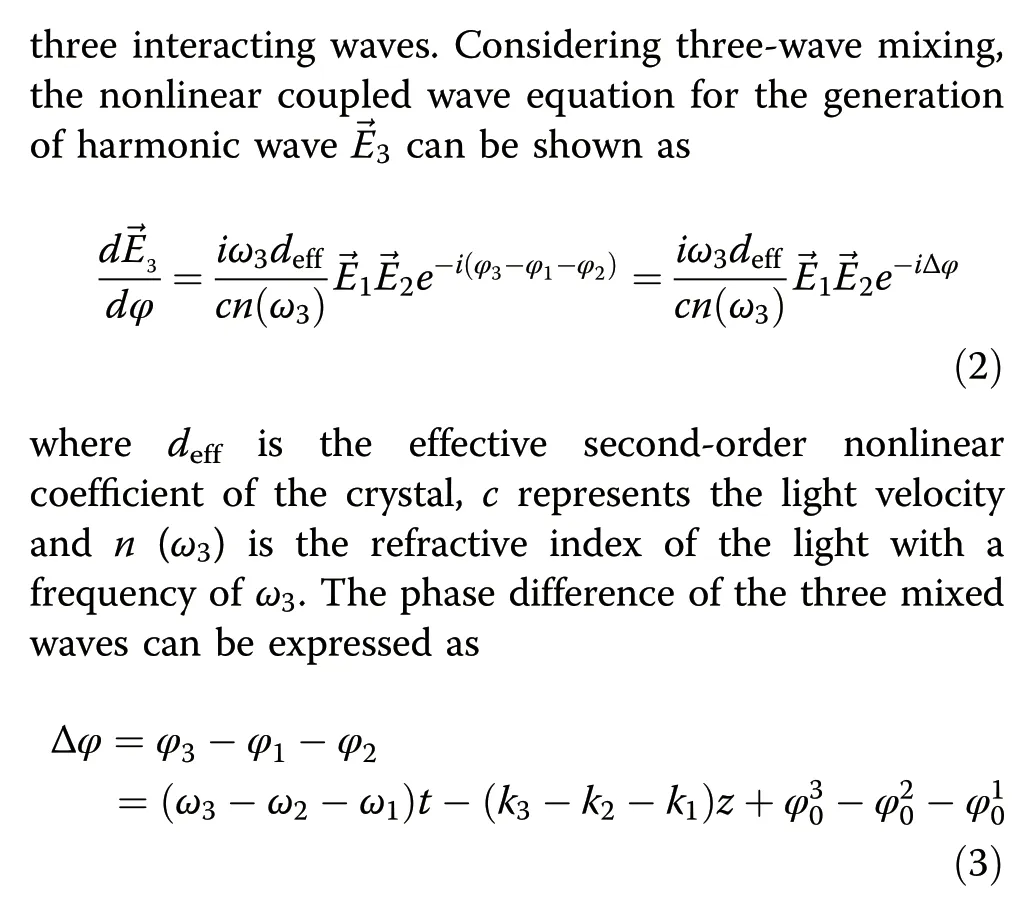
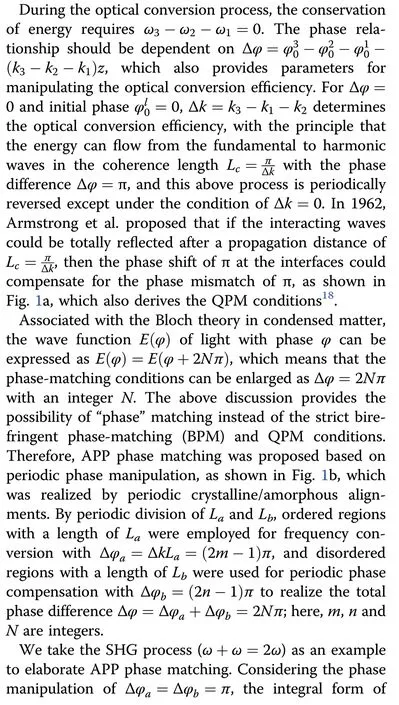
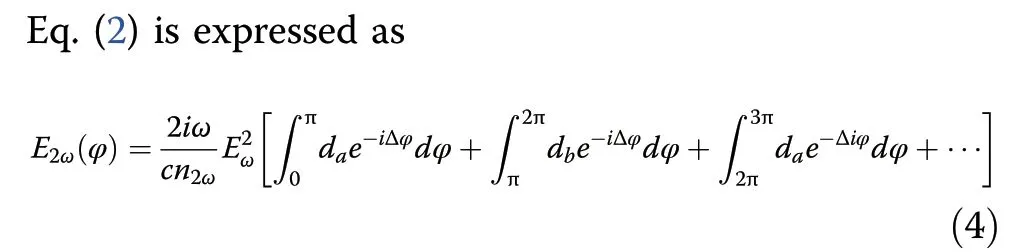
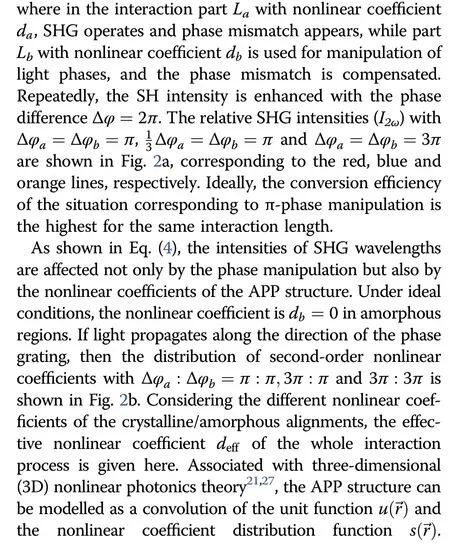
Fig.1 Two different ways to provide phase manipulation if the phase is mismatched.a Schematic of the traditional method with a π phase shift at the interfaces.The interacting waves are totally reflected repeatedly with a propagation distance of Upon each reflection,E(ω)and E(2ω)are inverted with a π phase shift at the interfaces.b Schematic of the APP with a continuous phase change.In crystalline regions,a nonlinear optical process occurs with a phase difference Δφa =(2m-1)π,and then,in amorphous regions,phase manipulation operates with an APP Δφb =(2n-1)π
Under the plane-wave approximation,electric field with a frequency of ωl(l=1,2,3)and an interaction length ofzcan be expressed as16
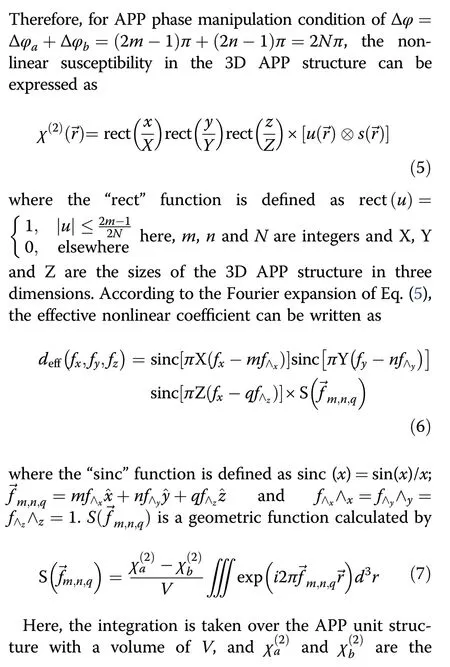

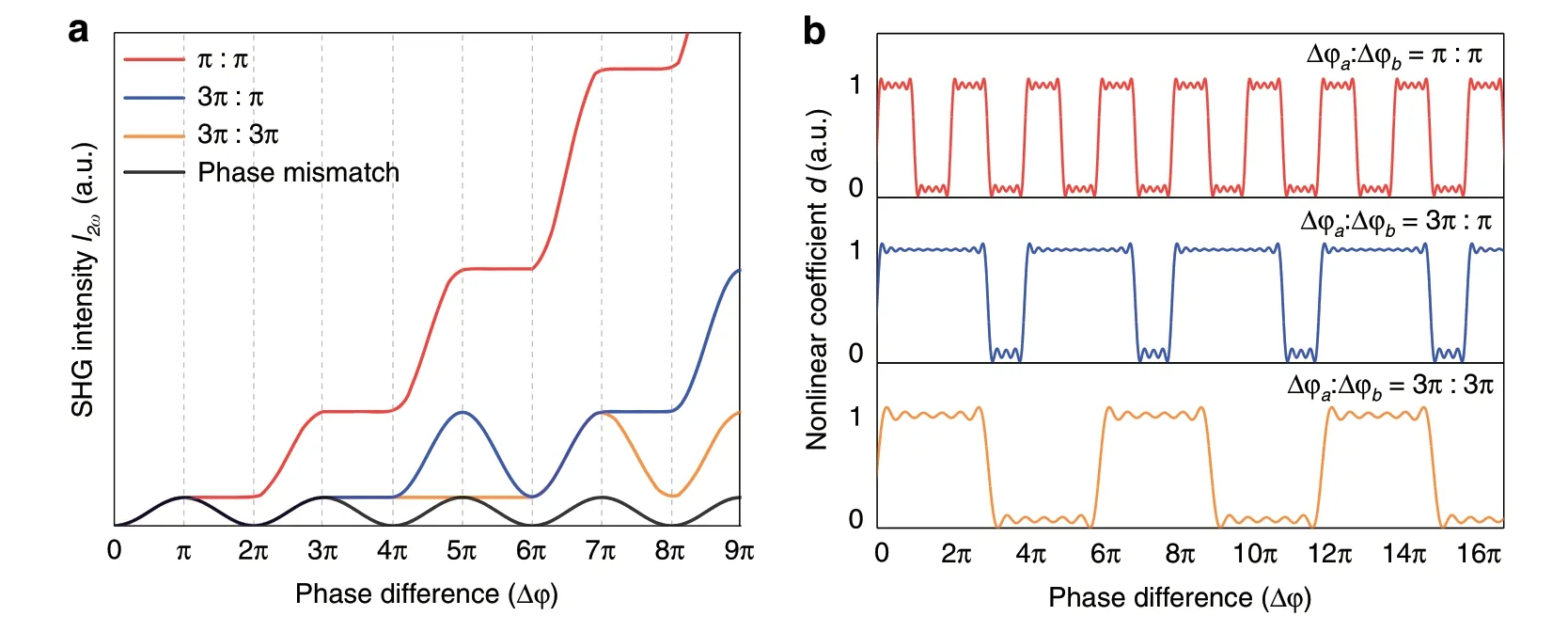
Fig.2 APP phase matching with light phase manipulation for nonlinear frequency conversion.a SHG intensity(I2ω)under phase mismatch and different APP phase-matching conditions with phase difference Δφ in a nonlinear crystal.The relative SHG intensities with light phasemanipulation of Δφa :Δφb =π :π,3π :π and 3π :3π correspond to the red,blue and orange lines,respectively.b Distribution of the second-order nonlinear coefficient d with the phase difference Δφ
Next,we demonstrate the theoretical and experimental feasibility of angular engineering of the APP.If the light propagates in the direction of the phase grating,then a specific SHG wavelength can be obtained by special reciprocal vector compensation.In addition,new angular degrees of freedom in spatial modulation can be introduced with multiple phase grating layers fabricated along the depth direction to obtain large-scale APP crystals,which enables angular APP phase matching(Fig.3a).In this way,we can realize tunable phase-matched SHG with angular engineering of the APP in one APP crystal.Compared with angular BPM limited by strict refractive index dispersion condition Δk=0 or angular QPM depending on ferroelectric materials28-30,the angular APP technique could realize tunable phase matching in the nonlinear optical materials no matter those with or without Δk=0 and reversed ferroelectric domains by continuous phase manipulation of Δφ=2Nπ.Moreover,angular APP strategy should be theoretically suitable for all non-centrosymmetric nonlinear crystals in the entire spectral transmission range.What’s more,large nonlinear coefficients could be employed under APP phase matching condition,which should be more flexible compared to the birefringence phase-matching condition.
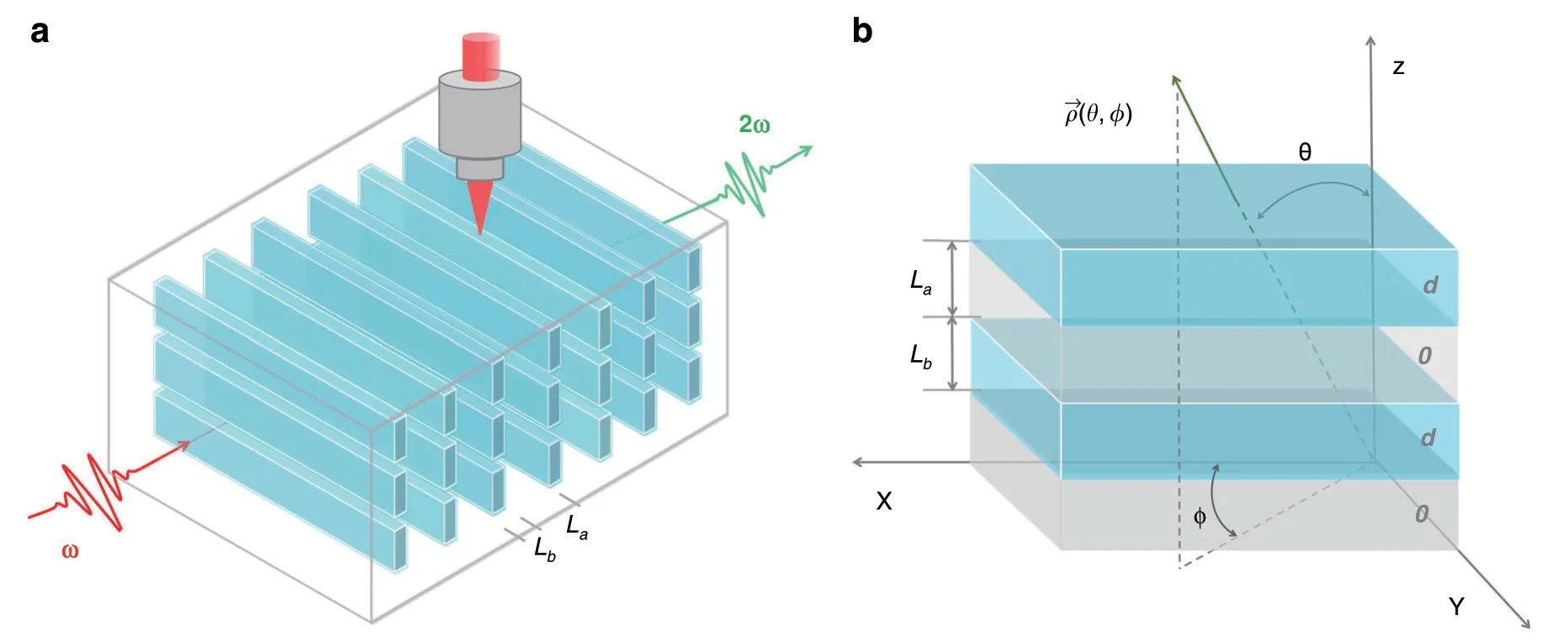
Fig.3 Angular APP phase-matching engineering diagram.a Schematic of laser direct writing of APP gratings.Multiple grating layers can be fabricated along the depth direction to obtain large-scale APP crystals.∧=La+Lb is the fabricated APP grating period,and La and Lb are the lengths of crystalline/amorphous regions of the crystal with second-order nonlinear coefficients of d/0,respectively.b APP gratings along the Z direction.→ρ(θ,?)is the propagation direction of the interacting waves;θ is the phase-matching angle between the propagation direction and optical axis;and ? is the azimuth angle
Taking the structure of APP gratings arranged along thez-axis as an example,the interacting waves propagate along the direction of →ρ(θ,?),where θ is the phasematching angle between the propagation direction andz-axis and?is the azimuth angle(Fig.3b).Besides the changing of grating period length,the function of angle tuning is realized by the manipulation of the optical path to satisfy the phase relation of Δφ=Δφa+Δφb=2Nπ of periodic crystalline/amorphous regions by continuous phase compensations.In the crystalline regions,nonlinear frequency conversion proceeds with the phase difference Δφa(θ,?)=(k3(θ,?)-k2(θ,?)-Associated with the APP phase matching,Δφa(θ,?)=(2m-1)π is required for efficient frequency conversion.For interacting waves with wave-vectors ofkl(θ,?)(l=1,2,3),there are 23types of polarization configurations for angular APP phase matching owing to the two different values of refractive indicesnoandne,wherenoandnestand for the refractive indices of ordinary(o)light and extraordinary(e)light,respectively.Generally,a particular phase angle satisfies typical SHG light with a specific polarization;thus,the eight types of phase-matching conditions cannot coexist for the dispersion of intrinsic refractive indices.Due to the finite processing accuracy,the phase grating period is uncertainly distributed with an error ofLr;thus,an uncertain phase variation Δφris generated that participates in the phase compensation process,providing the possibility for tunable phase-matched SHG with different polarization configurations.Therefore,SHG is concurrently accessible for different types of angular APP phase matching,which greatly utilizes the pump light to improve the conversion efficiency.In the amorphous regions,phase compensation operates withwhereni(i=1,2,3)are the wavelength-dependent refractive indices of interacting waves,regardless of angle.For APP phase matching,Δφb(θ,?)=(2n-1)π from amorphous regions is added to compensate for the phase mismatch in the crystalline regions to realize effective energy conversion.Notably,in APP crystals,the refractive index of the material also varies periodically from the crystalline region to the amorphous region;however,the change in refractive index is very small(<0.005),so the influence on the refraction at the interface of the crystalline/amorphous regions can be ignored26.
We experimentally realized efficient tunable SHG in the UV spectral range with APP quartz,which has been identified as an efficient deep-UV nonlinear optical crystal by APP techniques26.A fibre femtosecond laser with a central wavelength of 1030 nm and a pulse width of 240 fs was used as the writing source.The minimum focused diameter of the femtosecond laser corresponding to amorphous region can be controlled within 2 μm,basically satisfying fabrication requirements of APP periods.The grating error is measured generally to be<500 nm.The phase gratings are arranged along the optical axis of a Z-cut quartz crystal.By optimizing the incident energy of the writing laser,the crystallinity of the crystal is obviously destroyed and transformed into an amorphous state through laser irradiation,which can be confirmed by the significant reduction in the Raman resonance peaks(see Supplementary Information for details,Fig.S2).A 3 mm long APP quartz crystal with a period length ofLa=Lb=2.1 μm for SHG of the laser at a wavelength of 484 nm was fabricated based on the dispersion equation31,corresponding to light phase manipulation of Δφa=Δφb=π.
An optical parametric oscillator(optical parametric oscillator,Opolette HE 355 II)with a pulse width of 10 ns and a repetition frequency of 20 Hz was used as the pump light source.First,angular APP phase matching was experimentally demonstrated in the YZ plane(?=90?)with extraordinary light incidence,corresponding to type(eeo)APP phase matching,and(o)and(e)stand for the ordinary and extraordinary polarizations,respectively.The relationship between thedeffand incident angleof APP quartz in the YZ plane calculated by Eq.(6)can be expressed asWidely tunable phase-matched SHG wavelengths from 221 to 332 nm were experimentally achieved through continuous phase-mismatch compensation by rotating the APP quartz along the X direction(Fig.4a),which cover almost the entire UV range from 200 to 350 nm.The tunable SHG wavelengths are the results of the combination of angle tuning and phase variation participation.By theoretical analysis and fitting(orange line in Fig.4b),the SHG wavelengths from 242 to 332 nm are found to be attributed to angular APP phase matching with phase-matched angle.In addition,the SHG wavelength from 221 to 241 nm is mainly attributed to the participation of phase variation Δφrwith a fixed angle,which is generated by the distribution of the entire grating period Λ=La+Lbwithin a certain range from 3.8 to 4.6 μm with an error ofLr=±0.4 μm.Associated with the statistical analysis of the phase variation Δφrand angular engineering for Δφa(θ,?)and Δφb(θ,?),the experimental SHG signal intensities agree well with the theoretical calculations(purple dashed line in Fig.4c).Therefore,the participation of phase variation Δφrcaused by fabrication error provides a realizable method for tunable phase matching.In fact,the designed APP grating structure and the accuracy of the grating fabrication will both affect the phase matching bandwidth.Besides,there is also another possible way for broadening the phase matching bandwidth by the introduction of the particular patterns(such as chirped periodic patterns,fan-out grating,segmented grating,etc.)into the APP materials for the realization of tunable SHG,which would be performed in the further experiments.
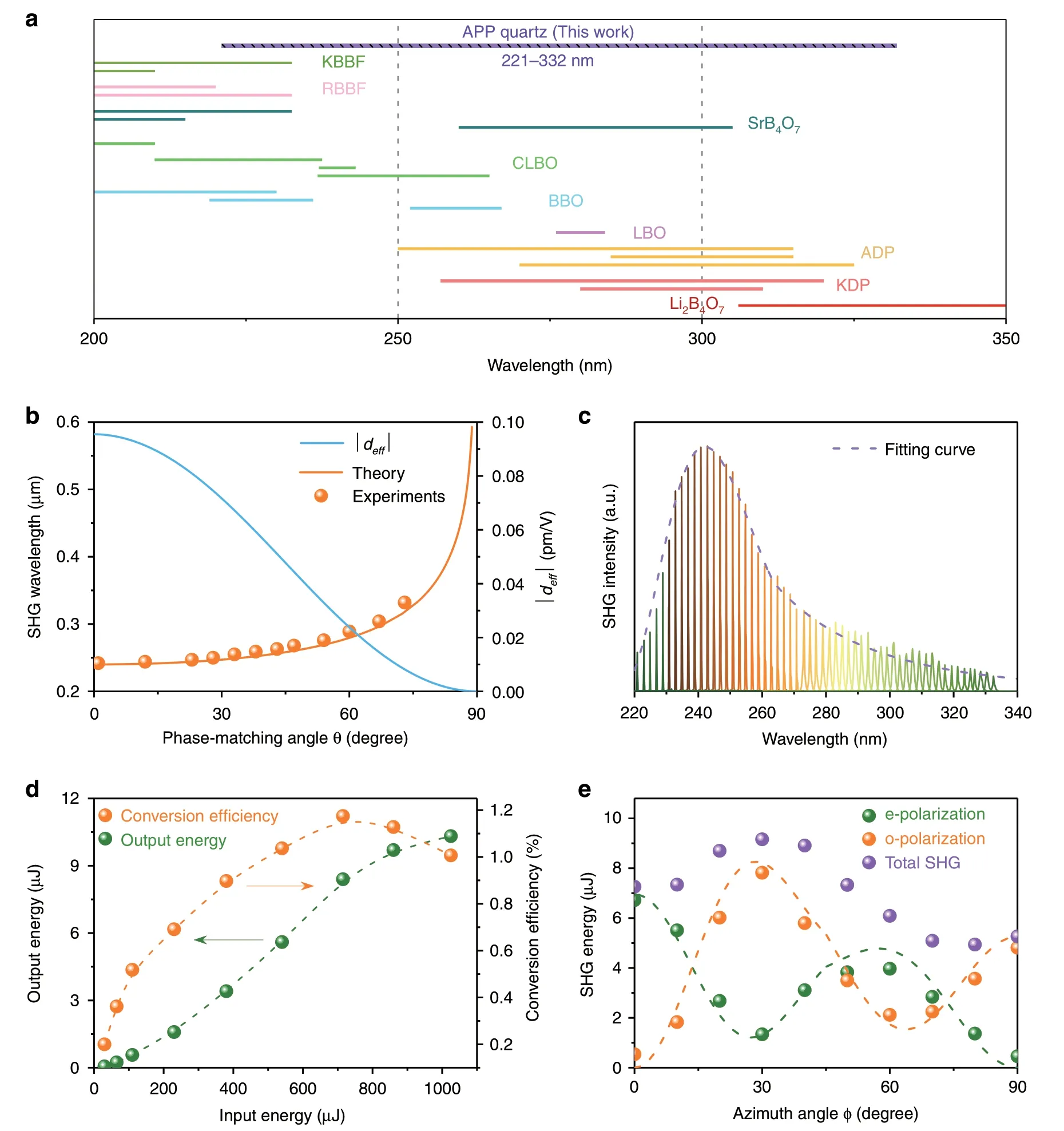
Fig.4 Experimental demonstration of angular APP phase matching with APP quartz with a period of La =Lb =2·1 μm.a Comparison of the tunable spectral region of this work with those of common nonlinear optical crystals in the deep-UV(200-350 nm)range.b Calculation of the angular APP phase-matching angle θ and distribution of absolute value |deff| in the YZ plane when ?=90°.The dots are the experimentally measured phase-matching angles corresponding to SHG wavelengths.c SHG signal intensities at different wavelengths from 221 to 332 nm.The dashed line is the fitting curve of SHG intensities.d Output energy and conversion efficiency of the SHG light at 242 nm.e Calculated(curves)and experimental(dots)SHG intensities for ordinary and extraordinary light at 250 nm with azimuth angle ? tuning from 0°to 90°along the Z direction,where the phase-matching angle is located at θ=29.6°
Compared with other common deep-UV nonlinear optical crystals,APP quartz shows a great advantage in wavelength tunability32-49(Fig.4a),regardless of BPM materials or QPM ferroelectric materials.The SHG energy reaches a maximum of 10.32 μJ under the input energy of 1025 μJ at a wavelength of 484 nm,corresponding to a SHG peak power of 1.46 kW and an optical conversion efficiency of η=1.01%(Fig.4d),which is almost 10 times higher than that of a QPM quartz with the finest twin structure(17.28 W,η=0.12%)at 266 nm50.The conversion efficiency decreases slightly at high energy,which should be induced by the poor quality of the fundamental laser with the increase in the incident energy under the present conditions.The conversion efficiency can be enhanced by improving the laser fabrication accuracy and beam quality and peak power of fundamental light sources.In addition,the efficiency can also be improved with a nonlinear crystal with larger nonlinear coefficients and longer interaction length.Taking the BBO crystal as an example47-49,the realized tunable wavelength range of BBO crystal is about 20 nm in the ultraviolet range as shown in Fig.4a.Moreover,the maximum output power as high as 1.37 W was recent realized at 213 nm with the efficiency over than 17% with a BBO crystal by the well-designed sumfrequency technique18,which indicates that the BBO crystal could be an excellent ultraviolet crystal and suitable for the high-power ultraviolet lasers with the APP strategy.
In addition,for realization of multiple types of APP phase matching,we also investigated the angular APP phase matching with a fixed phase-matching angle θ under arbitrary azimuth angle?.Taking the SHG process of pump light at a wavelength of 500 nm as an example,the phase-matching angles in the present APP quartz could be calculated to range from 25.64°to 35.40°for different types of APP phase matching;i.e.,types{(ooe),(oee/eoe),(eee),(ooo),(oeo/eoo),(eeo)} correspond to {25.64°,27.20°,29.06°,29.89°,32.34°,35.40°},respectively.The incident angle was located at θ=29.6°in the experiment with rotation of the APP quartz along the Z direction.Due to the contribution of the phase variation,all types of angular APP phase matching were observed in the SHG process.The SHG intensities for o light and e light were both measured in the experiment with azimuth angle?tuning from 0° to 90°(Fig.4e).By calculating the contribution of each type of phase matching,the experimental data(dots in Fig.4e)agree well with the theoretical simulation(dashed curves in Fig.4e).The total SHG energy reaches a maximum at an azimuth angle of approximately?=30°,and the output energy of SH light is 9.16 μJ with a fundamental wavelength energy of 1012 μJ,corresponding to o light and e light of 7.82 and 1.34 μJ,respectively.Therefore,the angular engineering by tuning azimuth angle?can be employed not only to select nonlinear coefficients,but also to control the polarization output in different phase matching types,which should be very difficult or unavailable in traditional phase-matching conditions due to the dispersion of refractive index,thus could be further applied in relevant scientific fields,such as laser-induced periodic surface structures,laser-scanning nonlinear optical techniques and even some quantum entanglement fields with the nonlinear upconversion process51-53.
Discussion
In conclusion,we have demonstrated angular engineering of an APP for widely tunable phase-matched SHG and experimentally demonstrated it in an APP quartz crystal.Through the introduction of angular engineering,efficient SHG was realized in an APP quartz with a tunability of 111 nm in the UV range and all possible phasematching types were simultaneously realized.The optical conversion efficiency and peak power are over 1% and 1 kW,respectively,which could be further improved by improving the fabrication techniques and experimental conditions.Angular APP phase matching should provide a new route for nonlinear optics and thus will be key to opening the door for realization of tunable lasers,which could have applications in modern photonics and physics.
Materials and methods
The quartz was cut alongz-axis with the length of about 5 mm.The APP quartz with phase grating period ofLa=Lb=2.1 μm was writing with the energy of 12 μJ by the femtosecond laser(ANTAUS 1030-20)with a pulse duration of 240 fs and a repetition rate of 200 kHz.The femtosecond laser with a central wavelength of 1030 nm was focused inside the crystal through a microscope objective(Mitutoyo 20x,NA=0.35)into the sample,and the crystal is placed on a three-dimensional moving platform with the writing speed of 1 mm s-1.In order to obtain a large-scale APP quartz to meet angular manipulation requirements,five layers phase gratings were fabricated along the depth direction with an interlayer distance of 0.25 mm.
Acknowledgements
This work was financially supported by the National Natural Science Foundation of China(NSFC)(92163207,52025021,51890863),Future Plans of Young Scholars at Shandong University.
Author contributions
H.Y.and H.Z.conceived the idea and designed the experiments.M.S.carried out the sample synthesis,characterization and laser experiments.F.L.provided constructive discussion and participated in the paper writing.All of the authors contributed to the overall scientific interpretation and edited the manuscript.
Conflict of interest
The authors declare no competing interests.
Supplementary informationThe online version contains supplementary material available at https://doi.org/10.1038/s41377-022-00715-w.
 Light: Science & Applications2022年2期
Light: Science & Applications2022年2期
- Light: Science & Applications的其它文章
- Phase-matching-induced near-chirp-free solitons in normal-dispersion fiber lasers
- Toward implantable devices for angle-sensitive,lens-less,multifluorescent,single-photon lifetime imaging in the brain using Fabry-Perot and absorptive color filters
- MXene supported surface plasmons on telecommunications optical fibers
- Nonvolatile electrical switching of optical and valleytronic properties of interlayer excitons
- Microsphere-assisted,nanospot,non-destructive metrology for semiconductor devices
- Probing the orbital angular momentum of intense vortex pulses with strong-field ionization
