Estimation of discharge and its distribution in compound channels*
MOHANTY Prabir Kumar, KHATUA Kishanjit Kumar
Department of Civil Engineering, National Institute of Technology, Rourkela, Orissa, India, E-mail: prbrmohanty@yahoo.com
Estimation of discharge and its distribution in compound channels*
MOHANTY Prabir Kumar, KHATUA Kishanjit Kumar
Department of Civil Engineering, National Institute of Technology, Rourkela, Orissa, India, E-mail: prbrmohanty@yahoo.com
(Received December 9, 2012, Revised April 7, 2013)
Results of research into a compound channel having width ratio (α) in excess of 11 are presented in the form of boundary shear distributions across the compound cross section. New relationship is derived between the percentage of shear carried by the flood plains (%Sfp) and the percentage of area occupied by the flood plains (%Afp). The equation so derived is taken as the basis to develop a new methodology to predict the stage discharge relationship specifically for wide compound channels using Darcy’s friction factor (f) for the main channel and flood plain regions. The methodology also is used for compound channels with smaller width ratios by applying the appropriate relation for %Sfpderived earlier by different researchers and seems to work well. Next, as a corollary to the methodology, separate formulae are proposed to estimate flow distribution in main channel and flood plain regions. The proposed method and its corollary are tested for their validity against well-published small-scale data series of previous researchers along with some large-scale data series from EPSRC-FCF (A-Series) compound channel experiments and very good agreement is observed between the measured values and predicted values for total flow as well as zonal distribution of flow. The methodology is also applied to some compound river section data published in literature and is found to serve well the purpose of predicting flow in real world application. This new method gives the least RMS value of error for discharge prediction compared with some other well-known methods used for estimating stage-discharge relation in compound channels by considering all data sets.
compound channels, boundary shear, stage-discharge relationship, discharge distribution, friction factor
Introduction
Estimating the discharge carrying capacity of a compound river section comprising a deep main channel and one or two adjacent flood plains with sufficient accuracy has been the subject of intense research over last half a century period. There have been many approaches to tackle this complex task. The methods are either based on experimental research where the field conditions are replicated in controlled laboratory environment and different flow aspects, e.g., velocity, boundary shear, secondary flow, turbulence characteristics, etc., are studied under various geometrical or hydraulic characteristics to derive the formulae relating discharge with various flow parameters, or are based on numerical approach where 1-, 2- or 3-D flow modeling is resorted by solving St.Venant’s equations, depth integrated Navier-Stokes equations and complete Reynolds averaged Navier-Stokes equations respectively. In the experimental approach, the resistance to flow for compound channels with different values ofαandβ, whereαis the ratio of width of flood plain to the width of main channel andβis the ratio of depth of flow on flood plain to depth of flow in main channel in the form of point boundary shear stress ()τis measured across various points along the flow perimeter, and useful relationships are derived to quantify the total shear force carried by flood plain or main channel regions in terms of the respective areas occupied by those zones. Knowing the relation between the above parameters and by using some forms of divided channel method (DCM), where the flow section is fragmented to suitable hydraulically homogeneous sections by drawing division lines in horizontal, vertical or diagonal direction from the junction ofmain channel and flood plain regions, many researchers[1,2]have been able to predict stage-discharge relations with sufficient accuracy in compound channels of various geometric and hydraulic characteristics. It has been amply demonstrated by various researchers that a method performing well in predicting the H-Q relation for a particular channel might not be able to correctly assess the flow distribution between the various sub-areas, viz., flood plain and main channel zones. In order to have a general acceptance of a particular approach the latter should successfully achieve the twin objective of predicting with sufficient accuracy, the total quantity of flow as well as flow in various sub-areas with varying stage in the channel[3]. The DCMs although perform better in predicting the overall discharge values in a compound section in comparison to single channel method (SCM) but still the former are not usually effective enough in predicting the zonal discharge with great accuracy[4]. Nevertheless by proper adoption of division techniques for the interface between the floodplain and the main channel and by carefully evaluating some parameters, such as shear percentage carried by the floodplain or the main channel against their respective percentage of area of cross section, many simple and yet very effective models have been developed and have been successfully used to predict stage-discharge relationships in compound channels. These traditional methods (e.g., DCM) have gained acceptance by researchers and engineers in field for their simplicity and effectiveness in predicting stage-discharge relationship for compound channels[1,2]and have been continuously improved upon.
In this regard in our paper, we suggest a new 1-D approach based on new experimental findings in a wide compound channel of high width ratio (=α11.96) by measuring the point boundary shear stress ()τacross the symmetric compound channel section where a central trapezoidal section is flanked by two adjacent symmetric rectangular flood plain sections. The present work is extended albeit in a different way from the previous research work done in compound channels of differentαvalues to predict stage discharge relationship i.e., forαup to 4[1,2], forαup to 5.25[5]and finally forαup to 6.67[6], the details of which are described in later sections in this paper. Using the method also the component discharges are estimated and compared with their observed values in case of several data series published in literature.
1. Materials and methods
For carrying out research in compound channels, the experimental setup was built in the Fluid Mechanics and Hydraulics Laboratory of NIT, Rourkela, India. The dimensions of the compound channel were adopted keeping in view the case of very wide compound channels with width ratio ()αgreater than 11 as seen in most of the natural Asiatic flooded channels and some other channels worldwide. The trapezoidal main channel is flanked by two adjacent symmetric rectangular floodplains. The bed and wall of the channel were made with Perspex sheet (0.006 m to 0.01 m thick and having Manning’snvalue is 0.01) cut to designed shape and dimensions, glued with chemicals and put in position. The roughness was maintained uniform in the main channel and the flood plain because the primary aim of the research was to investigate the impact of momentum transfer due to geometrical parameters only.
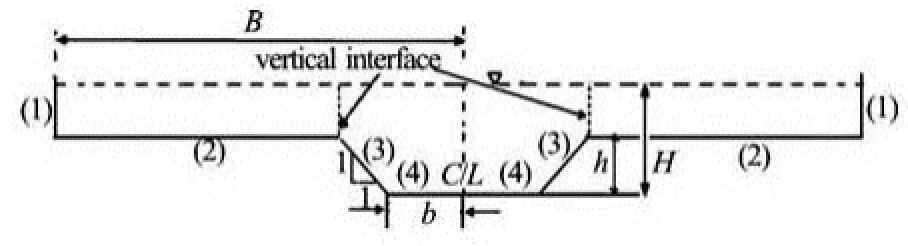
Fig.1(a) Schematic cross section for wide compound channel at NIT, Rourkela
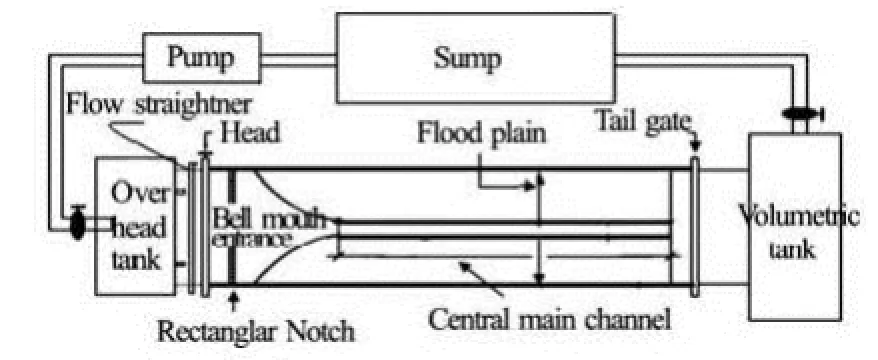
Fig.1(b) Plan view of experimental set up
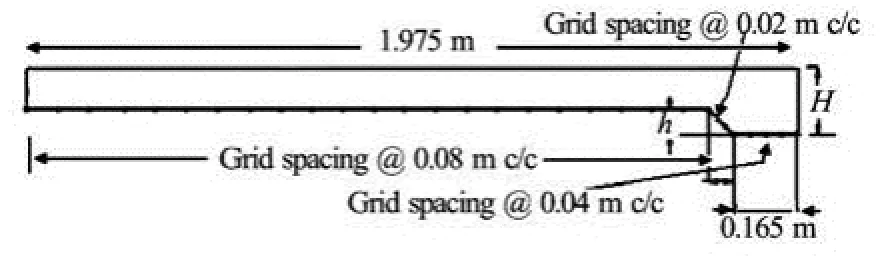
Fig.1(c) Typical grid used for measurement of boundary shear
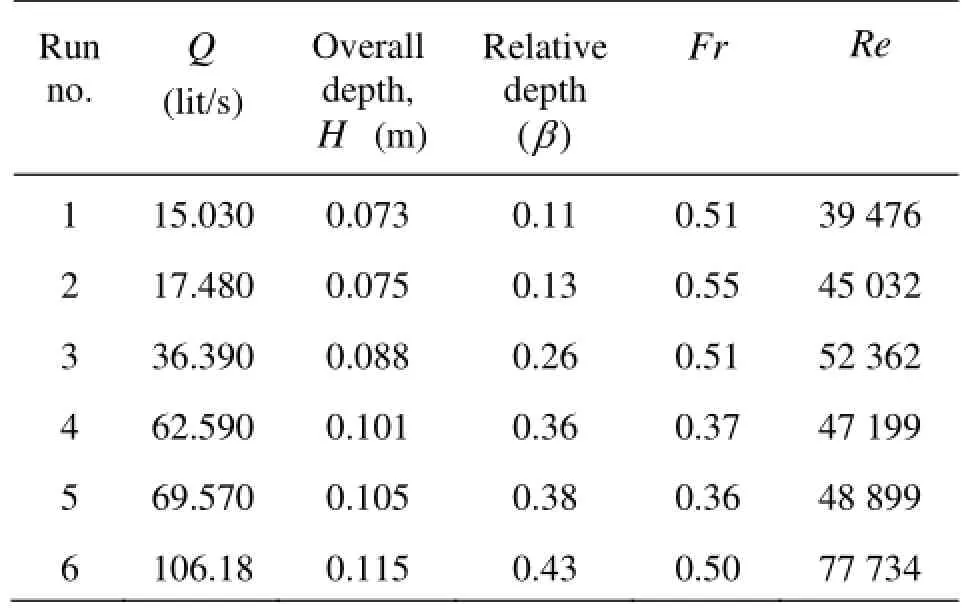
Table 1 Hydraulic parameters for the experiments at NIT, Rourkela
The whole compound channel was cast inside a rectangular steel tilting flume of about 15 m in length. The total width of the section including the floodplains (2)Bwas kept at 3.95 m and the bottom width of the main channel (2)bis 0.33 m, depth 0.065 m and side slope 1:1 with the aspect ratio ()δkept at value of 5, whereδis the ratio of bottom width of the main channel to the depth of the main channel section (Pl. see Fig.1(a) for the schematic cross section of the channel and Table 1 for hydraulic parameters of the experimental runs). The bed slope was kept fixed as 1.1×10-3for this particular set of experiments. Water was supplied to the flume from an underground sump via an overhead tank by a series of centrifugal pumps and recirculated to a large sump after flowing through the compound channel and a downstream volumetric tank fitted with closure valves for calibration purpose. Water entered the channel bell mouth section via an upstream rectangular notch specifically built to measure the continuous discharge in such a wide laboratory channel. An adjustable vertical gate along with flow straighteners was provided in the upstream section sufficiently ahead of rectangular notch to reduce turbulence and velocity of approach in the flow near the notch section. At the downstream end another adjustable tail gate was provided to control the flow depth and maintain a quasi-uniform flow in the channel (Fig.1(b) shows the experimental setup). A movable bridge fitted with all instruments such as point gauge (least count 0.0001 m), a series of micro-Pitot tubes etc. was provided across the flume for both spanwise and streamwise movements of personnel and instrument over the channel area so that each location on the plan of compound channel could be accessed for taking measurements.
The boundary shear stress was measured by a micro-pitot static tube (O.D. 0.00477 m) used as a Preston tube on various points across the wetted perimeter of the compound channel. Due to the symmetry of the section about a central vertical axis only a half of the cross section was used for measurement of point boundary shear stress ()τ. The points of measurement were spread over the entire half of the perimeter with a spacing of 4cm inside the main channel bed, a spacing of 0.02 m over the main channel wall, spacing of 0.08 m over the floodplain bed and at 0.02 m over the floodplain wall. Figure 1(c) shows the grid used for taking the measurements of boundary shear over the perimeter. The static and dynamic pressures were recorded and by suitable calibration technique the point boundary shear stress ()τacross the perimeter was estimated. After that the shear stress values were integrated over the entire perimeter to calculate the total shear force carried by the compound section. The total shear force thus computed was then compared with resolved component of weight force of the liquid along streamwise direction and if the difference was found to be greater than 10%, the experimental run was discarded and repeated once again. When the discrepancy was within 10% then the difference in shear was distributed to floodplain zone and main channel proportionately with their respective weighted perimeters.
2. Methodology
In the present work, an attempt is made to apply the Darcy-Weisbach equation to a straight compound channel incorporating the effect of momentum transfer between the main channel and floodplain and the distribution of zonal friction factors (fz). The compound section can be decomposed into one main channel section and two symmetrical floodplain sections by drawing vertical lines at the junction of the main channel and the floodplains and denoting the whole area of compound section asA, that of main channel asAmcand that of floodplain sections asAfp, from 1-D analysis the average shear stressτzin any zone can be expressed as

wherefzis the average Darcy is friction factor in a particular zone,ρthe water density andUzthe the average zonal streamwise velocity. For the floodplains we can write shear forceSfpcarried by it per unit length perpendicular to the channel cross section as

wherePfpis the floodplain wetted perimeter. From Eqs.(1) and (2) one can find an expression for average flood plain velocity

Or in terms of percentage of shear force in the floodplain area (%fpS, where %Sfpis defined as the percentage of ratio of shear force per unit length carried by the floodplains to the total shear force per unit length carried by the whole compound section ), we can give the above expression as

where the subscriptfpdenotes respective terms used for floodplain. Similarly giving an expression for main channel region

Or in terms of percentage of shear force carried by main channel (%mcS, where %mcSis defined as the percentage of ratio of shear force per unit length carried by the main channel to the total shear force per unit length carried by the whole compound section)

where the subscriptmcdenotes respective terms in main channel region. For most of the channels normally the bed and wall roughness are given in terms of Manning’s roughness value,n. However Darcy’s friction factorf, Manning’s roughnessnand Chezy’s coefficientCare related by the relation
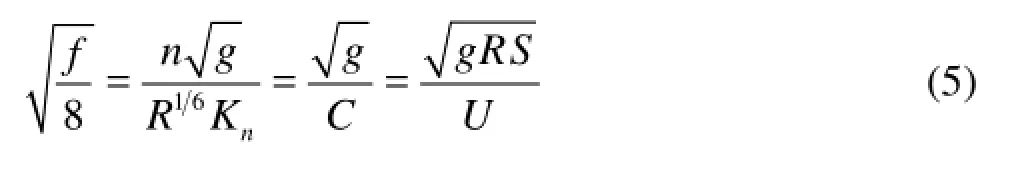


3. Estimation of boundary shear
Previous researchers[1,2,5,6]have estimated boundary shear carried by various zones such as floodplain and main channel regions by conducting laboratory experiments on compound channels having different geometric and hydraulic parameters (e.g.,αandβ). It has been shown that the percentage shear force carried by different zones is a non- linear function of the percentage area occupied by that zone. In other words, %Sfpand %Smcare usually power or exponential functions of %Afpand %Amcrespectively. For compound sections having different width ratios, generally different relationships hold well and as in the present case the width ratio is in the range of 6.67-12 so a new expression has to be developed which would be applicable in the present case. In the next few paragraphs the sequential development of the expressions for different compound sections with different values ofαis briefly outlined as a prelude to the latest developed model of boundary shear.
Various boundary elements comprising the wetted parameters are labeled as (1), (2), (3) and (4) in Fig.1(a). Label (1) denotes the vertical wall(s) of floodplain of length ((H-h)), whereHis the total depth of flow from main channel bed, andhis the depth of main channel. Label (2) denotes floodplain beds of length (B-b), whereB=1/2 (total width of compound channel), andb=1/2 width or bed of main channel represented by label (4) .Label (3) denotes the inclined main channel walls of length (2h). The shear stress distributions at each point of the wetted perimeter are numerically integrated over the respective sub-lengths of each boundary element (1), (2), (3), and (4) to obtain the respective boundary shear force per unit length for each element in the half section of the symmetric channel cross section. Twice the sum of the boundary shear forces for all the elements thus calculated in beds and walls of the compound channel gives the total shear force resisted in the whole compound section and is used as a divisor to calculate the shear force percentages carried by the boundary elements (1) through (4). The percentage of shear force carried by floodplains comprising elements (1) and (2) is represented as %Sfpand for the main channel comprising elements (3) and (4) is represented as %Smc.
Following Knight and Demetriou[1], Knight and Hamed[2]proposed an equation for %Sfpfor a compound channel section as
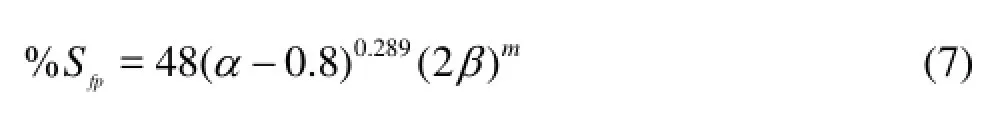
Equation (7) is applicable for the channels having equal surface roughness in the floodplain and main channel. For channels with non-homogeneous roughness, the equation was improved by Knight and Hamed[2]as
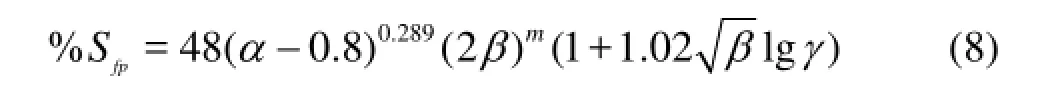
The exponentmcan be evaluated from the relation

For homogeneous roughness section (=γ1, whereγis defined as ratio of floodplain roughness to the main channel roughness), Eq.(8) reduces to Eq.(7). The adequacy of the Eq.(8) for smaller width ratio channels has been shown in Ref.[2]. A regression analysis was also made by Khatua and Patra[5]and they proposed an equation for %fpSas

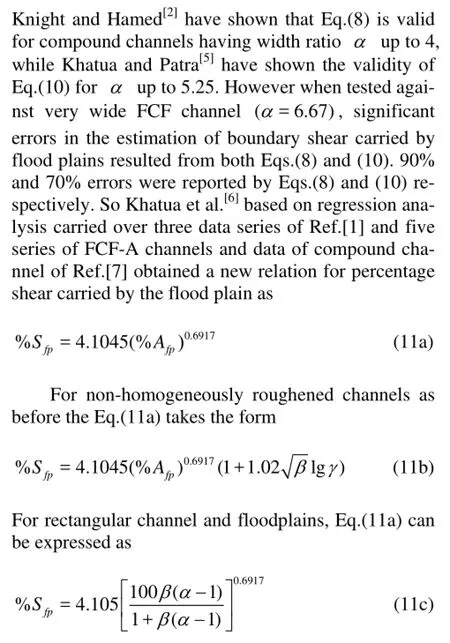


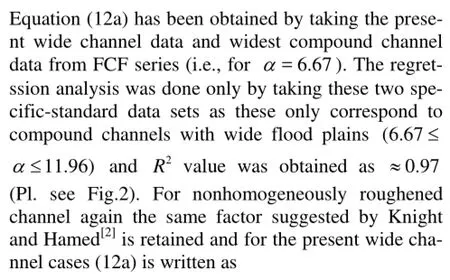
Fig.2 Regression curve for shear force carried by flood plain with area of floodplain
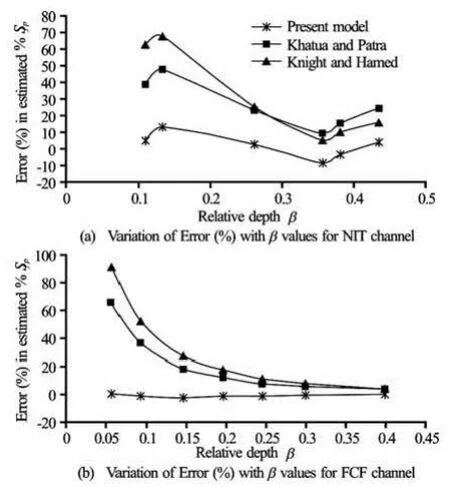
Fig.3 Comparison for %fpS for various models in wide NIT channel and FCFA-1 channel
For trapezoidal main channel and rectangular floodplains, in terms of non-dimensional parametersα,β,δands, where “s” is the value of side slope of trapezoidal main channel (1:s::V:H) the percentage of floodplain area can be simplified asFor the trapezoidal main channel having side slope 1:1VHas in the present case and rectangular flood plains, =1sand Eq.(13) reduces to
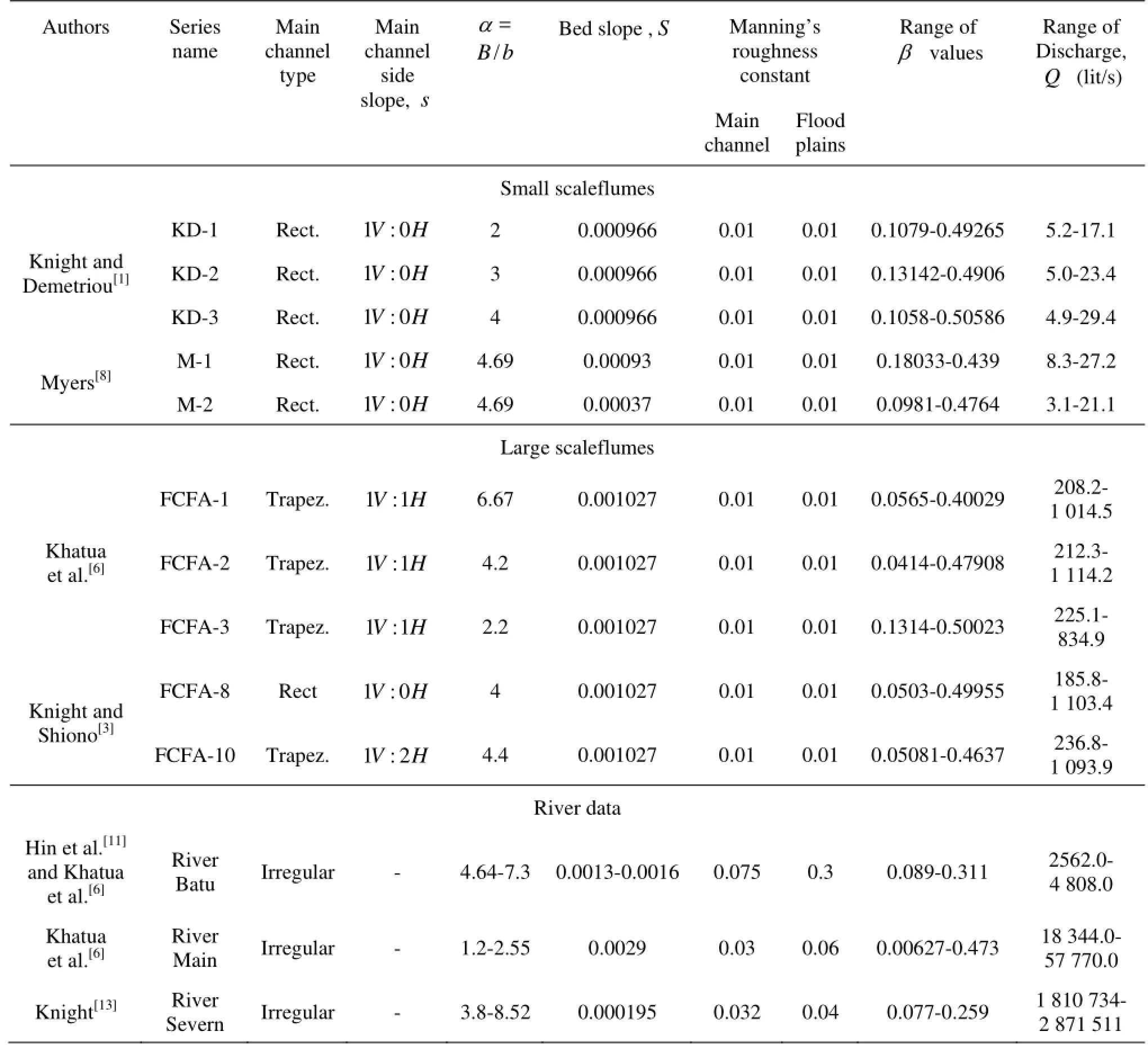
Table 2 Overview of experimental and natural river data considered for validation
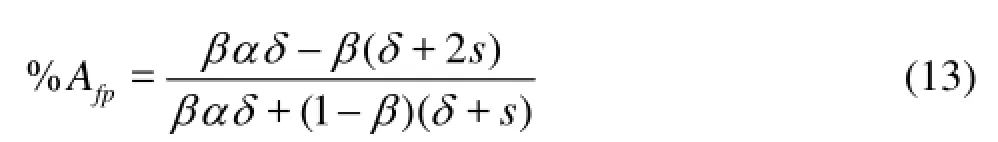
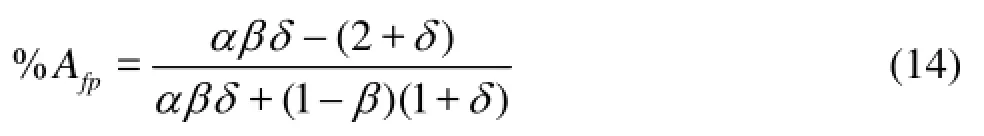
So from Eqs.(12a) and (14) the shear force carried by floodplains can be expressed in terms of non-dimensional parameters defining the compound channel as
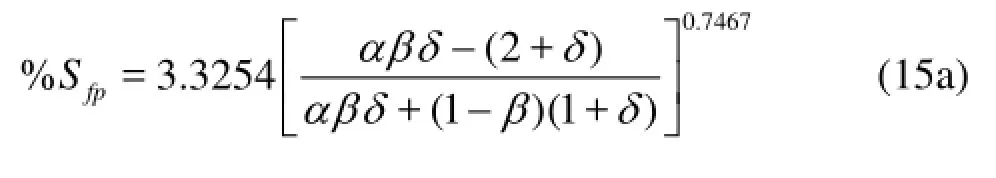
For non-homogeneity of floodplain and main channel roughness, Eq.(15a) is to be modified as
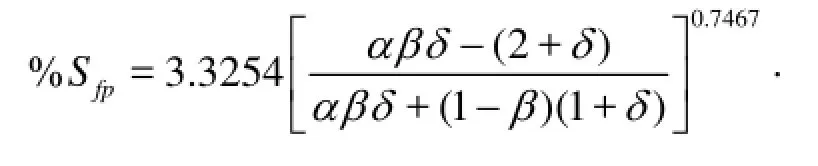

Equations (15a) and (15b) are alternative forms of Eqs.(12a) and (12b) respectively and the former pair is to be used for all types whereas latter pair is to be used in case of trapezoidal main channel and rectangular floodplains specifically. The percentage error in estimated shear carried by flood plains (%Sfp) by Eq.(12) or Eq.(15) is the least when compared to previous models for both NIT channel as well as FCF-A1 channel and are shown in Fig.3.
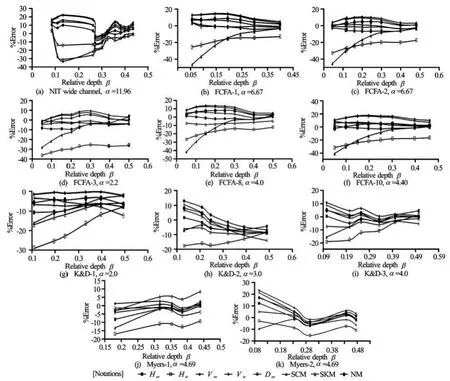
Fig.4 Error (%) in discharge estimation for different data series
4. Results and discussion
4.1Flow estimation for small-scale and large-scale data
The method so developed has been applied to a number of small-scale and large-scale data widely published in literature to estimate the stage-discharge relationship in addition to the experimental data of NIT channel. Three data sets of (K and D Series)[1]and two data series (M-1, and 2)[8]which are smallscale laboratory experimental data and five large-scale data sets from EPSRC-FCF (Series A-1, 2, 3, 8 and 10) are selected to test the method for its validation. The overviews of these experimental data are provided in Table 2. The above data series consisting both of small-scale and large-scale flume experiments have long served the purpose of being used as benchmark data series and so have been widely used by various researchers[5,6]etc. in the field of compound channels to verify and validate their respective stage-discharge models. The width ratioαvaries from 2 to 4 in case of K and D series, is 4.89 in case of M-1 and 2 series and is from 2.2 to 6.67 in case of FCF-A series channels. Also some compound channels are rectangular and some are trapezoidal as evident from Table 2. While applying the method for discharge estimation, for calculating the shear force percentage carried by the floodplains forα≤6.67, Eq.(11a) and forα≥6.67, Eq.(12a) have been chosen so as to correctly represent the shear percentage (%Sfp) in terms of percentage of area occupied by floodplains (%Afp). The new method (NM) is pitted against some traditional methods such as the single channel method (SCM), divided channel methods (DCMs) consisting of horizontal division methods with length of interface excluded and included for calculVeeandVierespectively) and a diagonal division method with the length of interface being excluded (Dee), and finally the SKM method of Shiono and Knight[9]. The discharge is estimated in each method and compared with actual discharge to find the percentage of error for each flowdepth in each case as in Eq.(16).
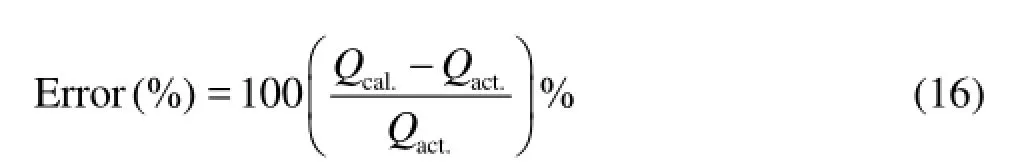
whereQcal.is calculated or estimated discharge andQact.is actual or observed discharge.
4.2Application to field data
Any method or approach to predict the stage-discharge relation must pass through the test of satisfactorily performing in realistic situations, i.e., for field cases or rivers. For this purpose three distinct cases, much publicized in literature are chosen to test the relevance of our method in real applications. As against the laboratory flume experiments, where the flow takes place under human control, the same does not hold in case of rivers under flood. Both the cross-section and roughness are much irregular in comparison with the laboratory flumes. So field data needs to be very carefully obtained and must undergo rigorous testing before being accepted for validation purpose. In view of this, only river data published in well referred journals have been considered here. The first one is an equatorial river named the Batu[11,6]flowing in Kuching, the capital city of Sarawak State, Malaysia.
The next river data is the Main[12,6]. The River Main is located in County Antrim, Northern Ireland. It rises in the Antrim Plateau about 6 km south-east of Ballymoney, County Antrim. The dimensions include a top width of 14 m, a total width inclusive of floodplains of 27.3 m-30.4 m, and bankfull depth ~0.9 m-1.0 m.
The third river data is for the Severn at Montford Bridge (in England), as this site has been extensively monitored for practical hydrometry and research purposes, providing a large body of accurate current metering data[13]. It is a natural cross-section located in a straight reach with a cableway extending over the full width including the floodplains .The bankfull width and depth are 40 m and 6.3 m respectively. The total width, including the floodplains, is approximately 120 m and the reach-averaged longitudinal bed slope is 0.000195.
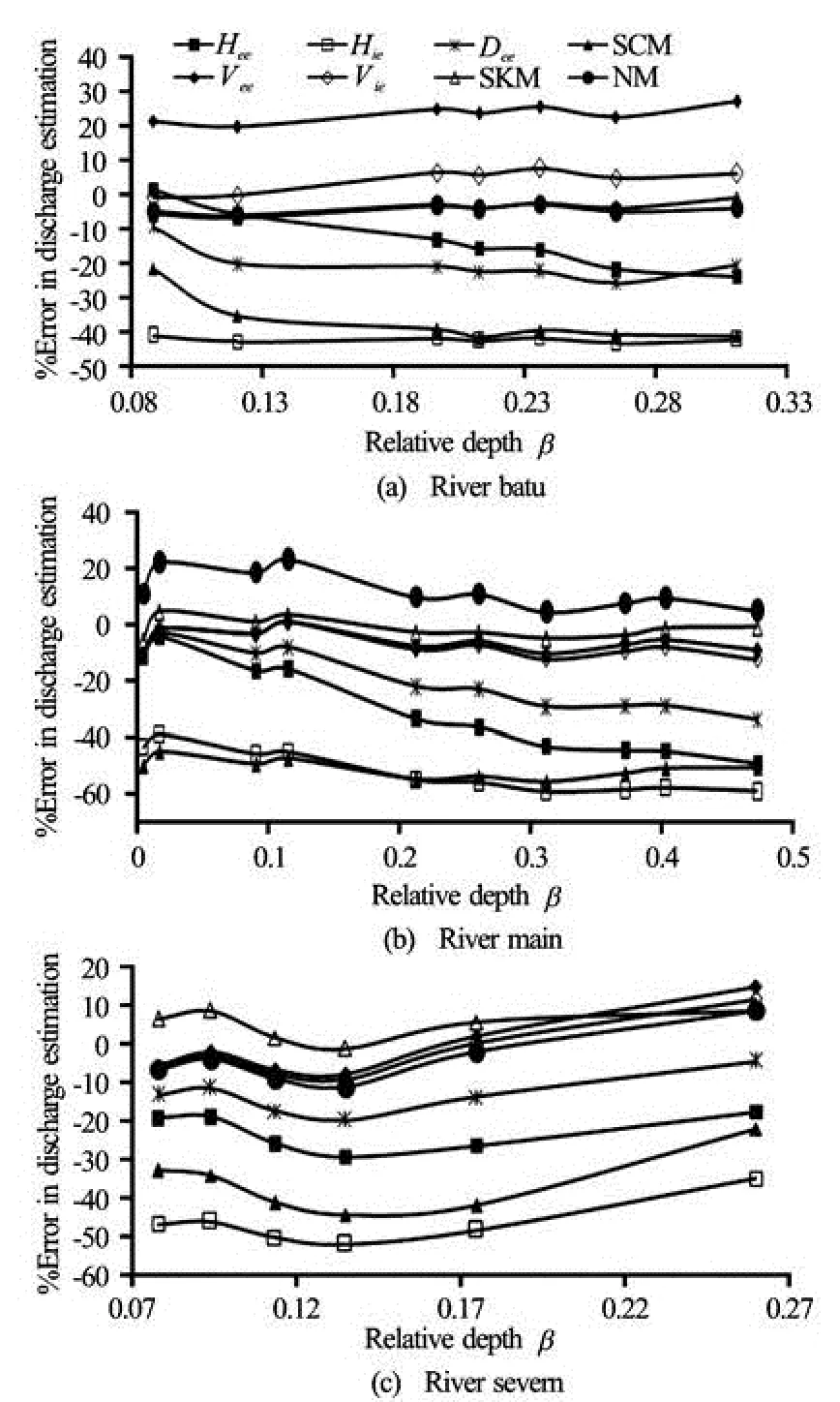
Fig.5 Error (%) in discharge estimation for river data
The main channel and floodplain characteristics and relevant information for all three rivers are collected from the above cited references and are also given in Table 2 in this paper for ready reference. All the rivers have also non-homogeneous roughness along their perimeter, i.e., floodplain roughness are coarser than that of main channel. So accordingly for calculating the shear force percentage carried by the floodplains forα≤6.67 Eq.(11b) and forα≥6.67 Eq.(12b) have been used instead of Eqs.(11a) and (12a) respectively used in case of laboratory flumes having homogeneous roughness (the same Manning’snvalue) in floodplain and main channel, so as to correctly predict the shear force percentage (%Sfp) in terms of percentage of area occupied by floodplains(%Afp). Then after estimating the shear force the usual process of finding the discharge by NM as explained above is followed. All the other methods are also applied to these river data for comparison. The error in estimation of discharge by various methods is shown in Fig.5.
As is evident in all three diagrams in Fig.5 again the NM is among the best of the methods when predicting stage-discharge relation in field application. In all the data series considered in this paper, it is seen that methods sometimes over-predict and sometimes under-predict the discharge amount with varying relative depth there by resulting in positive and negative errors.
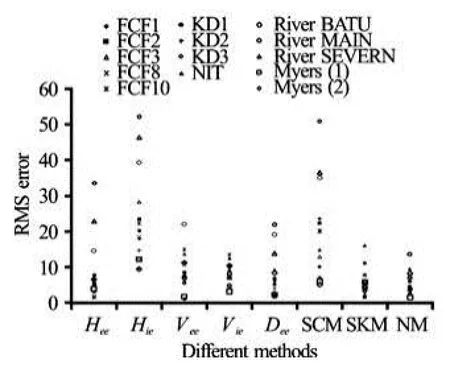
Fig.6(a) Top-series wise RMSE value for the methods
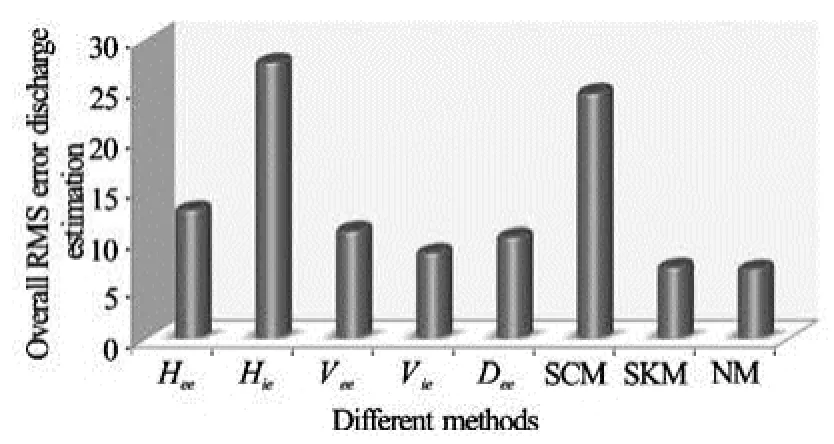
Fig.6(b) Bottom-overall RMSE value for the methods
So in order to make a comparison among all the methods regarding their performance in individual data series as well as their overall performance considering all 14 data sets, the root mean squared error (%) (RMSE) is computed. It is seen that both the horizontal methods (HeeandHie), diagonal method (Dee) and NM give less error as compared to other methods for NIT channels only. ForHee, RMSE is 6.94 and for NM it is 9.67. For FCF-A series (1, 2, 3, 8 and 10) channels the RMS values of the error (%) for NM are estimated to be 6.2, 3.78, 3.01, 5.99 and 3.75 respectively. Similarly considering the (KD-1, 2 and 3) series channels the performance of NM is again very encouraging as the RMSE values are 4.6, 6.1 and 2.6 respectively for the channels. Figure 4 along with Fig.5 adequately achieves the effectiveness of the NM in successfully predicting the stage-discharge relation under a widely varying range of geometric and hydraulic parameters encountered in compound channels. Figure 6(a) shows the performance of individual methods in a series manner, whereas Fig.6(b) shows the overall performance of all the methods. As can be seen from Fig.6(a) the best three methods are NM, SKM andVie, whereas bottom ranking two methods are SCM andHie.
The RMSE for overall performance is shown in Fig.6(b) and it is seen that the values for NM, SKM andVieare 7.31, 7.47 and 8.94 respectively, whereas those for SCM,Hie,Hee,VeeandDeeare 24.79, 27.80, 13.10, 11.08 and 10.49 respectively. The series RMSEs for NM are given in Table 3. It clearly confirms the NM as a very viable tool for discharge estimation in compound channel.
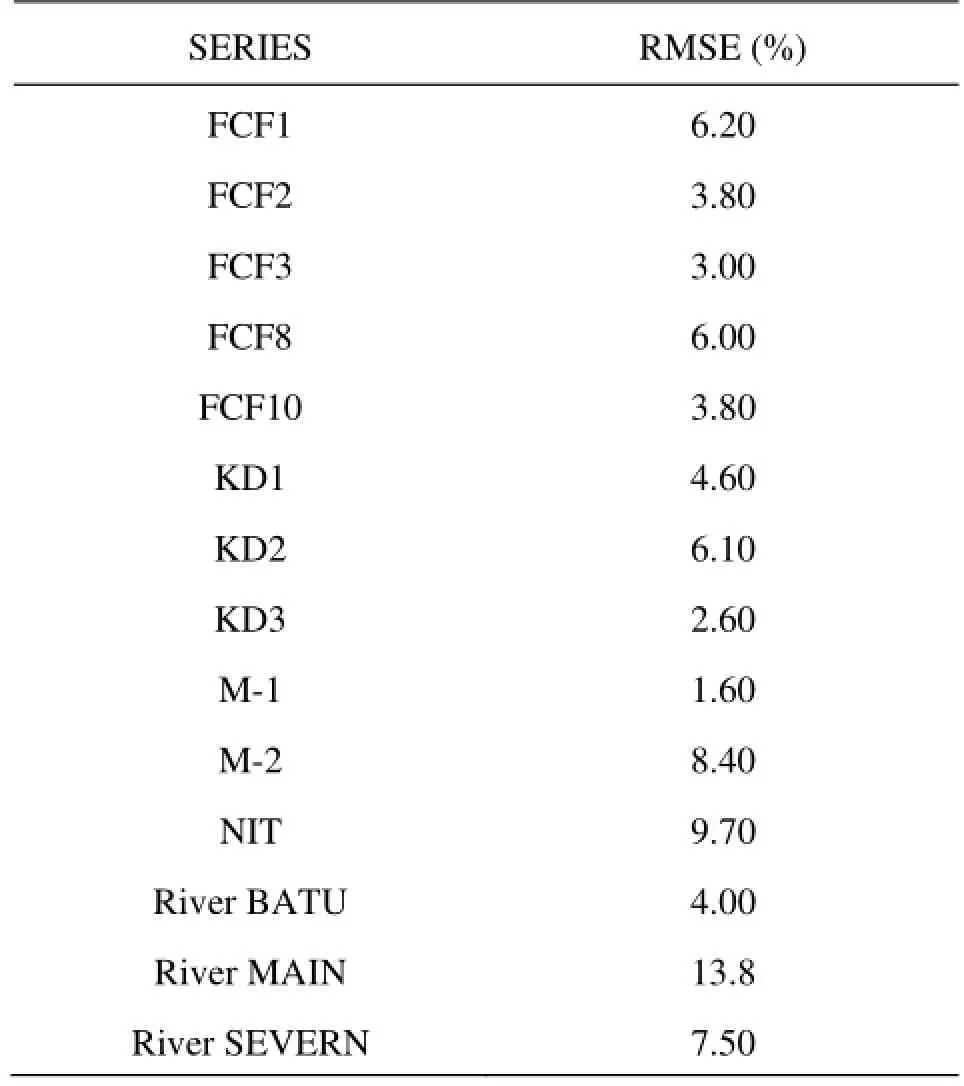
Table 3 Series wise RMS value of error (%) for new method
5. Flow distribution
It is a well-known fact that for a method to be considered successful, it must model accurately not only total discharge in the compound section, but also the component discharges in the deep section and floodplains[8,14]etc.. So the present method is tested in this regard by computing component discharges in floodplain and main channel zones by the procedure as outlined above. The component discharge data of FCF-A series channels, KD series channels and for river Batu are only available in literature to the best knowledge of authors and so are only used here for validation of NM. The observed and predicted mainchannel discharge percentages in terms of ratio of main channel discharge (Qmc) to total discharge (Q) for the series considered are shown in the scatter diagram given in Fig.7. It is evident from the diagram that the zonal discharge predictions are very close to their observed values.
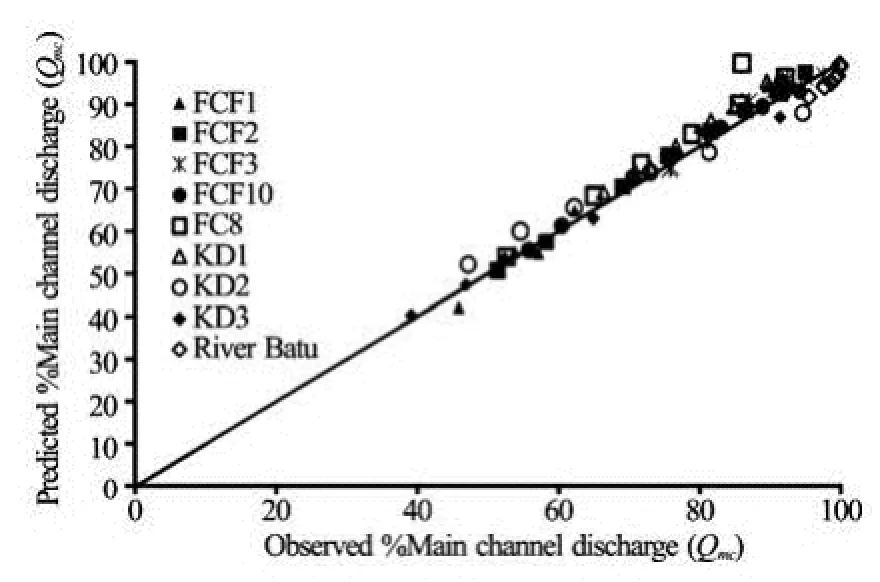
Fig.7 Scatter diagram for observed and modeled main channel discharge percentage
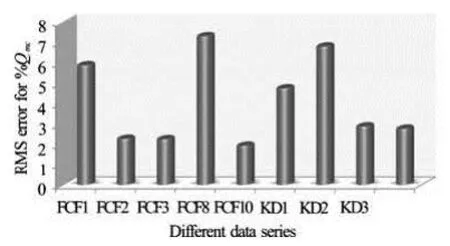
Fig.8 RMSE value for flow distribution by the NM
The series RMSE for discharge distribution is estimated and is shown in Fig.8. It is seen that in all the data series considered for this purpose, the present method can predict the flow distribution by estimating main channel discharge with RMSE smaller than 10% in each case and average RMSE is 4.17% only.
6. Conclusions
The following conclusions can be drawn from the studies reported in this paper.
(1) Fresh experiments conducted in a straight compound channel with very large width ratio (=α11.96) reveal that the shear force carried by the floodplains has a nonlinear relation with area covered by the floodplains and a new mathematical expression is derived on the basis of regression analysis with the coefficient of determination2Rvalue of 0.967.
(2) The new model for shear force percentage gives the least error in large-scale FCF widest channel (series A-1) as well as in the experimental channel of NIT, Rourkela when compared with the models of previous researchers and is found to predict shear force carried by the floodplains with sufficient accuracy.
(3) When different common approaches are applied to the new wide compound channel, it is found that the SKM method and SCM method give larger overall RMS error whereas the horizontal method (Hee) gives the best result. However a unique feature is also noticed for SCM that beyond theβvalue of 0.27, the performance of SCM is quite acceptable. It thus implies that a very wide compound channel behaves as a single channel at a relative depth much smaller than 0.5 which has been the usual characteristic of less wide channels.
(4) A new method is suggested based on zonal variation of friction factor (f) in the compound channel to predict total discharge carried by the whole section and component discharges carried by the floodplain and main channel.
(5) The method is able to predict stage-discharge relationship and component discharges for straight compound channels of different geometrical shapes and over a range of width ratios. This method is validated for its effectiveness over a wide range of publicshed data series comprising small-scale flumes, largescale flumes (FCF series) as well as some field data and is also compared with some other well is established models for discharge estimation as regards their accuracy in predicting stage discharge relation.
(6) The new method has very satisfactorily predicted the total discharge in all cases and is found to have the least overall RMSE in discharge estimation among all the methods considered.
(7) The method also is able to predict component discharges in a very satisfactory manner for both flume data series and river data series. Being a simple and yet very effective tool, it is hoped the new method will be useful for researchers and field engineers alike for discharge estimation in compound channels over a range of width ratios (αvalues from 2 to 12).
Acknowledgement
The authors wish to acknowledge thankfully the support received by the second author from DST India (Grant No. SR/S3/MERC/066/2008) for conducting experimental research work.
[1] KNIGHT D. W., DEMETRIOU J. D. Flood plain and main channel flow interaction[J]. Journal of Hydraulic Engineering, ASCE, 1983, 109(8): 1073-1092.
[2] KNIGHT D. W., HAMED M. E. Boundary shear in symmetrical compound channels[J]. Journal of Hydraulic Engineering, ASCE, 1984, 110(10): 1412-1430.
[3] ANDERSON M. G., WALLING D. E. and BATES P. D. Floodplain processes[M]. Chichester, UK: Wiley, 1996, 139-181.
[4] WEBER J. F., MENéNDEZ A. Performance of lateral velocity distribution models for compound channel sections[C]. River Flow 2004, Procedings, International Conference on Fluvial Hydraulics. Rotterdam, The Netherlands: A. A. Balkema, 2004, 1: 449-457.
[5] KHATUA K. K., PATRA K. C. Boundary shear stress distribution in compound open channel flow[J]. ISH Journal of Hydraulic Engineering, 2007, 13(3): 39-54.
[6] KHATUA K. K., PATRA K. C. and MOHANTY P. K. Stage-discharge prediction for straight and smooth compound channels with wide floodplains[J]. Journal of Hydraulic Engineering, ASCE, 2012, 138(1): 93-99.
[7] KHATUA K. K. Interaction of flow and estimation of discharge in two stage meandering compound channels[D]. Doctoral Thesis, Rourkela, India: National Institute of Technology, 2008.
[8] MYERS W. R. C. Velocity and discharge in compound channels[J]. Journal of Hydraulic Engineering, ASCE, 1987, 113(6): 753-766.
[9] SHIONO K., KNIGHT D. W. Turbulent open channel flows with variable depth across the channel[J]. Journal of Fluid Mechanics, 1991, 222: 617-646.
[10] BHOWMIK N. G., DEMISSIE M. Carrying capacity of flood plains[J]. Journal of the Hydraulics Dvision, ASCE, 1982, 108(HY3): 443-453.
[11] HIN L. S., BESSAIH N. and LING L. P. et al. A study of hydraulic characteristics for flow in equatorial rivers[J]. Internatinal Journal of River Basin Management, 2008, 6(3): 213-22.
[12] MYERS W. R. C., LYNNESS J. F. Flow resistance in rivers with floodplains[R]. Final Report on research grant GR/D/45437, Londonderry, UK: University of Ulster, 1990.
[13] KNIGHT D. W. River channels and floodplains[R]. Final Report for severn trent water authority, severntrent water authority, 1989, 1-100.
[14] KNIGHT D. W., SHAMSELDIN A. Y. River basin modeling for flood risk mitigation[M]. London, UK: Taylor and Francis, 2006, 301-334.
Notations
α– Width ratio
β– Relative depth or depth ratio
γ– Ratio of floodplain roughness to main channel roughness
δ– Aspect ratio of main channel
τ– Point boundary shear stress
τz– Average shear stress in any zone
A– Area of section
Amc– Area of main channel
%Afp– Percentage of flood plain area
%Amc– Percentage of main channel area
B– Half total width of compound section
b– Half width of main channel
Umc– Average streamwise velocity in main channel
Ufp– Average streamwise velocity in floodplain
fmc– Average Darcy’s frition factor in main channel
ffp– Average Darcy’s frition factor in floodplains
nmc– Average Manning’s roughness in main channel
nfp– Average Manning’s roughness in floodplains
R2– Coefficient of determination
Sfp– Shear force per unit length carried by floodplains
Smc– Shear force per unit length carried by main channel
Qmc– Component discharge in main channel
Qfp– Component discharge in floodplains
10.1016/S1001-6058(14)60017-2
* Biography: MOHANTY Prabir Kumar (1968-), Male, Ph. D.
KHATUA Kishanjit Kumar,
E-mail: kkkhatua@yahoo.com
 水動(dòng)力學(xué)研究與進(jìn)展 B輯2014年1期
水動(dòng)力學(xué)研究與進(jìn)展 B輯2014年1期
- 水動(dòng)力學(xué)研究與進(jìn)展 B輯的其它文章
- Deepwater gas kick simulation with consideration of the gas hydrate phase transition*
- Detrended analysis of Reynolds stress in a decaying turbulent flow in a wind tunnel with active grids*
- Influences of soil hydraulic and mechanical parameters on land subsidence and ground fissures caused by groundwater exploitation*
- A hybrid DEM/CFD approach for solid-liquid flows*
- An ocean circulation model based on Eulerian forward-backward difference scheme and three-dimensional, primitive equations and its application in regional simulations*
- Scale analysis of turbulent channel flow with varying pressure gradient*
