XU Ming-zhou,DING Yun-zheng,ZHOU Yong-zheng

(School of Information and Engineering,Jingdezhen Ceramic Institute,Jingdezhen 333403,China)

Abstract:In this article,we study central limit theorem for countable nonhomogeneous Markov chain under the condition of uniform convergence of transition probability matrices for countable nonhomogeneous Markov chain in Ces`aro sense.By Grtner-Ellis theorem and exponential equivalent method,we obtain a corresponding moderate deviation theorem for countable nonhomogeneous Markov chain.

Keywords:central limit theorem;moderate deviation;nonhomogeneous Markov chain;martingle

1 Introduction

Huang et al.[1]proved central limit theorem for nonhomogeneous Markov chain withfinite state space.Gao[2]obtained moderate deviation principles for homogeneous Markov chain.De Acosta[3]studied moderate deviations lower bounds for homogeneous Markov chain.De Acosta and Chen[4]established moderate deviations upper bounds for homogeneous Markov chain.It is natural and important to study central limit theorem and moderate deviation for countable nonhomogeneous Markov chain.We wish to investigate a central limit theorem and moderate deviation for countable nonhomogeneous Markov chain under the condition of uniform convergence of transition probability matrices for countable nonhomogeneous Markov chain in Ces`aro sense.

Suppose that{Xn,n≥0}is a nonhomogeneous Markov chain taking values inS={1,2,···}with initial probability

and the transition matrices

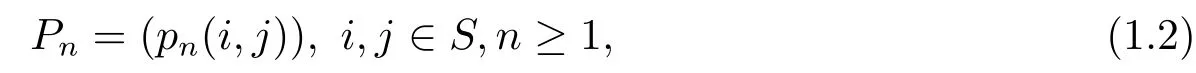
wherepn(i,j)=P(Xn=j|Xn?1=i).Write

When the Markov chain is homogeneous,P,Pkdenote,respectively.

IfPis a stochastic matrix,then we write

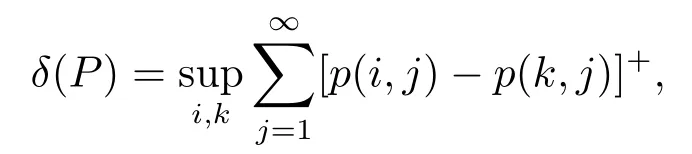
where[a]+=max{0,a}.

LetA=(aij)be a matrix defined asS×S.Write.

Ifh=(h1,h2,···),then we write.Ifg=(g1,g2,···)0,then we write|.The properties below hold(see Yang[5,6])

(a)kABk≤kAkkBkfor all matricesAandB;

(b)kPk=1 for all stochastic matrixP.

Suppose thatRis a‘constant’stochastic matrix each row of which is the same.Then{Pn,n≥1}is said to be strongly ergodic(with a constant stochastic matrixR)if for all.The sequence{Pn,n≥1}is said to converge in the Cesro sense(to a constant stochastic matrixR)if for everym≥0,

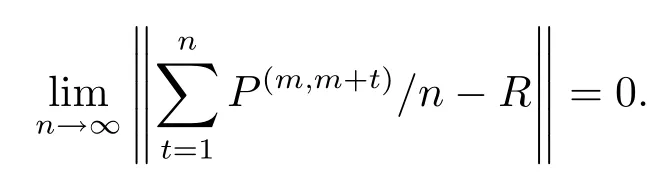
The sequence{Pn,n≥1}is said to uniformly converge in the Ces`aro sense(to a constant stochastic matrixR)if

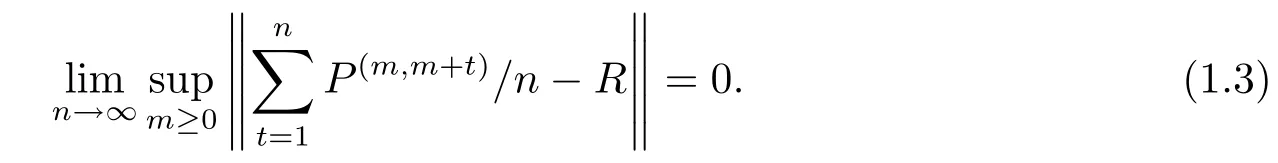
Sis divided intoddisjoint subspacesC0,C1,···,Cd?1,by an irreducible stochastic matrixP,of periodd(d≥1)(see Theorem 3.3 of Hu[7]),andPdgivesdstochastic matrices{Tl,0≤l≤d?1},whereTlis defined onCl.As in Bowerman et al.[8]and Yang[5],we shall discuss such an irreducible stochastic matrixP,of perioddthatTlis strongly ergodic forl=0,1,···,d?1.This matrix will be called periodic strongly ergodic.

Remark 1.1IfS={1,2,···},d=2,P=(p(i,j)),p(1,2)=1,p(k,k?1)=,thenPis an irreducible stochastic matrix of period 2.Moreover,

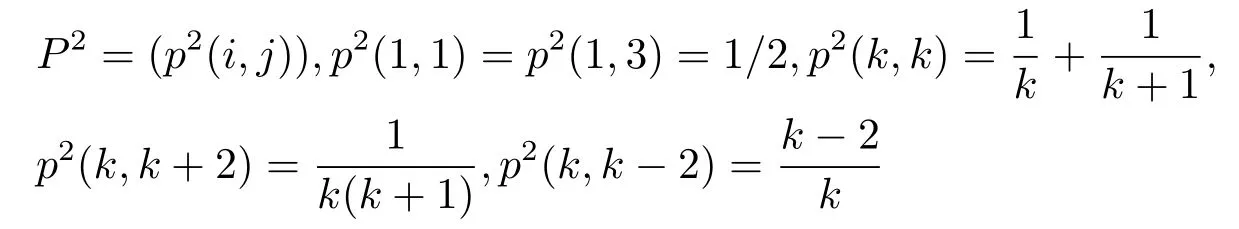
fork≥2.

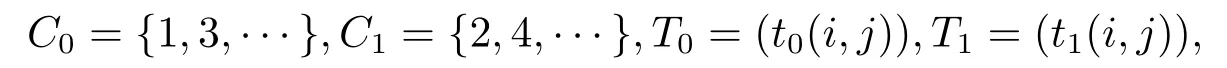
where

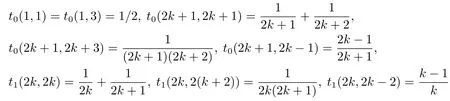
fork≥1.The solution ofπP=πandare

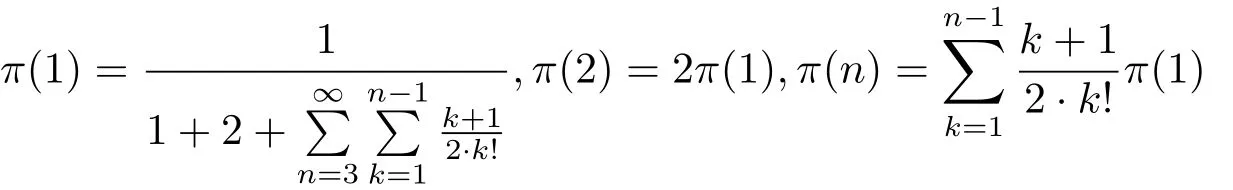
forn≥3.

Theorem 1.1Suppose{Xn,n≥0}is a countable nonhomogeneous Markov chain taking values inS={1,2,···}with initial distribution of(1.1)and transition matrices of(1.2).Assume thatfis a real function satisfying|f(x)|≤Mfor allx∈R.Suppose thatPis a periodic strongly ergodic stochastic matrix.Assume thatRis a constant stochastic matrix each row of which is the left eigenvectorπ=(π(1),π(2),···)ofPsatisfyingπP=πand.Assume that

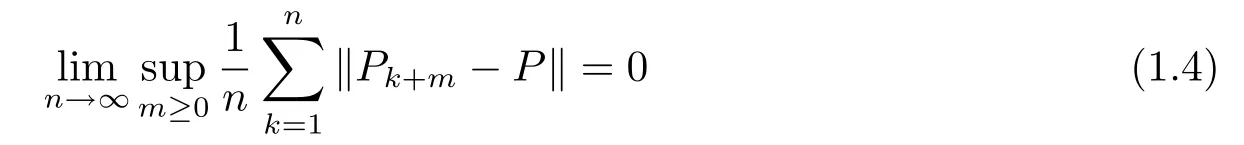
and

Moreover,if the sequence ofδ-coefficient satisfies

then we have

Theorem 1.2Under the hypotheses of Theorem 1.1,if moreover

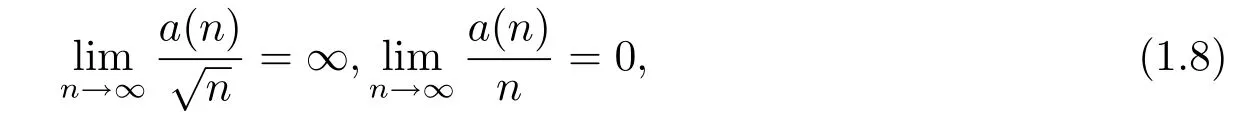
then for each open setG?R1,

and for each closed setF?R1,

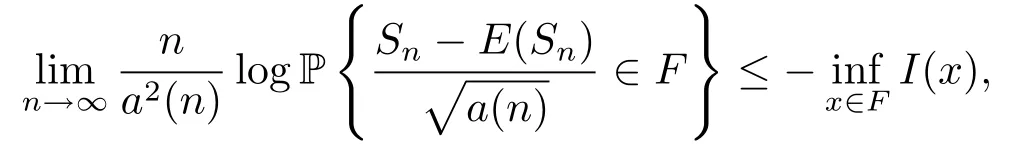
In Sections 2 and 3,we prove Theorems 1.1 and 1.2.The ideas of proofs of Theorem 1.1 come from Huang et al.[1]and Yang[5].

2 Proof of Theorem 1.1

Let

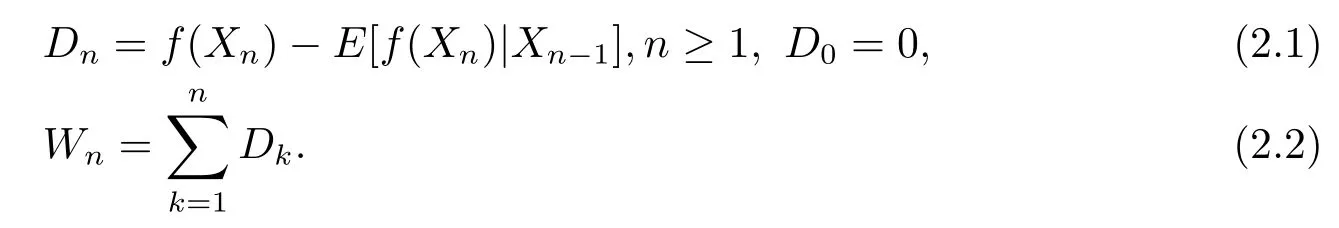
WriteFn=σ(Xk,0≤k≤n).Then{Wn,Fn,n≥1}is a martingale,so that{Dn,Fn,n≥0}is the related martingale difference.Forn=1,2,···,set

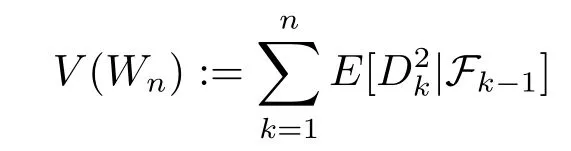
and

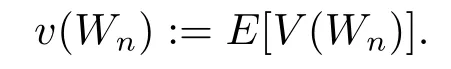
It is clear that

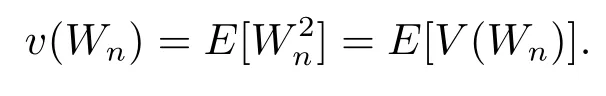
As in Huang et al.[1],to prove Theorem 1.1,we first state the central limit theorem associated with the stochastic sequence of{Wn}n≥1,which is a key step to establish Theorem 1.1.

Lemma 2.1Assume{Xn,n≥0}is a countable nonhomogeneous Markov chain taking values inS={1,2,···}with initial distribution of(1.1)and transition matrices of(1.2).Supposefis a real function satisfying|f(x)|≤Mfor allx∈R.Assume thatPis a periodic strongly ergodic stochastic matrix,andRis a constant stochastic matrix each row of which is the left eigenvectorπ=(π(1),π(2),···)ofPsatisfyingπP=πand.Suppose that(1.4)and(1.5)are satisfied,and{Wn,n≥0}is defined by(2.2).Then

As in Huang et al.[1],to establish Lemma 2.1,we need two important statements below such as Lemma 2.2(see Brown[9])and Lemma 2.3(see Yang[6]).

Lemma 2.2Assume that(?,F,P)is a probability space,and{Fn,n=1,2,···}is an increasing sequence ofσ-algebras.Suppose that{Mn,Fn,n=1,2,···}is a martingale,denote its related martingale difference byξ0=0,ξn=Mn?Mn?1(n=1,2,···).Forn=1,2,···,write

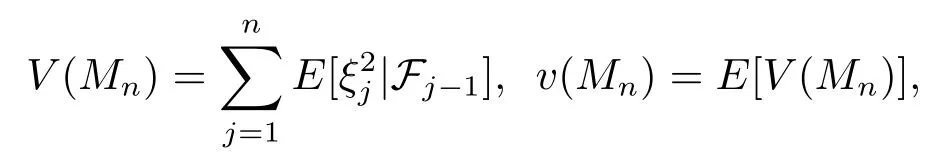
whereF0is the trivialσ-algebra.Assume that the following holds

(i)

(ii)the Lindeberg condition holds,i.e.,for any?>0,

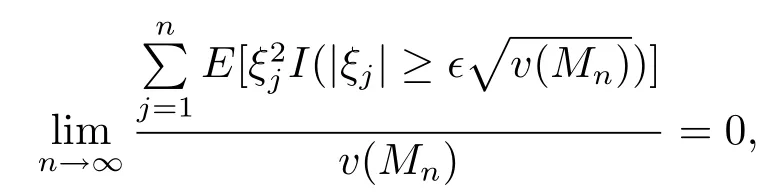
whereI(·)denotes the indicator function.Then we have

Writeδi(j)=δij,(i,j∈S).Set

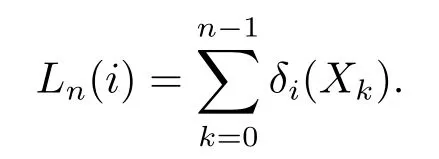
Lemma 2.3Assume that{Xn,n≥0}is a countable nonhomogeneous Markov chain taking values inS={1,2,···}with initial distribution(1.1),and transition matrices(1.2).Suppose thatPis a periodic strongly ergodic stochastic matrix,andRis matrix each row of which is the left eigenvectorπ=(π(1),π(2),···)ofPsatisfyingπP=πand.Assume(1.4)holds.Then

Now let’s come to establish Lemma 2.1.

Proof of Lemma 2.1Applications of properties of the conditional expectation and Markov chains yield

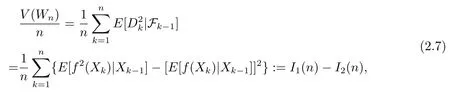
where

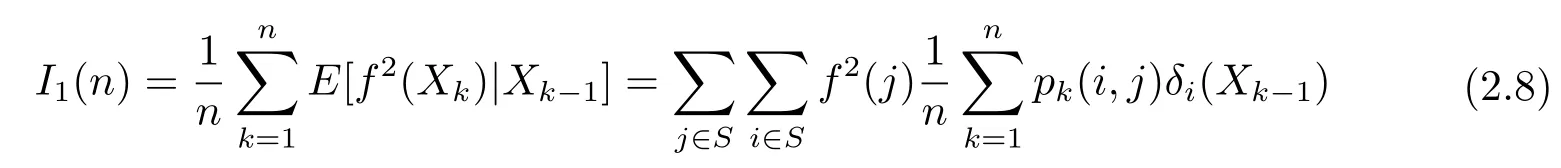
and

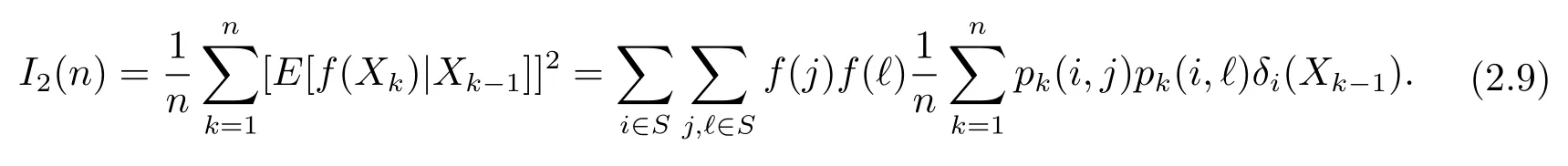
We first use(1.4)and Fubini’s theorem to obtain

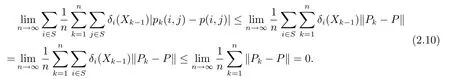
Hence,it follows from(2.10)andπP=πthat

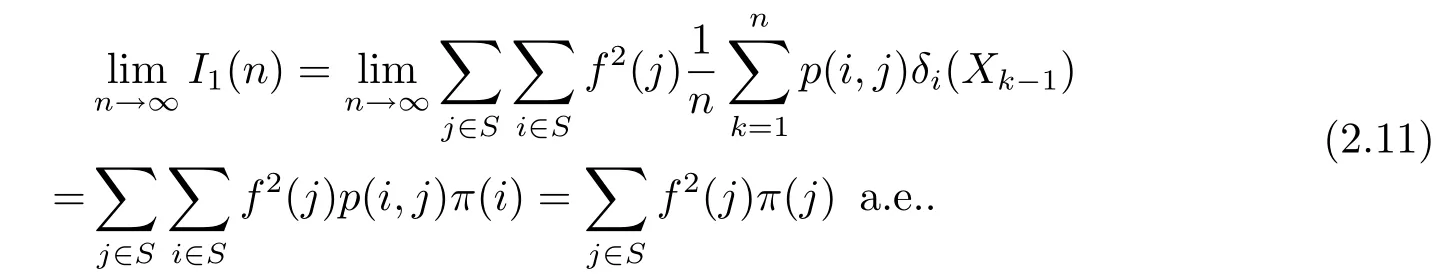
We next claim that

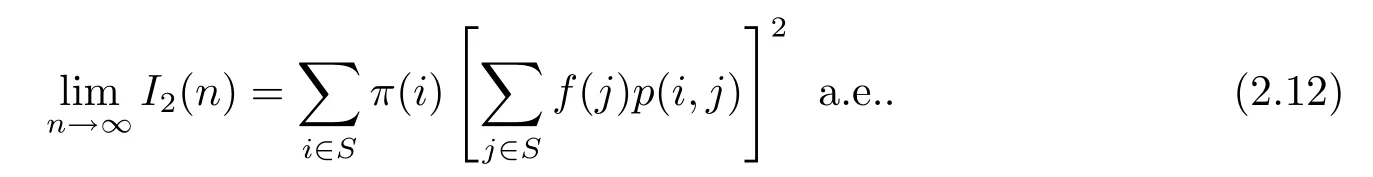
Indeed,we use(1.4)and(2.9)to have

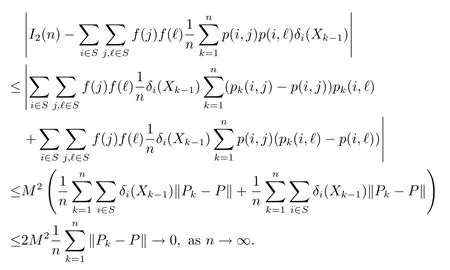
Thus we use Lemma 2.3 again to obtain

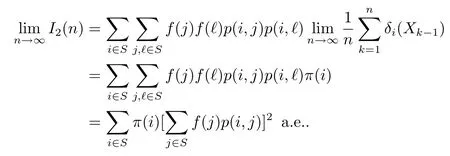
Therefore(2.12)holds.Combining(2.11)and(2.12)results in

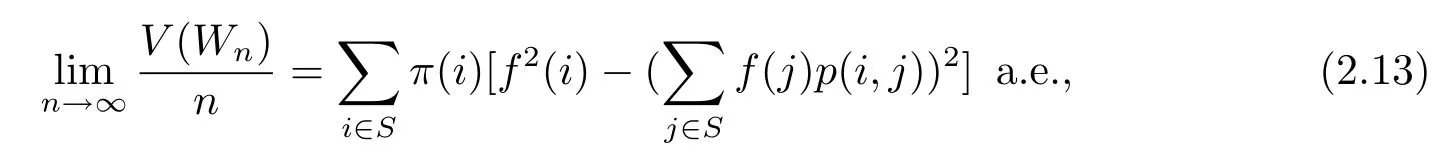
which gives

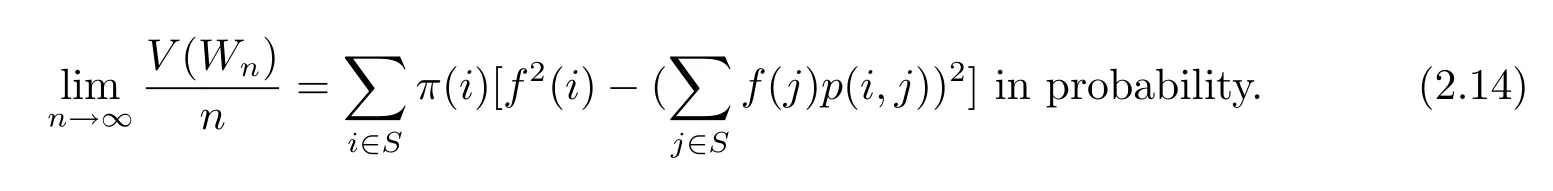
Since{V(Wn)/n,n≥1}is uniformly bounded,{V(Wn)/n,n≥1}is uniformly integrable.By applying the above two facts,and(1.5),we have

Therefore we obtain

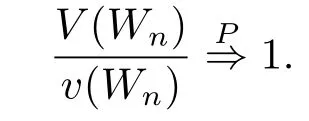
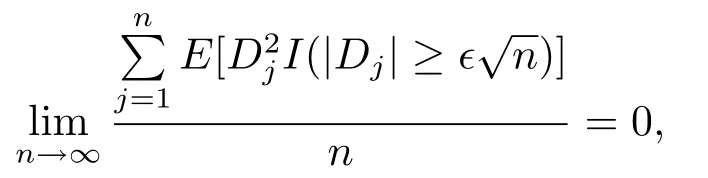
which implies that the Lindeberg condition holds.Application of Lemma 2.2 yields(2.3).This establishes Lemma 2.1.

Proof of Theorem 1.1Note that

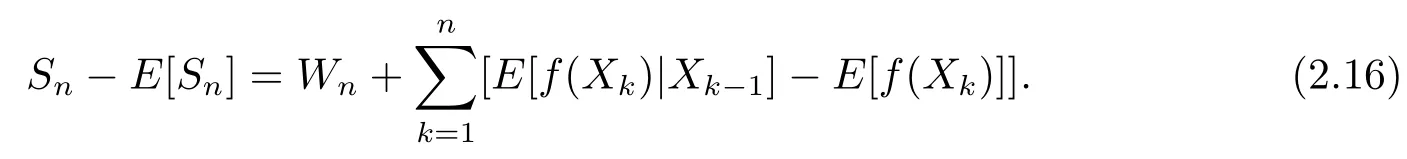
Write

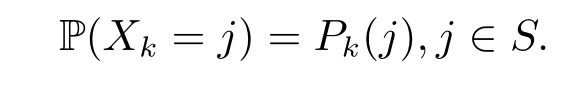
Let’s evaluate the upper bound of|E[f(Xk)|Xk?1]?E[f(Xk)]|.In fact,we use the C-K formula of Markov chain to obtain

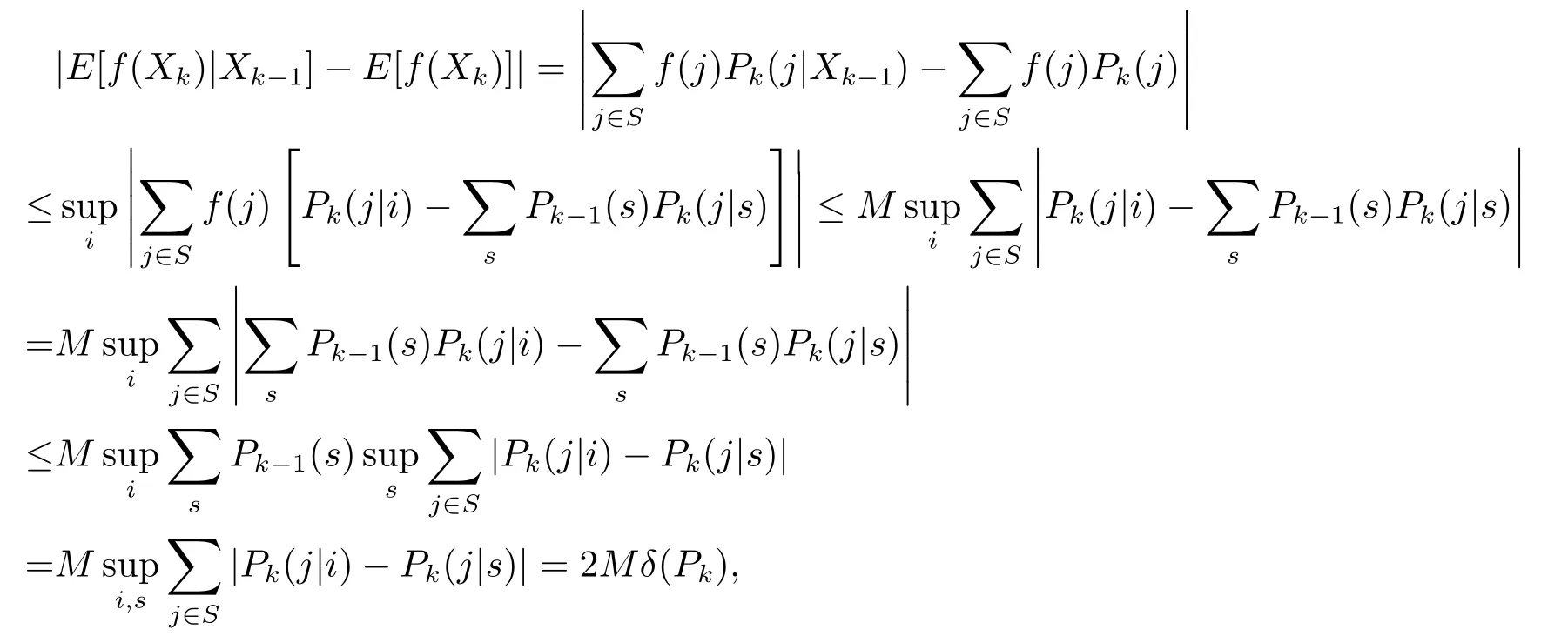
here

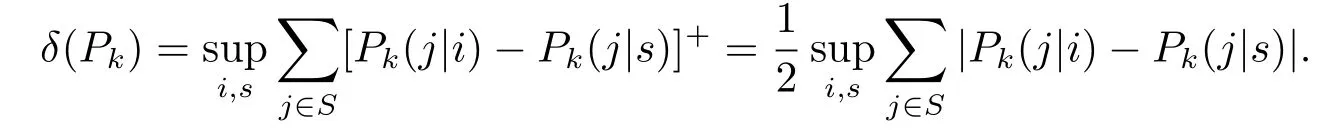
Application of(1.6)yields

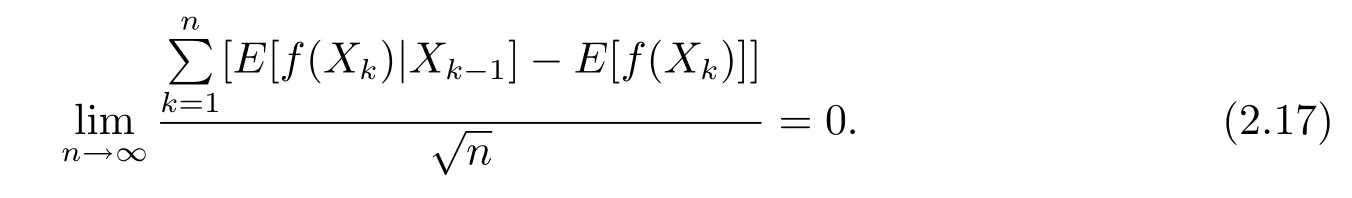
Combining(1.6),(2.3),(2.16),and(2.17),results in(1.7).This proves Theorem 1.1.

3 Proof of Theorem 1.2

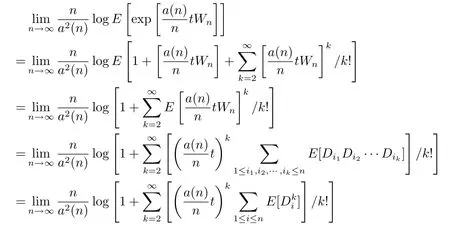
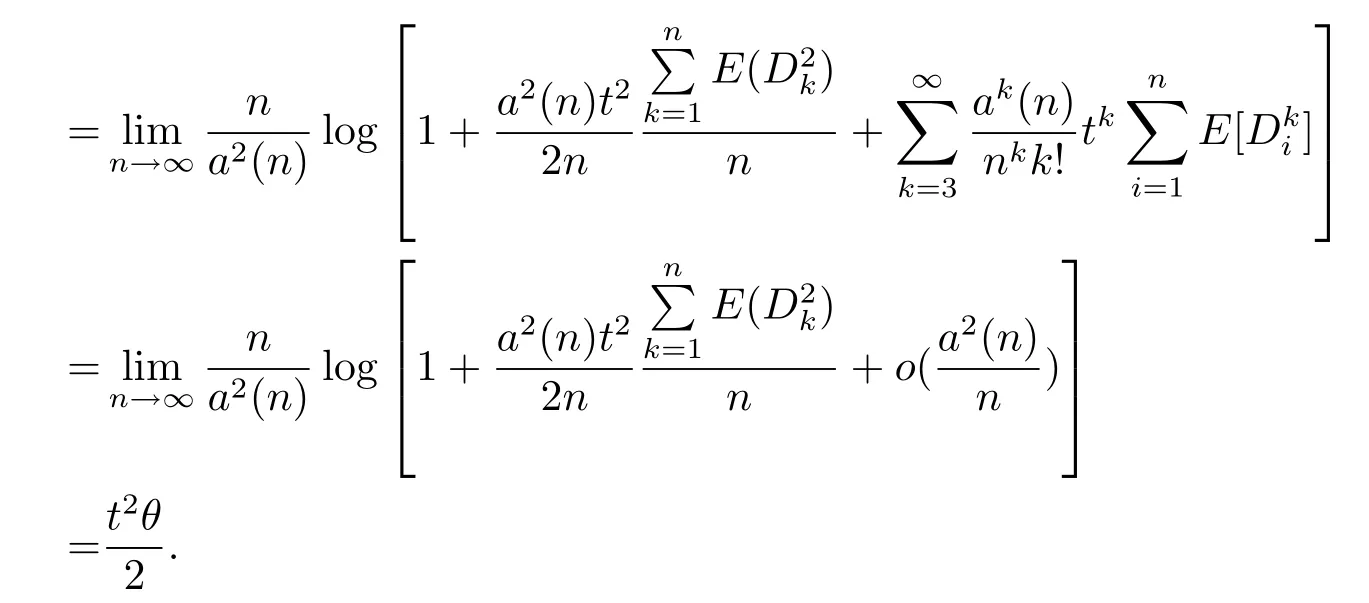
In fact,by(1.8),

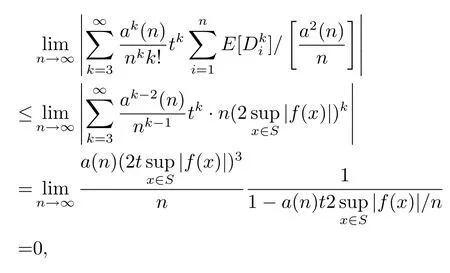
and the claim is proved.Hence,by using Grtner-Ellis theorem,we deduce thatWn/a(n)satisfies the moderate deviation theorem with rate function.It follows from(1.8)and(2.17)that??>0,

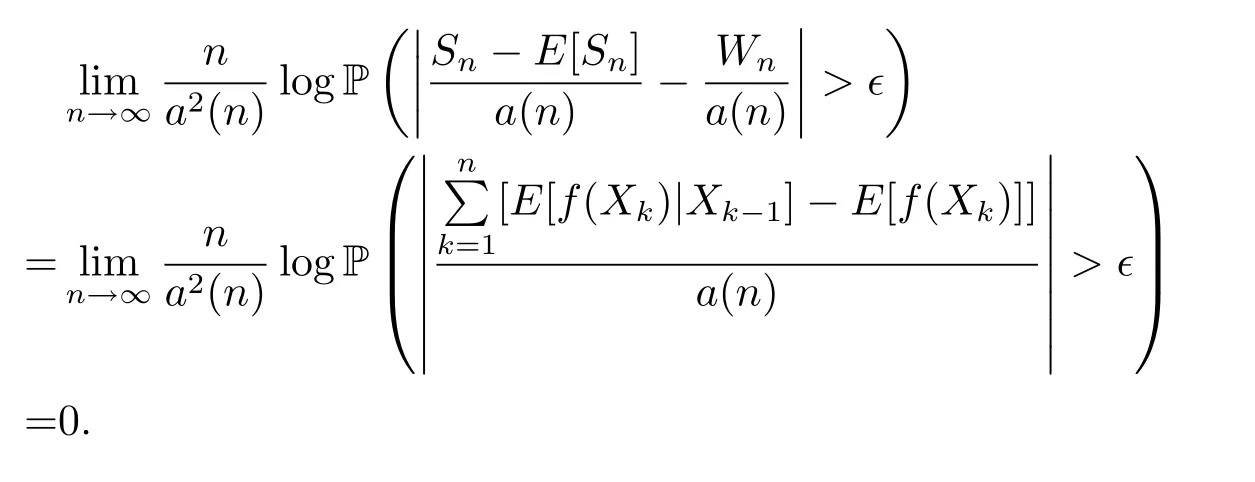
Thus,by the exponential equivalent method(see Theorem 4.2.13 of Dembo and Zeitouni[10],Gao[11]),we see thatsatisfies the same moderate deviation theorem aswith rate function.This completes the proof.